Determine the centre of mass of a uniform hemisphere of radius R.
To determine the center of mass of a uniform hemisphere of radius R, we can follow these steps:
Step 1: Define the Geometry
We have a uniform hemisphere with radius R. We can set up a coordinate system where the flat circular face of the hemisphere lies on the xy-plane, and the curved surface extends upwards along the z-axis. The center of the flat face is at the origin (0, 0, 0).
Step 2: Consider a Differential Element
To find the center of mass, we will consider a differential mass element. We can take a thin semicircular ring at a distance r from the center of the flat face, with a small thickness dr.
Step 3: Calculate the Mass of the Differential Element
The area of a full circle is πr2, so the area of the semicircular ring is 12πr2. The mass per unit area σ of the hemisphere is given by:
σ=MArea of hemisphere=M12πR2
The mass of the differential ring dm can be expressed as:
dm=σ⋅Area of the ring=σ⋅(πr⋅dr)
Substituting for σ:
dm=M12πR2⋅(πr⋅dr)=2MR2⋅r⋅dr
Step 4: Determine the Coordinates of the Center of Mass
The x-coordinate of the center of mass xcm is zero due to symmetry. Now, we need to find the y-coordinate of the center of mass ycm:
ycm=1M∫ydm
For the semicircular ring, the y-coordinate can be expressed as y=√R2−r2 (the height of the ring above the xy-plane). Thus:
ycm=1M∫R0√R2−r2⋅dm
Substituting for dm:
ycm=1M∫R0√R2−r2⋅2MR2rdr
Step 5: Solve the Integral
Now we simplify and solve the integral:
ycm=2R2∫R0r√R2−r2dr
Using the substitution r=Rsinθ, we can change the limits and solve the integral:
∫r√R2−r2dr=R23(πR22)=R3π6
Thus, substituting back, we find:
ycm=2R2⋅R3π6=πR3
Step 6: Final Result
The center of mass of the hemisphere is then:
Center of mass=(0,0,ycm)=(0,0,4R3π)
Summary
The center of mass of a uniform hemisphere of radius R is at the coordinates:
(0,0,4R3π)
We have a uniform hemisphere with radius R. We can set up a coordinate system where the flat circular face of the hemisphere lies on the xy-plane, and the curved surface extends upwards along the z-axis. The center of the flat face is at the origin (0, 0, 0).
To find the center of mass, we will consider a differential mass element. We can take a thin semicircular ring at a distance r from the center of the flat face, with a small thickness dr.
The area of a full circle is πr2, so the area of the semicircular ring is 12πr2. The mass per unit area σ of the hemisphere is given by:
The x-coordinate of the center of mass xcm is zero due to symmetry. Now, we need to find the y-coordinate of the center of mass ycm:
Now we simplify and solve the integral:
The center of mass of the hemisphere is then:
The center of mass of a uniform hemisphere of radius R is at the coordinates:
Similar Questions
A particle of mass m was transferred from the centre of the base of a uniform hemisphere of mass M and radius R into infinity.
What work was performed in the process by the gravitational force exerted on the particle by the hemisphere?
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionWhat can be said about the centre of mass of a uniform hemisphere without making any calculation? Will its distance from the centre be more than r/2 or less than r/2?
View SolutionWhat can be said about the centre of mass of a solid hemisphere of radius r without making any calculation. Will its distance from the centre be more than r/2 or less than r/2?
View SolutionFind the centre of mass of a uniform disc of radius a from which a circualr section of radius b has been removed. The centre of hole is at a distance c from the centre of the disc.
View SolutionFind distance of centre of mass of solid hemisphere of radius 8cm from centre
View SolutionFind distance of centre of mass of solid hemisphere of radius 8cm from centre
View SolutionCalculate the gravitational field intensity and potential at the centre of the base of a solid hemisphere of mass m, radius R
View SolutionThe magnitude of the gravitational field at distance r1 and r2 from the centre of a uniform sphere of radius R and mass M are F1 and F2 respectively. Then:
View SolutionCalculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
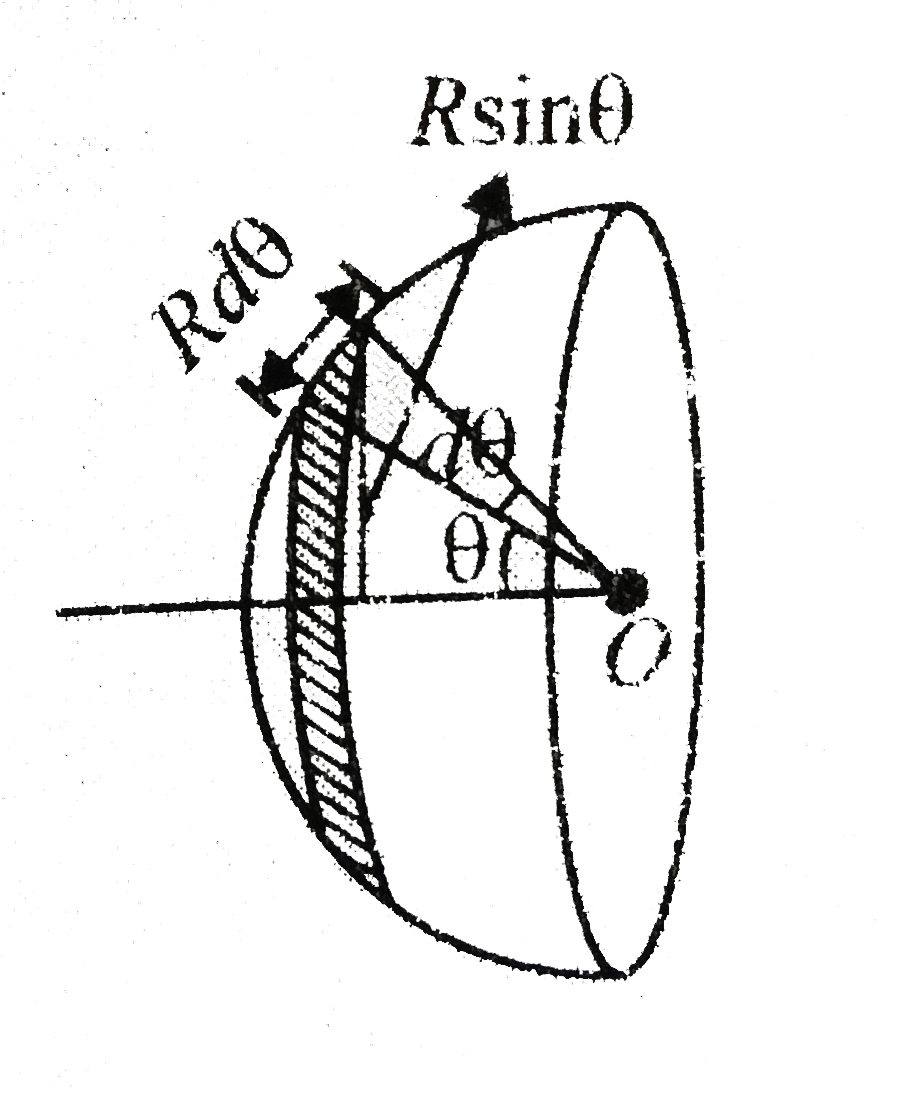
View Solution
