The diagram shows a tall cylindrical container kept on a horizontal surface. From a tap, water come with constant rate qm3/s. Initially the container was empty. An orifice of area of cross section A is made at some height h in the side wall of the container. If the horizontal range of the water jet in steady is found to be 2h, then the relation between h and q is
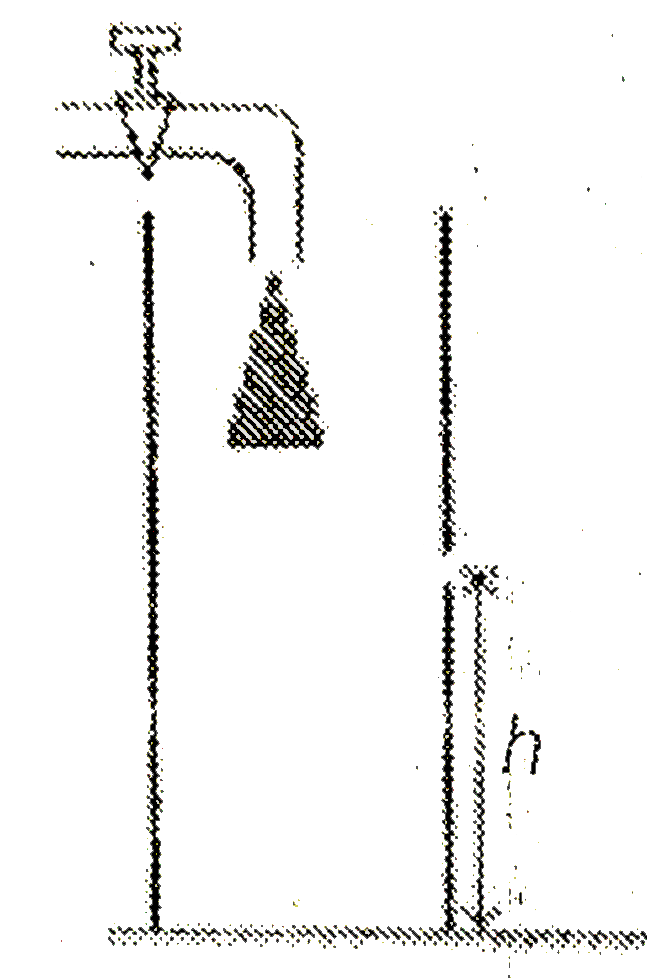
h=q2gA2
h=q22gA2
h=3q22gA2
h=2q2gA2
The correct Answer is:B
Step by step video, text & image solution for The diagram shows a tall cylindrical container kept on a horizontal surface. From a tap, water come with constant rate q m^(3)//s. Initially the container was empty. An orifice of area of cross section A is made at some height h in the side wall of the container. If the horizontal range of the water jet in steady is found to be 2h, then the relation between h and q is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES OF MATTERBook:DC PANDEYChapter:PROPERTIES OF MATTERExercise:More than one option is correct
Explore 21 Videos
- PROPERTIES OF MATTERBook:DC PANDEYChapter:PROPERTIES OF MATTERExercise:Comprehension
Explore 32 Videos
- PROPERTIES OF MATTERBook:DC PANDEYChapter:PROPERTIES OF MATTERExercise:Integer
Explore 8 Videos
- PROJECTILE MOTIONBook:DC PANDEYChapter:PROJECTILE MOTIONExercise:Level - 2 Subjective
Explore 10 Videos
- RAY OPTICSBook:DC PANDEYChapter:RAY OPTICSExercise:Integer type q.
Explore 15 Videos
Similar Questions
Water is filled to a height in a long container. From what height below the free surface a hole should be bored in the wall of a container so that the horizontal range is maximum?
View Solution_E01_216_Q01.png)
A syringe containing water is held horizontally with its nozzle at a height h above the ground as shown in fig the cross sectional areas of the piston and the nozzle are A and a respectively the piston is pushed with a constant speed v. Find the horizontal range R of the stream of water on the ground.
View Solution
Knowledge Check
A tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
A2√h(H−h)B4√h(H+h)C4√h(H−h)D2√h(H+h)A cylindrical vessel of cross section A contains water to a height h. There is a hole in the bottom of radius 'a'. The time in which it will be emptied is:
A2Aπa2√hgB√2Aπa2√hgC2√2Aπa2√hgDA√2πa2√hgIn the figure shown, a light container is kept on a horizontal rough surface of coefficient of friction μ=ShV. A very small hole of area S is made at depth 'h'. Water of volume 'V' is filled in the container. The friction is not sufficient to keep the container at rest. The acceleration of the container initially is
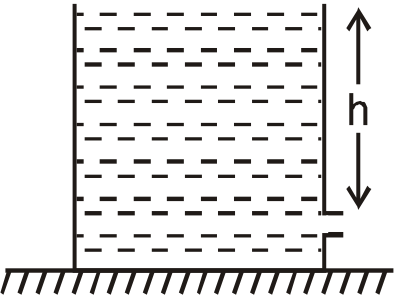 AVShgBgCzeroDShVg
AVShgBgCzeroDShVg
A cylindrical vessel of area of cross section A is filled with a liquid up to a height H. A very small hole of area of cross section a is made at the bottom of the vellel. Find the time taken by the vessel to become empty.
View SolutionAt the mount of the tap area of cross-section is 2.0cm2 and the speed of water is 3m/s. The area of cross-section of the water column 80 cm below the tap is (use g=10m/s2)
View SolutionIn a capillary tube having area of cross - section A, water rises to a height h. If cross-sectional area is reduced to A9, the rise of water in the capillary tube is
View SolutionOil is filled in a cylindrical container up to height 4m. A small hole of area 'p' is punched in the wall of the container at a height 1.52m from the bottom. The cross sectional area of the container is Q. Ifpq=0.1 then v is (where vis the velocity of oil coming out of the hole)
View SolutionIn the previous question, at the mouth of the tap the area of cross-section is 2.5cm2 and the speed of water in 3m/s. The area of cross-section of the water column 80cm below the tap is.
View Solution