The displacement x of a particle varies with time as x=4t2–15t+25. Find the position, velocity and acceleration of the particle at t = 0.
To solve the problem, we need to find the position, velocity, and acceleration of a particle whose displacement x varies with time t according to the equation:
x=4t2−15t+25
We will evaluate these quantities at t=0.
Step 1: Find the Position at t=0
To find the position of the particle at t=0, we substitute t=0 into the displacement equation:
x(0)=4(0)2−15(0)+25
Calculating this gives:
x(0)=0−0+25=25
Thus, the position of the particle at t=0 is:
Position=25 meters
Step 2: Find the Velocity at t=0
Velocity is the first derivative of displacement with respect to time. We differentiate the displacement equation:
v(t)=dxdt=ddt(4t2−15t+25)
Using the power rule of differentiation:
v(t)=8t−15
Now, we substitute t=0 into the velocity equation:
v(0)=8(0)−15=−15
Thus, the velocity of the particle at t=0 is:
Velocity=−15 meters per second
Step 3: Find the Acceleration at t=0
Acceleration is the derivative of velocity with respect to time. We differentiate the velocity equation:
a(t)=dvdt=ddt(8t−15)
Differentiating gives:
a(t)=8
Since acceleration is constant, it does not depend on time. Thus, at t=0:
Acceleration=8 meters per second squared
Summary of Results
At t=0:
- Position: 25 meters
- Velocity: −15 meters per second
- Acceleration: 8 meters per second squared
- Position: 25 meters
- Velocity: −15 meters per second
- Acceleration: 8 meters per second squared
Topper's Solved these Questions
- KINEMATICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINEMATICSEXERCISE - M.C.Q.
20 Videos
- KINEMATICSBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINEMATICSEXERCISE - 5 Marks
2 Videos
- GRAVITATIONBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - GRAVITATIONEXERCISE - MULTIPLE CHOICE QUESTIONS
19 Videos
- KINETIC THEORY OF GASESBOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - KINETIC THEORY OF GASESEXERCISE - Objective question
20 Videos
Similar Questions
The displacement x of a particle varies with time t as
x=4t2−15t+25
Find the position, velocity and acceleration of the particle at t=0. When will the velocity of the particle become zero? Can we call the motion of the particle as one with uniform acceleration ?
View SolutionThe displacement s of a particle at a time t is given bys=t3−4t2−5t. Find its velocity and acceleration at t = 2.
View Solution
Knowledge Check
If the displacement of a particle varies with time as √x=t+3
Avelocity of the particle is inversely proportional to tBvelocity of particle varies linearly with tCvelocity of particle is proportional to √tDinitial velocity of the particle is zeroIf displacements of a particle varies with time t as s=1t2 , then.
AThe particle is moving with constant velocityBThe particle is moving with variable acceleration of decreasing orderCThe particle is moving with constant retardationDThe particle has constant speed but variable velocityIf the displacement of a particle varies with time as √x=t+7 . The -
Avelocity of the particle is inversely proportional to tBvelocity of the particle is proportional to tCvelocity of the particle is proportional to √tDthe particle moves with a constant acceleration
The displacement x of a particle varies as
√x=t−2
(i) What is the velocity and acceleration of the particle at t=0?
(ii) When will the velocity of the particle become zero?
(iii) What is the displacement of the particle when its velocity is zero?
(iv) Is the motion of the particle uniformly accelerated or not?
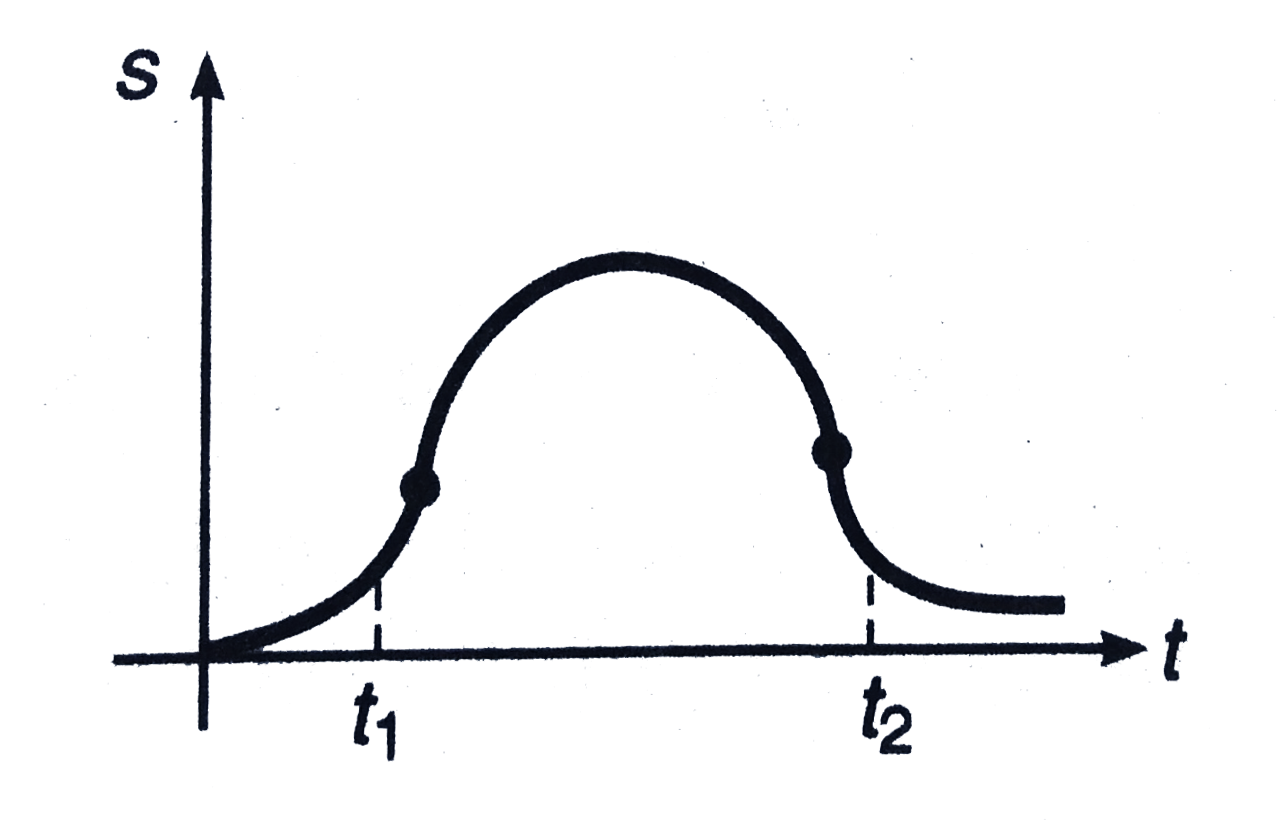
View SolutionFigure shows the displacement-time graph of a particle moving in a straight line. Find the signs of velocity and acceleration of particle at time t=t1andt=t2.
View SolutionThe position x of a particle varies with time t as x=at^(2)-bt^(3) .The acceleration of the particle will be zero at time t equal to
View Solutionthe angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View SolutionIf the displacement x of a particle (in metre) is related with time (in second) according to relation
x=2t3−3t2+2t+2
find the position, velocity and acceleration of a particle at the end of 2 seconds.
View Solution
