The equation of a travelling wave is given as y=5sin10π(t−Q.01x), along the x-axis . Here, all quantities are in SI units. The phase difference between the points separated by a distance of 10m alond x-axis is
x2
π
2π
π4
The correct Answer is:B
To find the phase difference between two points separated by a distance of 10 meters along the x-axis for the wave described by the equation y=5sin(10π(t−0.01x)), we can follow these steps:
Step 1: Identify the wave equation
The given wave equation is:
y=5sin(10π(t−0.01x))
This can be rewritten as:
y=5sin(10πt−0.1πx)
Step 2: Identify the wave parameters
From the wave equation, we can identify:
- Angular frequency ω=10π rad/s
- Wave number k=0.1π rad/m
Step 3: Calculate the wavelength
The wave number k is related to the wavelength λ by the formula:
k=2πλ
Substituting the value of k:
0.1π=2πλ
Solving for λ:
λ=2π0.1π=20 m
Step 4: Calculate the phase difference
The phase difference Δϕ between two points separated by a distance d is given by:
Δϕ=k⋅d
Substituting k and d=10 m:
Δϕ=(0.1π)⋅10=π
Step 5: Conclusion
The phase difference between the two points separated by a distance of 10 meters along the x-axis is:
Δϕ=π radians
The given wave equation is:
y=5sin(10π(t−0.01x))
This can be rewritten as:
y=5sin(10πt−0.1πx)
From the wave equation, we can identify:
- Angular frequency ω=10π rad/s
- Wave number k=0.1π rad/m
The wave number k is related to the wavelength λ by the formula:
k=2πλ
Substituting the value of k:
0.1π=2πλ
Solving for λ:
λ=2π0.1π=20 m
The phase difference Δϕ between two points separated by a distance d is given by:
Δϕ=k⋅d
Substituting k and d=10 m:
Δϕ=(0.1π)⋅10=π
The phase difference between the two points separated by a distance of 10 meters along the x-axis is:
Δϕ=π radians
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - ONLY ONE OPTION IS CORRECT62videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct23videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Subjective Questions2videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery9videos
VECTORS
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
Similar Questions
The equation of a wave is given by Y=5sin10π(t−0.01x) along the x-axis. (All the quantities are expressed in SI units). The phase difference between the points separated by a distance of 10m along x-axis is
View SolutionThe equation of a simple harmonic wave is given by y=6sin2π(2t–0.1x) ,where x and y are in mm and t is in second. The phase difference between two particles 2 mm apart at any instant is
View Solution
Knowledge Check
- Question 1 - Select One
For the travelling harmonic wave
y(x, t) = 2 cos2π(10t - 0.008x + 0.35) where X and Y are in cm and t is in s. The phase difference between oscillatory motion of two points separated by distance of 0.5 m isA0.2πradB0.4πradC0.6πradD0.8πrad
A plane progressive wave is given by y=3×10−7sin(8500t−25x), where t is in second y is in m. Find the amplitude, and phase difference between two points separated by a distance 0.01m.
View SolutionFor the harmonic travelling wave y=2cos2pi(10t-0.0080x+3.5) where x and y ar in cm and t is in second. What is the phase diffference between the oscillatory motion at two points separated by a distance of
View SolutionThe equation of a transverse wave is given by
y=10sinπ(0.01x−2t)
where x and y are in cm and t is in second. Its frequency isView SolutionA travelling wave in a string is represented by y=3sin(π2t−π4x). The phase difference between two particles separated by a distance 4 cm is ( Take x and y in cm and t in seconds )
View SolutionA wave travelling along the length of a string is shown in Fig. 14.4.12. Using the scale on the given axis, find
(i) The amplitude of the wave
(ii) The wavelength of the wave
(iii) If the frequency of the wave is 10 Hz, What is the speed of the wave?
(iv) What is the initial phase of the wave ?
(v) What is the phase difference between two points separated by a distance 10 cm ?
(vi) If this wave is made to travel through water what is the wavelength of the wave, if the speed of, the waves in water is 10 m/s.
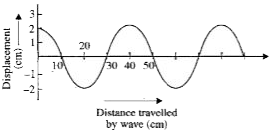 View Solution
View SolutionThe displacement equation of a wave is y = 0.5 sin π(2+−0.01x) where x is in meter and t is in second. Find the frequency, amplitude, speeds and phase difference between two particles situated at a distance of 5 m from each other.
View SolutionThe equation of a travelling wave is,
Y=Asin2π(pt−x/5)
Then the ratio of maximum particle velocity to wave velocity is,View Solution

