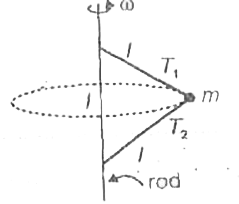
The figure shows a rod arranged at an angle of 30∘ from the horizontal. Two strings are fixed to rod as shown. Attached to the two strings is the mass m as shown. The rod is rotated maintaining its direction in space, so that m travels in a circular path. The strings are of equal length and make angle of 60∘ with the rod as shown. Calculate the minimum value of the tangential speed (ms) of the mass such that the string with tension T2 does not become slack when the mass is directly aboye the rod. Take length of string as. l=2.4m
'(##CEN_KSR_PHY_JEE_C07_036_Q22##)'
The correct Answer is:6
Topper's Solved these Questions
Similar Questions
Knowledge Check
A uniform rod of length 3L and mass m is suspended from a horizontal roof by two strings of length L and 2L as shown. Then the tension in the left string of length L is :
AmgBmg4Cmg2Dmg3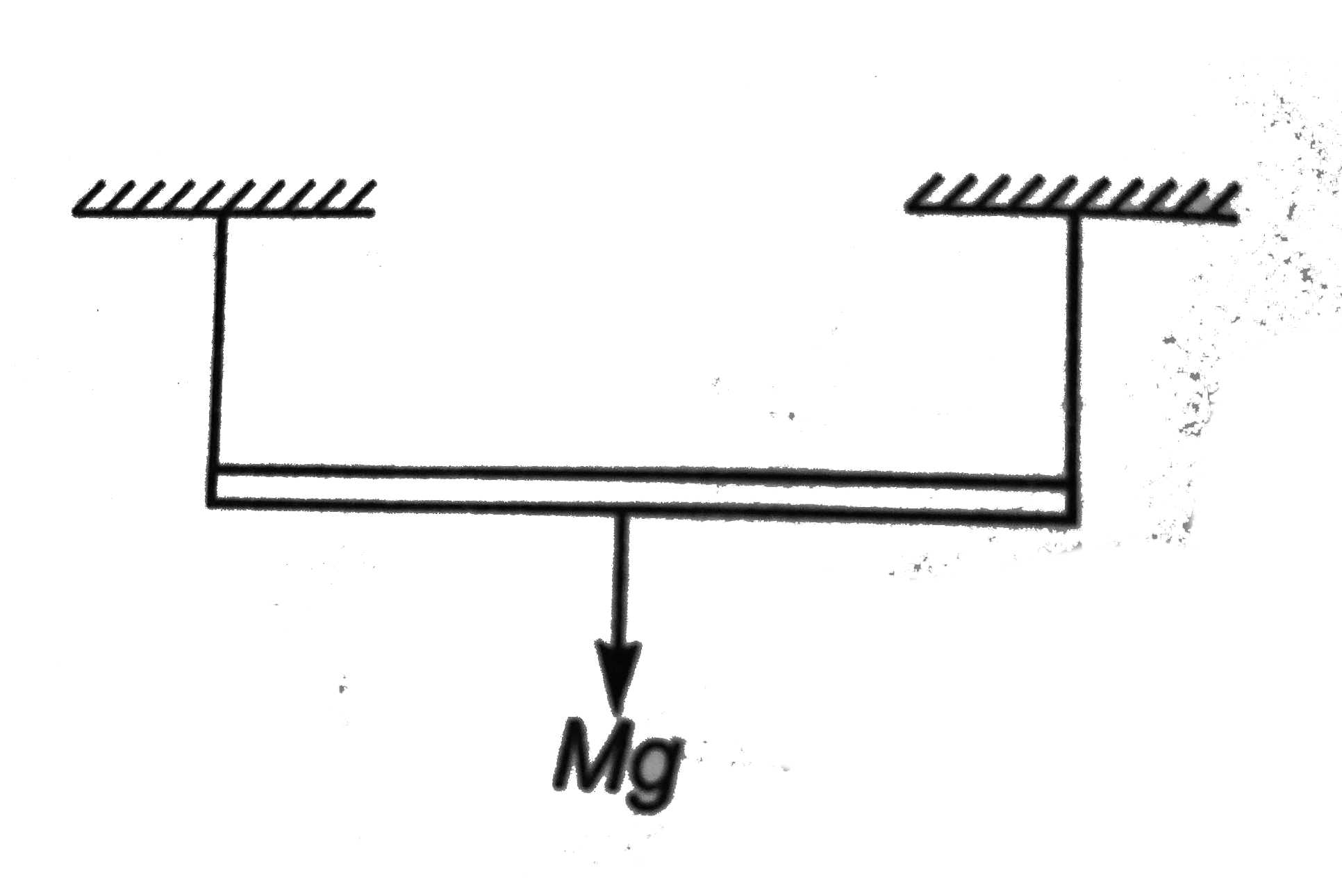
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.Aremains unaffectedBincreaseCdecreaseDbecome equal to weight of the rod.A uniform rod of mass and length l is suspended by two strings at its ends shown. When one of the strings is cut, the rod starts falling with an initial angular acceleration.
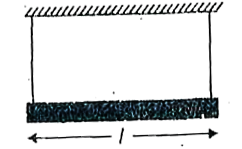AglBg2lC3g2lD3g4l
-
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.
View Solution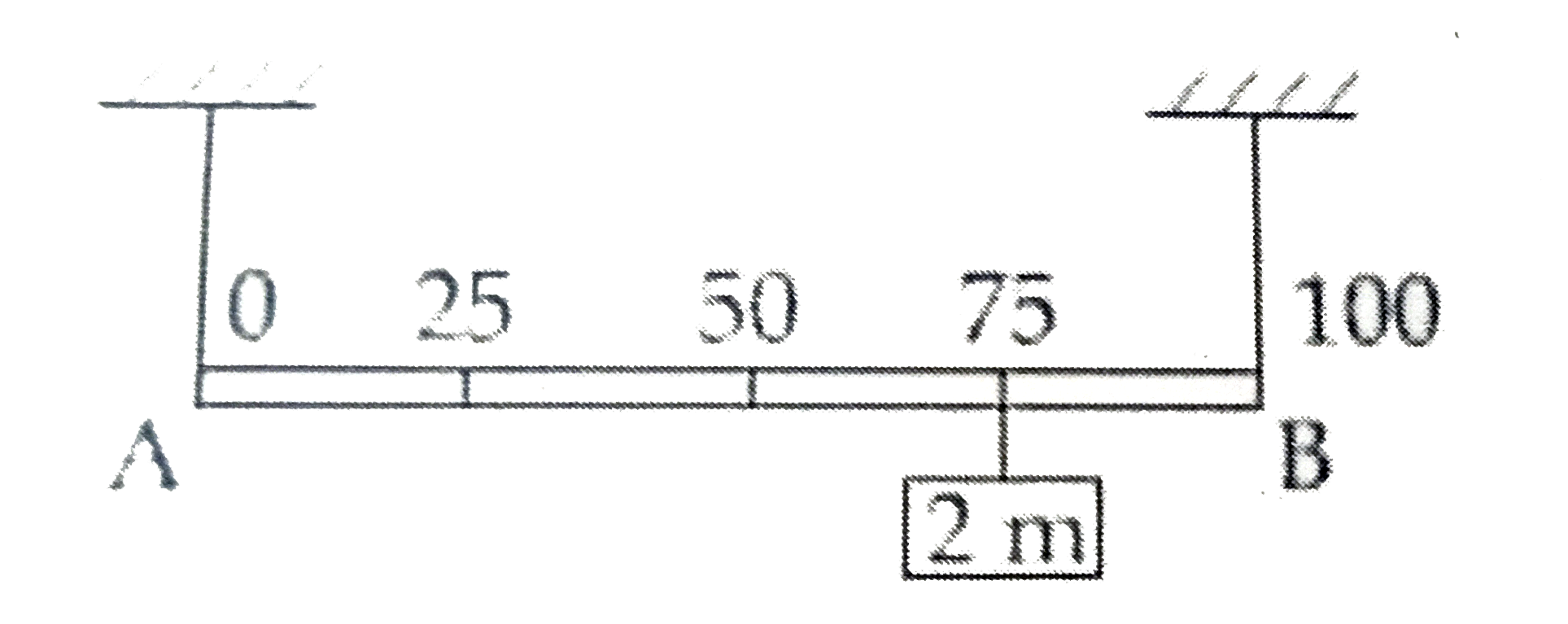 
Shown in the figure is rigid and uniform one meter long rod AB held in horizontal position by two strings tied to its ends and attached to the ceiling . The rod is of mass 'm' and has another weight of mass 2 m hung at a distance of 75 cm from A . The tension in the string at A is :
View Solution-
Shown in the figure is rigid and uniform one meter long rod AB held in horizontal position by two strings tied to its ends and attached to the ceiling . The rod is of mass 'm' and has another weight of mass 2 m hung at a distance of 75 cm from A . The tension in the string at A is :
View Solution A uniform rod of mass and length l is suspended by two strings at its ends shown. When one of the strings is cut, the rod starts falling with an initial angular acceleration.
View SolutionA rod of length 1m is sliding in a corner as shown. At an instant when the rod makes an angle of 60∘ with the horizontal plane, the velocity of point A on the rod is 1 m/s. The angular velocity of the rod at this instant is :
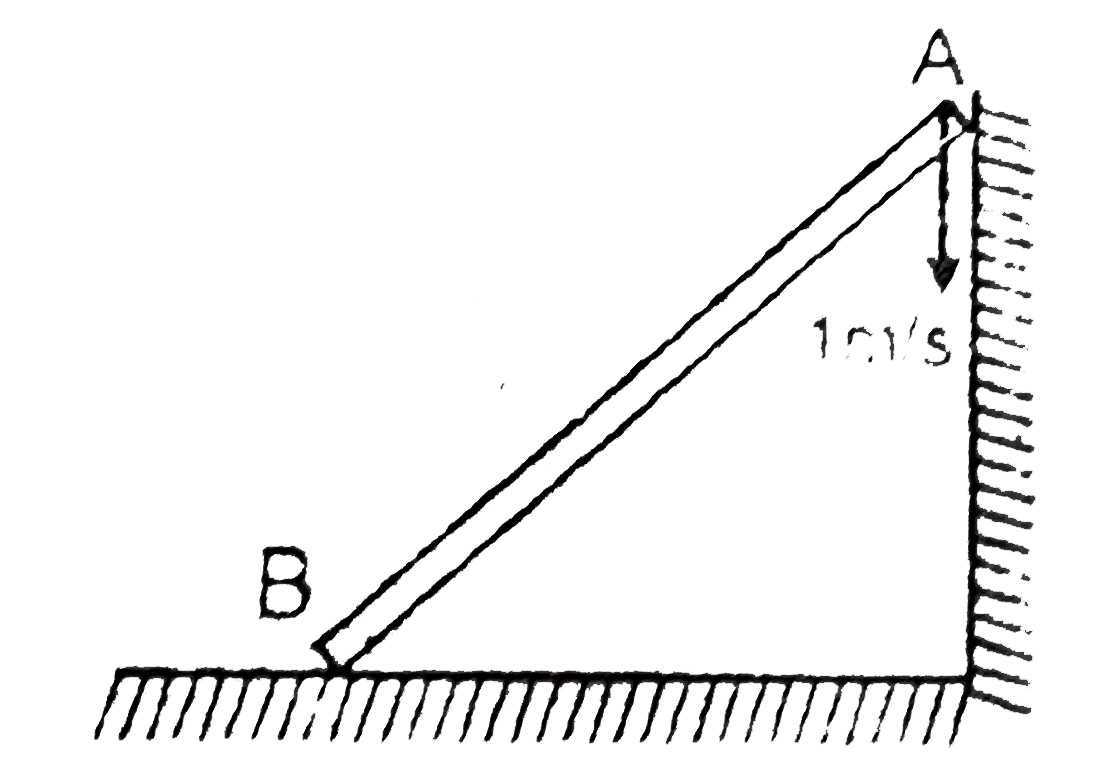
View Solution