The figure shows a system consisting of (i) a ring the outer radius 3R rolling clockwise without slipping on a horizontal surface with angular speed and (ii) an inner disc of radius 2R rotating anti clockwise with angular speed The ring and disc are separted. The point P on the inner disc is at a distance R from the origin, where OP makes an angle of with the horizontal. Then with respect to the horizontal surface,
The point has a linear velocity
The point has a linear velocity
The point has a linear velocity
The point has a linear velocity
The correct Answer is:A, B
To solve the problem, we need to determine the velocity of point P on the inner disc with respect to the horizontal surface. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the Components of the System
We have:
- A ring of outer radius 3R rolling clockwise with angular speed ω.
- An inner disc of radius 2R rotating anti-clockwise with angular speed ω2.
- Point P is located at a distance R from the origin, making an angle of 30∘ with the horizontal.
Step 2: Determine the Linear Velocity of the Ring
The linear velocity v of the center of the ring can be calculated using the formula:
v=R⋅ω
Since the radius of the ring is 3R, the linear velocity of the center of the ring is:
vring=3R⋅ω
This velocity acts in the negative x-direction (since it rolls clockwise).
Step 3: Calculate the Velocity of Point P on the Inner Disc
The point P on the inner disc is at a distance R from the center of the disc. The angular velocity of the inner disc is ω2. The linear velocity of point P due to the rotation of the disc is given by:
vP=R⋅ω2=Rω2
Since the disc rotates anti-clockwise, this velocity will have components in both the x and y directions.
Step 4: Resolve the Velocity of Point P into Components
The angle OP makes with the horizontal is 30∘. Therefore, we can resolve the velocity of point P into its x and y components:
- The x-component of the velocity:
vPx=Rω2⋅cos(30∘)=Rω2⋅√32=√3Rω4
- The y-component of the velocity:
vPy=Rω2⋅sin(30∘)=Rω2⋅12=Rω4
Step 5: Combine the Velocities
Now we combine the velocities of the ring and the point P:
- The total x-component of the velocity:
vtotalx=−3Rω+√3Rω4
- The total y-component of the velocity:
vtotaly=Rω4
Step 6: Final Velocity of Point P
The total velocity of point P with respect to the horizontal surface is:
vP=(−3Rω+√3Rω4)^i+Rω4^j
This simplifies to:
vP=(−12Rω4+√3Rω4)^i+Rω4^j
vP=(−(12−√3)Rω4)^i+Rω4^j
Conclusion
The final expression gives us the velocity of point P with respect to the horizontal surface.
We have:
- A ring of outer radius 3R rolling clockwise with angular speed ω.
- An inner disc of radius 2R rotating anti-clockwise with angular speed ω2.
- Point P is located at a distance R from the origin, making an angle of 30∘ with the horizontal.
The linear velocity v of the center of the ring can be calculated using the formula:
v=R⋅ω
Since the radius of the ring is 3R, the linear velocity of the center of the ring is:
vring=3R⋅ω
This velocity acts in the negative x-direction (since it rolls clockwise).
The point P on the inner disc is at a distance R from the center of the disc. The angular velocity of the inner disc is ω2. The linear velocity of point P due to the rotation of the disc is given by:
vP=R⋅ω2=Rω2
Since the disc rotates anti-clockwise, this velocity will have components in both the x and y directions.
The angle OP makes with the horizontal is 30∘. Therefore, we can resolve the velocity of point P into its x and y components:
- The x-component of the velocity:
vPx=Rω2⋅cos(30∘)=Rω2⋅√32=√3Rω4
- The y-component of the velocity:
vPy=Rω2⋅sin(30∘)=Rω2⋅12=Rω4
Now we combine the velocities of the ring and the point P:
- The total x-component of the velocity:
vtotalx=−3Rω+√3Rω4
- The total y-component of the velocity:
vtotaly=Rω4
The total velocity of point P with respect to the horizontal surface is:
vP=(−3Rω+√3Rω4)^i+Rω4^j
This simplifies to:
vP=(−12Rω4+√3Rω4)^i+Rω4^j
vP=(−(12−√3)Rω4)^i+Rω4^j
The final expression gives us the velocity of point P with respect to the horizontal surface.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - AR_TYPE2videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - LC_TYPE8videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - SCQ_TYPE29videos
RIGID BODY DYNAMICS 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
Similar Questions
A disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.
 View Solution
View SolutionA disc of mass m and radius R rotating with angular speed ω0 is placed on a rough surface (co-officient of friction =μ). Then
 View Solution
View SolutionA disc of mass M and Radius R is rolling with an angular speed ω on the horizontal plane. The magnitude of angular momentum of the disc about origin is:
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0 . We consider a point A on the surface of the disc. Then, the acceleration of point A is
View SolutionA uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is
 View Solution
View SolutionAs shown in the figure, a disc of mass m is rolling without slipping with angular velocity ω. When it crosses point B disc will be in
 View Solution
View SolutionA disc of radius R is rolling purely on a flat horizontal surface, with a constant angular velocity. The angle between the velocity ad acceleration vectors of point P is
 View Solution
View SolutionA disc of radius R is rolling purely on a flat horizontal surface, with a constant angular velocity. The angle between the velocity and acceleration vectors of point P is
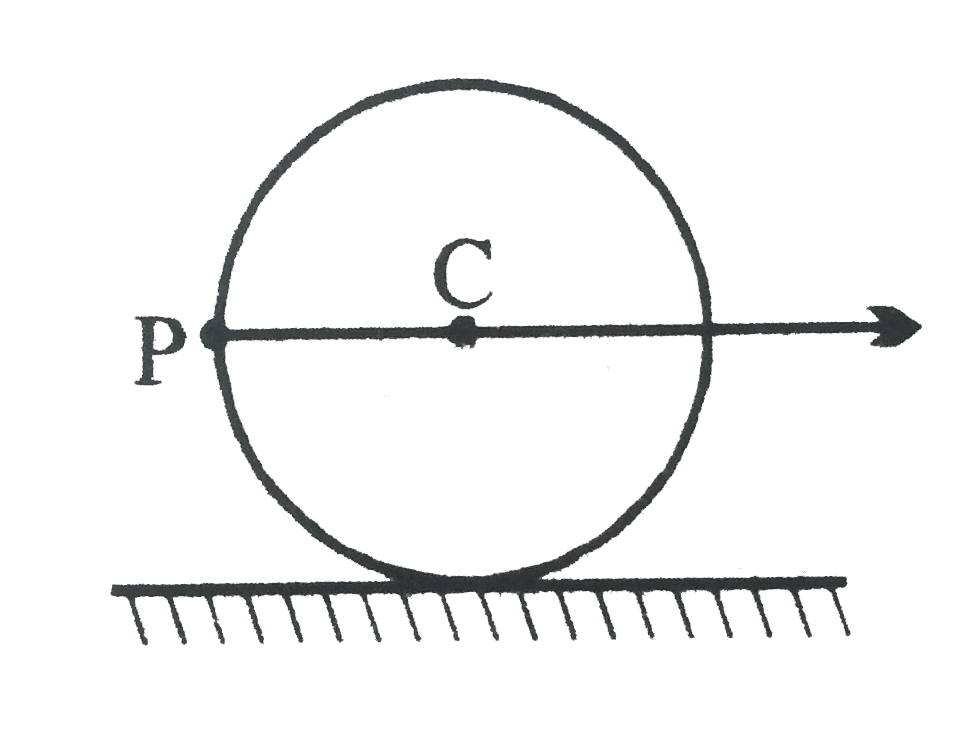 .View Solution
.View Solution
A disc of radius R is rolling purely on a flat horizontal surface, with a constant angular velocity the angle between the velocity ad acceleration vectors of point P isView Solution
A ring of radius R rolls on a horizontal surface with constant acceleration a of the centre of mass as shown in figure. If ω is the instantaneous angular velocity of the ring. Then the net acceleration of the point of contact of the ring with gound isView Solution
