The friction coefficient between the two blocks shown in figure is μ but the floor is smooth. A. What maximum horizontal force F can be applied without disturbing the equilibrium of the systme? b.suppose the horizontal force applied is double of that found in part a. Findthe accelerations of the two masses.
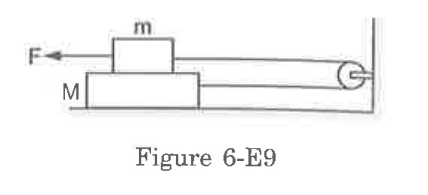
Both upper block andlower block will have acceleration 2ms2

R1=mgF=μR1−T=0
→T−μR1=0
andF--mu mg =T…..ii<br>rarr T=mu mg<br>Pu∈gT=mu mg<br>F=mu mg +mu mg = 2mu mg<br>b.2F=T-mumg -ma<br>=0.........i<br>T-ma-mu mg =0 [:.R=mg]<br>rarr T=ma+mumg<br>Pu∈gvalueofT∈i.wehave<br>2F-Mamu mg +mu mg-ma=0<br>Pu∈gF=2mumg<br>2(2μmg)−2μmg=a(M+m)
→4μmg−2μmg=a(M+m)
→a=2μmgM+m
Both blocks move with this acceleration a in opposite direction.
Topper's Solved these Questions
Similar Questions
The coefficient of static friction between the two blocks shown in figure is μ and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?

View SolutionThe coefficient of static friction between two blocks is 0.5 and the table is smooth. The maximum horizontal force that can be applied to move the blocks together is N. (take g=10ms−2)

View Solution
Knowledge Check
Coefficient of static friction between the two blocks in Fig. 7.124 is μ and the table is frictionless . Maximum horizontal force which can be applied on the lower such that the blocks move together , is :
 Aμm1gBμm2gCμ(m1−m2)gDμ(m1+m2)g
Aμm1gBμm2gCμ(m1−m2)gDμ(m1+m2)gThe friction coefficient between the plank and floor is μ. The man applies, the maximum possible force on the string and the system remains at rest. Then :
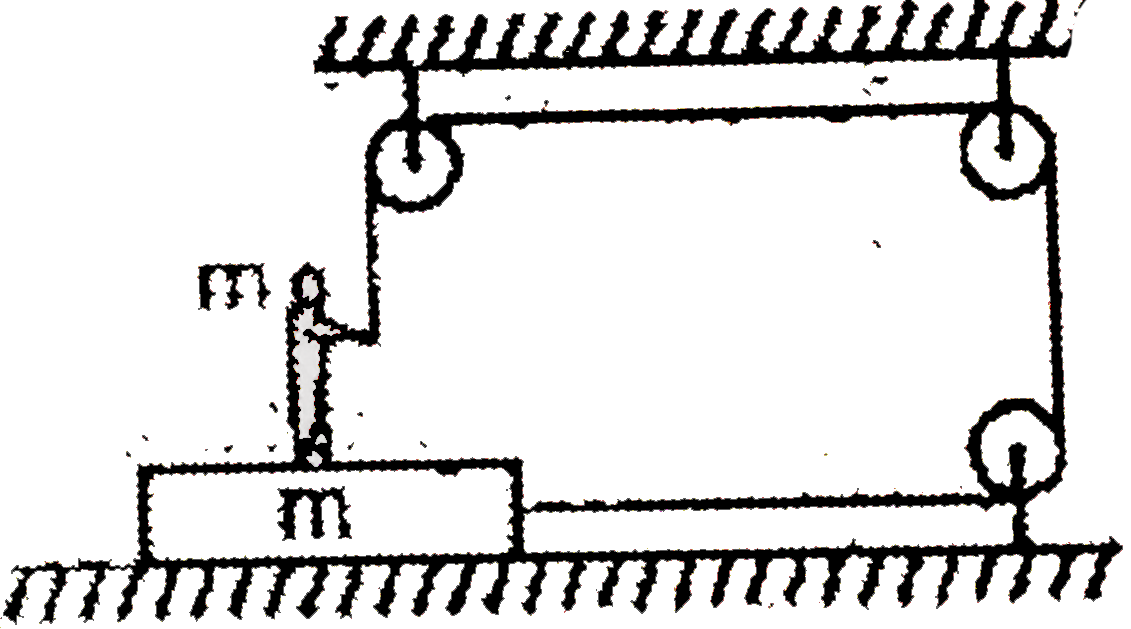 Afriction force between plank and surface is 2μmg1+μBfrictional force on man is zeroCtension in the string is 2μmg1+μDnet force on man is zero
Afriction force between plank and surface is 2μmg1+μBfrictional force on man is zeroCtension in the string is 2μmg1+μDnet force on man is zeroEach of the two block shown in the figure has mass m. The pulley is smooth and the coefficient of friction for all surfaces in contact is μ. A constant horizontal force P applied in two cases shown in such a way that block A start just sliding then the value of minimum force P in case-I and case-II is :
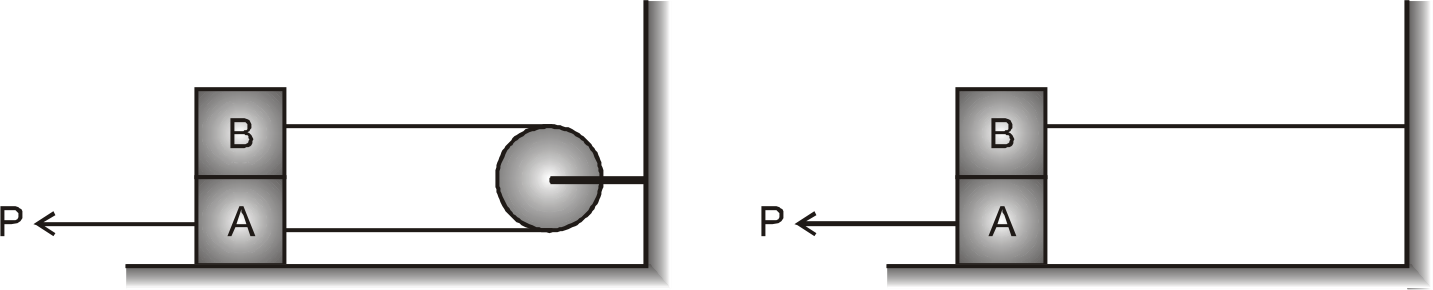 A2μmg,3μmgB3μmg,2μmgC4μmg,3μmgD3μmg,3μmg
A2μmg,3μmgB3μmg,2μmgC4μmg,3μmgD3μmg,3μmg
The friction coefficient between the blocks is μ. If force F is applied on the lower block , analyse motion of two blocks.
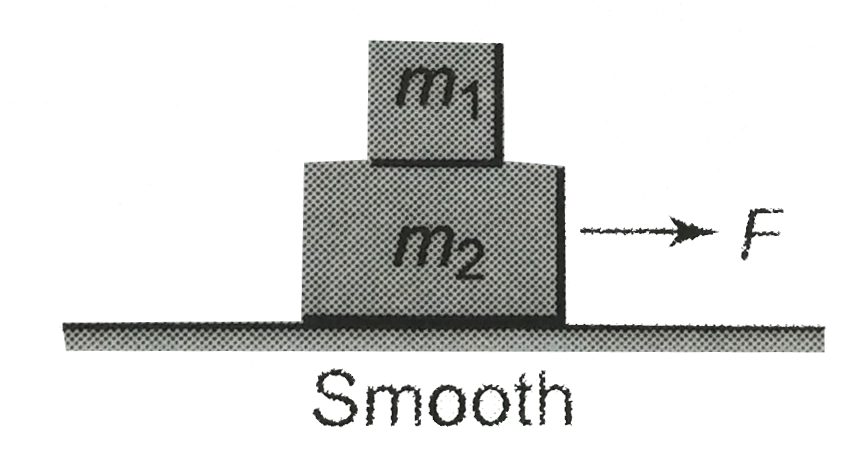
View SolutionBlock B of mass m has been placed on block A of mass 3 m as shown. Block A rests on a smooth horizontal table. F1 is the maximum horizontal force that can be applied on the block A such that there is no slipping between the blocks. Similarly, F2 is the maximum horizontal force that can be applied on the block B so that the two blocks move together without slipping on each other. When F1andF2 both are applied together as shown in figure.
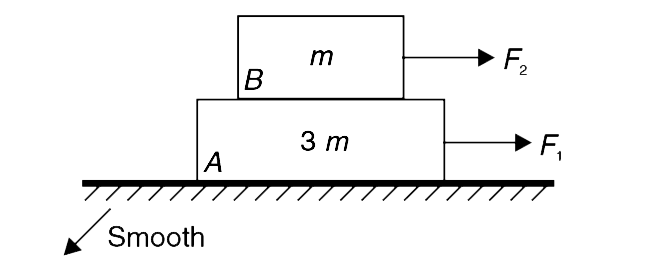
(a) Find the friction force acting between the blocks.
(b) Acceleration of the two blocks.
(c) If F2 is decreased a little, what will be direction of friction acting on B.
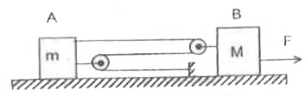
View SolutionTwo blocks A and B of masses m and M are attached with ideal string and pulley, and placed on smooth horizontal surface. Force F is applied horizontally as shown in figure. Find the ratio of the acceleration of A to that of B.
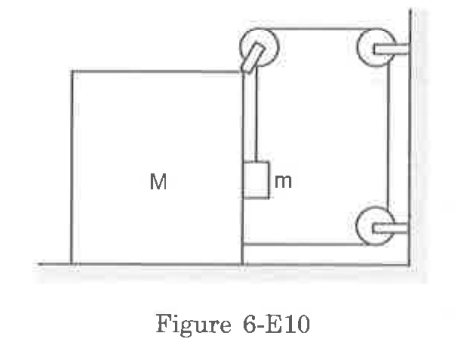
View SolutionA block of mass m slips on a rough horizontal table under the action of horiozontal force applied to it. The coefficient of friction between the block and the table is μ. The table does not move on the floor. Find the total frictional force aplied by the floor on the legs of the table. Do you need the friction coefficient between the table and the floor or the mass of the table ?
View Solution
Two blocks A and B are shown in figure. The minimum horizontal force F applied on Blocks B for which slipping begins at B and ground is
View Solution
