The linear momentum is conserved in
elastic collisions
inelastic collisions
Both a and B
Neither a nor b
The correct Answer is:C
To solve the question regarding the conservation of linear momentum in different types of collisions, we can follow these steps:
Step 1: Understand the Concept of Linear Momentum
Linear momentum (p) is defined as the product of an object's mass (m) and its velocity (v). Mathematically, it is expressed as:
p=m⋅v
Step 2: Identify Types of Collisions
There are two main types of collisions:
- Elastic Collisions: In these collisions, both momentum and kinetic energy are conserved.
- Inelastic Collisions: In these collisions, momentum is conserved, but kinetic energy is not.
Step 3: Apply the Law of Conservation of Momentum
The law of conservation of momentum states that in a closed system (where no external forces are acting), the total momentum before the collision is equal to the total momentum after the collision. This applies to both types of collisions:
- For elastic collisions:
pinitial=pfinal
- For inelastic collisions:
pinitial=pfinal
Step 4: Analyze the Options
Given the options:
1. Elastic collisions
2. Inelastic collisions
3. Both A and B
4. Neither A nor B
Since we have established that linear momentum is conserved in both elastic and inelastic collisions, we can conclude that:
Step 5: Choose the Correct Option
The correct answer is:
Both A and B (Option 3)
Summary
Linear momentum is conserved in both elastic and inelastic collisions.
---
Linear momentum (p) is defined as the product of an object's mass (m) and its velocity (v). Mathematically, it is expressed as:
p=m⋅v
There are two main types of collisions:
- Elastic Collisions: In these collisions, both momentum and kinetic energy are conserved.
- Inelastic Collisions: In these collisions, momentum is conserved, but kinetic energy is not.
The law of conservation of momentum states that in a closed system (where no external forces are acting), the total momentum before the collision is equal to the total momentum after the collision. This applies to both types of collisions:
- For elastic collisions:
pinitial=pfinal
- For inelastic collisions:
pinitial=pfinal
Given the options:
1. Elastic collisions
2. Inelastic collisions
3. Both A and B
4. Neither A nor B
The correct answer is:
Both A and B (Option 3)
Linear momentum is conserved in both elastic and inelastic collisions.
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Match the coloumns
9 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
A ballistic pendulum is a device that was used to measure the speeds of bullets before the development of electronic tiring, devices. The device consists of a large block of wood of mass M, hanging from two long cords. A bullet of mass m is fired into the block. the bullet comes quickly into rest and the block + bullet rises to a vertical distance h before the pendulum comes momentarily to rest as the ends of the arc. ltbr> In the process. the linear momentum is conserved. In such a collision. some kinetic energy is dissipated as heat: so mechanical energy is not conserved. When there is a loss in mechanical energy, the collision is said to be inelastic. Further when two bodies coalesce, the collision is said to be perfectly inelastic.
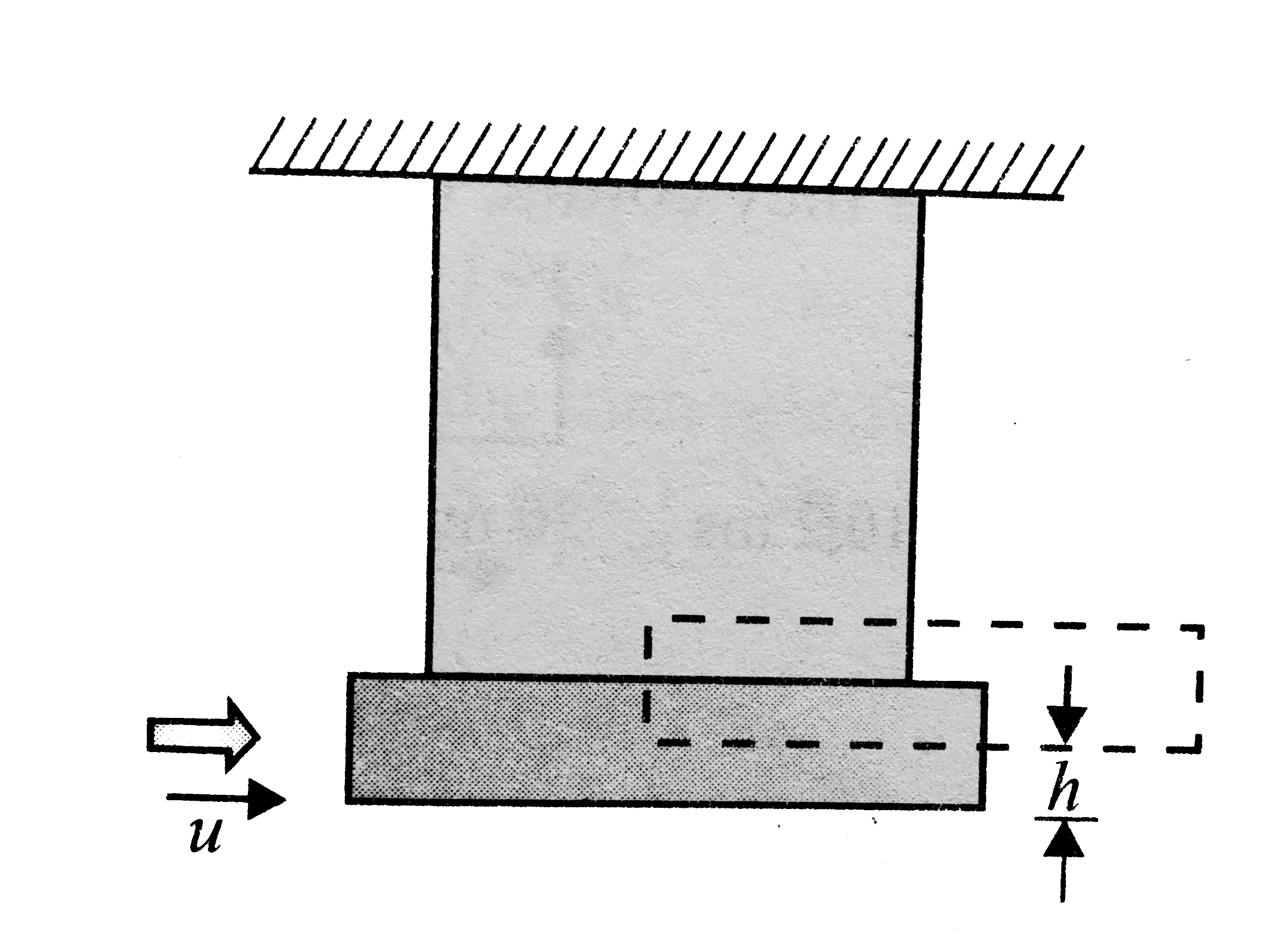
The energy dissipated as heat in the collision is
View SolutionA ballistic pendulum is a device that was used to measure the speeds of bullets before the development of electronic tiring, devices. The device consists of a large block of wood of mass M, hanging from two long cords. A bullet of mass m is fired into the block. the bullet comes quickly into rest and the block + bullet rises to a vertical distance h before the pendulum comes momentarily to rest as the ends of the arc. ltbr> In the process. the linear momentum is conserved. In such a collision. some kinetic energy is dissipated as heat: so mechanical energy is not conserved. When there is a loss in mechanical energy, the collision is said to be inelastic. Further when two bodies coalesce, the collision is said to be perfectly inelastic.
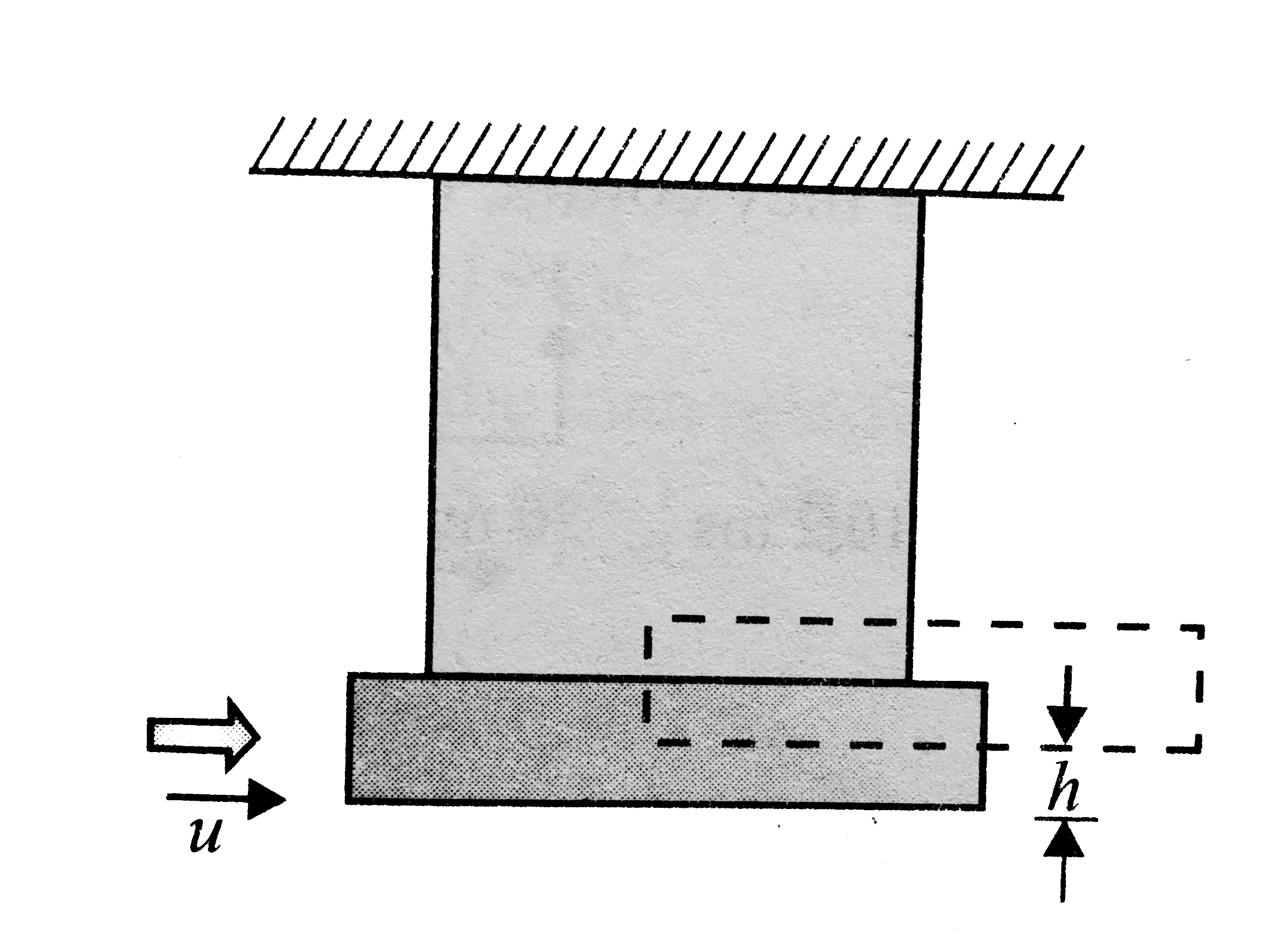
The collision of block-bullet system is
View Solution
Knowledge Check
Assertion: The conservation of kinetic energy in elastic collision applies after the collision is over and does not hold at every instant of the collision.
Reason: During a collision the total linear momentum is conserved at each instant of the collision.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true not but reason is not the correct explanation of assertion.CIf assertion is true but reason is falseDIf both assertion and reason are false.
A ballistic pendulum is a device that was used to measure the speeds of bullets before the development of electronic tiring, devices. The device consists of a large block of wood of mass M, hanging from two long cords. A bullet of mass m is fired into the block. the bullet comes quickly into rest and the block + bullet rises to a vertical distance h before the pendulum comes momentarily to rest as the ends of the arc. ltbr> In the process. the linear momentum is conserved. In such a collision. some kinetic energy is dissipated as heat: so mechanical energy is not conserved. When there is a loss in mechanical energy, the collision is said to be inelastic. Further when two bodies coalesce, the collision is said to be perfectly inelastic.
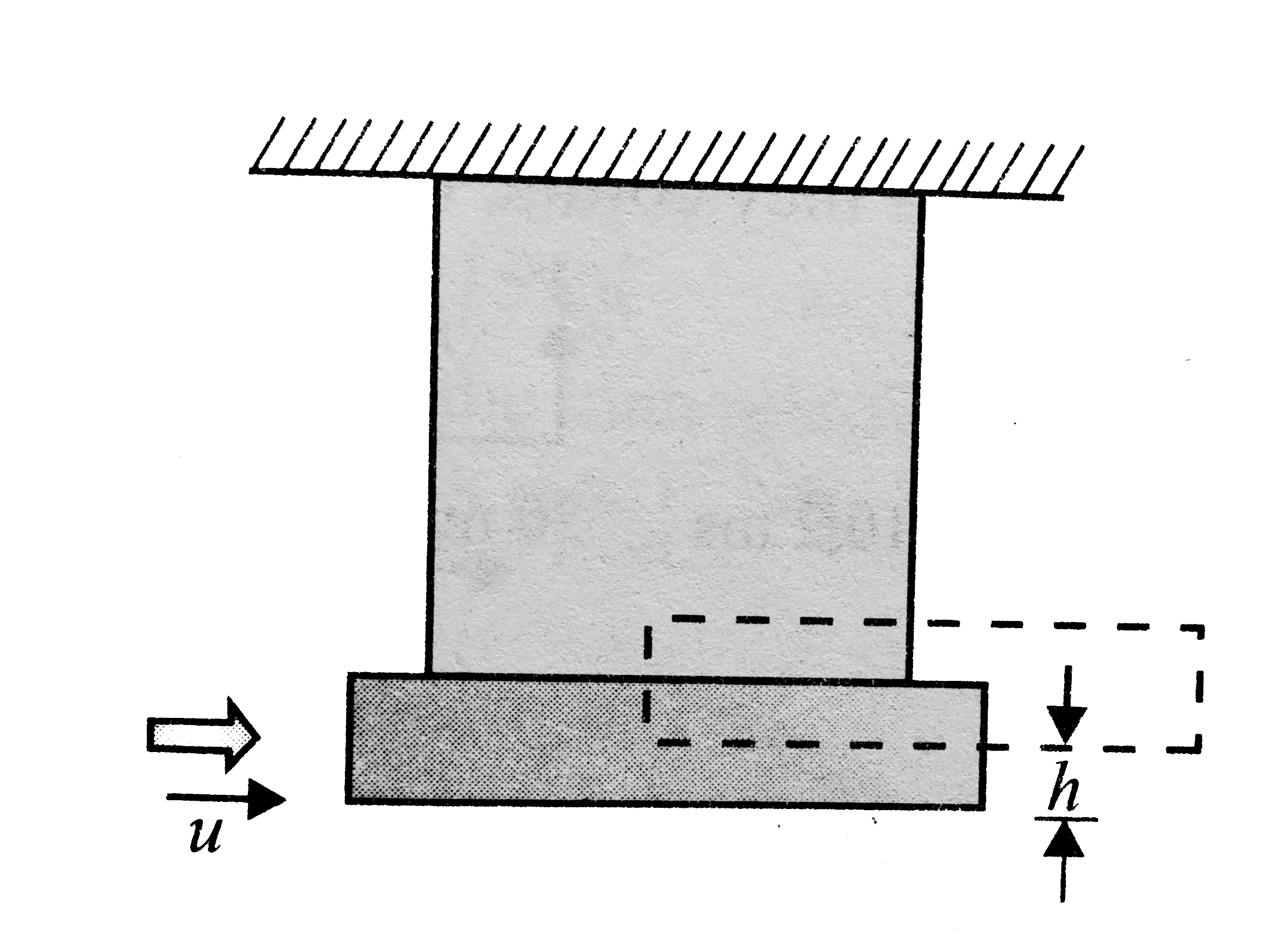
After collision what is the combined velocity of the bullet + block system?
View SolutionA ballistic pendulum is a device that was used to measure the speeds of bullets before the development of electronic tiring, devices. The device consists of a large block of wood of mass M, hanging from two long cords. A bullet of mass m is fired into the block. the bullet comes quickly into rest and the block + bullet rises to a vertical distance h before the pendulum comes momentarily to rest as the ends of the arc. ltbr> In the process. the linear momentum is conserved. In such a collision. some kinetic energy is dissipated as heat: so mechanical energy is not conserved. When there is a loss in mechanical energy, the collision is said to be inelastic. Further when two bodies coalesce, the collision is said to be perfectly inelastic.
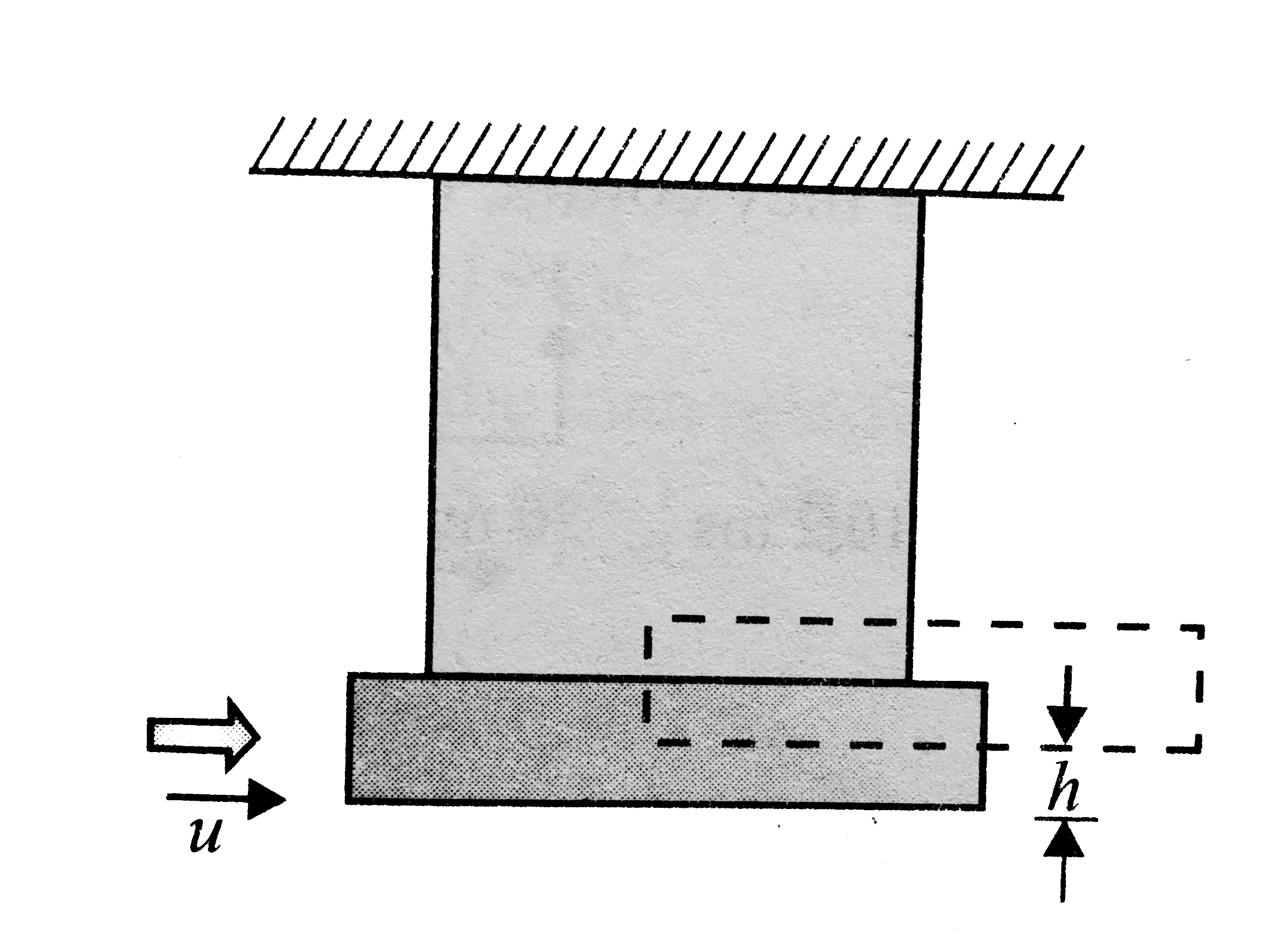
What is the initial speed of the bullet in terms of height h?
View SolutionAssertion: There is not loss in energy in elastic collision.
Reason: Linear momentum is conserved in elastic collision.
View SolutionAngular momentum is conserved
View SolutionA planet is revolving around the sun in an elliptcal orbit. Its KE is different for different points and the total energy is negative. Its linear momentum is not conserved the eccentricity decides the shape of the orbit.
Linear momentum of the planet is
View SolutionA planet is revolving around the sun in an elliptcal orbit. Its KE is different for different points and the total energy is negative. Its linear momentum is not conserved the eccentricity decides the shape of the orbit.
Net torque on the planet is
View SolutionA planet is revolving around the sun in an elliptcal orbit. Its KE is different for different points and the total energy is negative. Its linear momentum is not conserved the eccentricity decides the shape of the orbit.
Velocity of the planet is minimum at
View Solution
