The magnetic moment of a current I carrying circular coil of radius r and number of turns N varies as
1r2
1r
r
r2
The correct Answer is:D
To find how the magnetic moment m of a current-carrying circular coil varies with the radius r, number of turns N, and current I, we can follow these steps:
Step 1: Understand the Formula for Magnetic Moment
The magnetic moment m of a circular coil is given by the formula:
m=N⋅I⋅A
where:
- N is the number of turns,
- I is the current flowing through the coil,
- A is the area of the coil.
Step 2: Calculate the Area of the Coil
The area A of a circular coil with radius r is calculated using the formula for the area of a circle:
A=πr2
Step 3: Substitute the Area into the Magnetic Moment Formula
Substituting the expression for area A into the magnetic moment formula:
m=N⋅I⋅(πr2)
Step 4: Simplify the Expression
This can be simplified to:
m=N⋅I⋅π⋅r2
From this expression, we can see that the magnetic moment m is directly proportional to r2:
m∝r2
Step 5: Conclusion
Thus, we conclude that the magnetic moment of a current-carrying circular coil varies as the square of the radius r. Therefore, the answer to the question is:
m∝r2
The magnetic moment m of a circular coil is given by the formula:
m=N⋅I⋅A
where:
- N is the number of turns,
- I is the current flowing through the coil,
- A is the area of the coil.
The area A of a circular coil with radius r is calculated using the formula for the area of a circle:
A=πr2
Substituting the expression for area A into the magnetic moment formula:
m=N⋅I⋅(πr2)
This can be simplified to:
m=N⋅I⋅π⋅r2
From this expression, we can see that the magnetic moment m is directly proportional to r2:
m∝r2
Thus, we conclude that the magnetic moment of a current-carrying circular coil varies as the square of the radius r. Therefore, the answer to the question is:
m∝r2
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - HOTS
5 Videos
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - EXEMPLAR PROBLEMS
6 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
- NUCLEIBOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - Assertion And Reason
15 Videos
Similar Questions
The magneitc field produced at the center of a current carrying circular coil of radius r, is
View Solution(a) Using Biot-Savart's law, derive an expression for the magnetic field at the centre of a circular coil of radius R, number of turns N, carrying current.
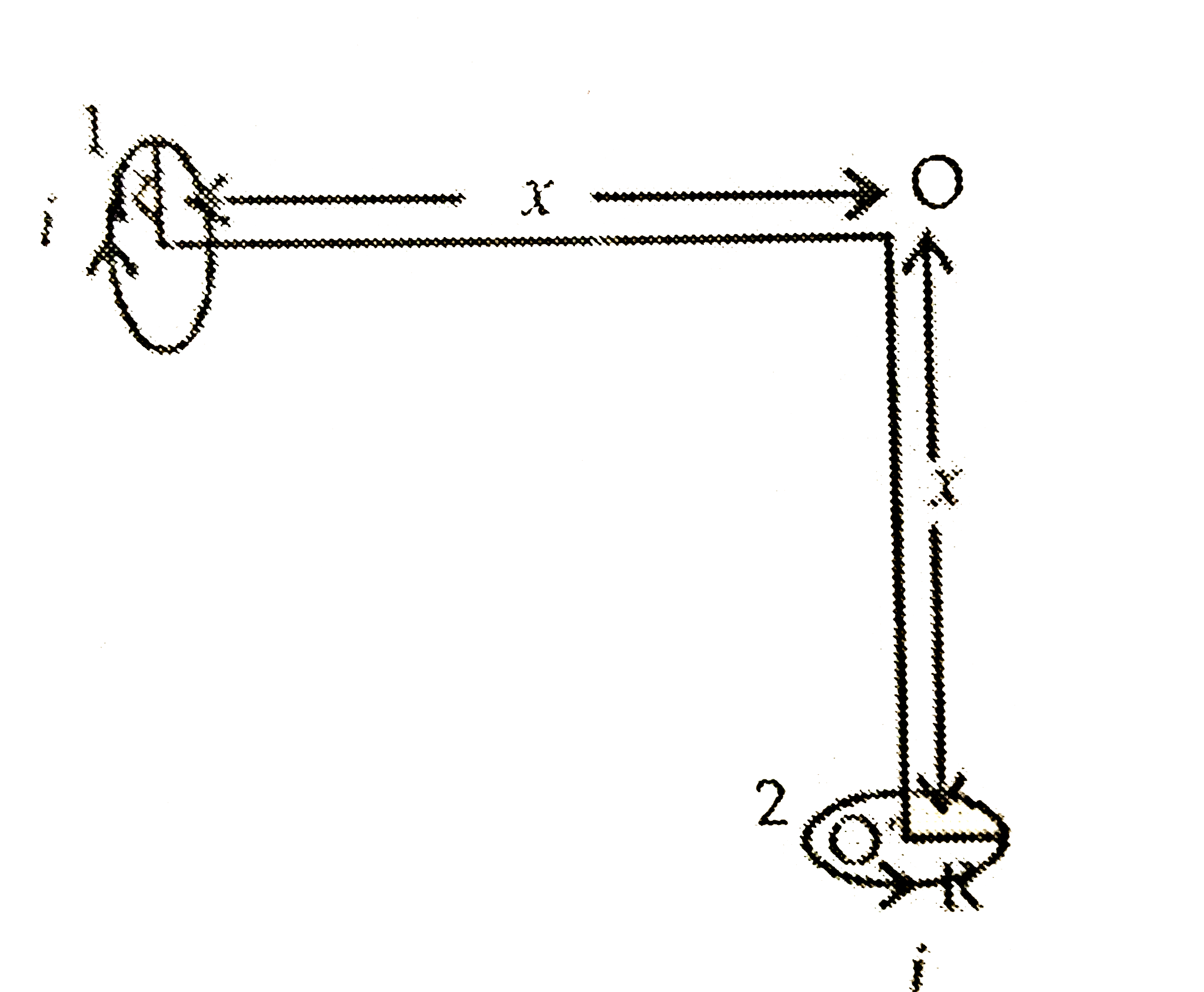
(b) Two small identical circular coils marked 1,2 carry equal currents and are placed with their geometric axes perpendicular to each other as shown in the figure. Derive an expression for the resultant magnetic field at O.
View SolutionWhat will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionUse Biot-Savart law to derive the expression for the magnetic field on the axis of a current carrying circular loop of radius R.
Draw the magnetic field lines due to circular wire carrying current I.
View SolutionRatio of magnetic field at the centre of a current carrying coil of radius R and at a distance of 3R on its axis is
View SolutionThe ratio of the magnetic field at the centre of a current carrying circular coil to its magnetic moment is x. If the current and radius both are doubled the new ratio will become
View SolutionThe ratio of the magnetic field at the centre of a current carrying circular coil to its magnetic moment is x. If the current and radius both are doubled the new ratio will become
View SolutionObtain an expression for magnetic flux density B at the centre of a circular coil of radius R, having N turns and carrying a current I.
View SolutionObtain an expression for magnetic flux density B at the centre of a circular coil of radius R, having N turns and carrying a current I.
View SolutionIf the current (I) flowing through a circular coil, its radius (R ) and number of turns (N) in it are each doubled, magnetic flux density at its centre becomes:
View Solution
