The magnitude of the force (in newtons) acting on a body varies with time t (in micro seconds) as shown in the fig AB, BC and CD are straight line segments. The magnitude of the total impulse of the force on the body from t=4μs to t=16μs is ….Ns
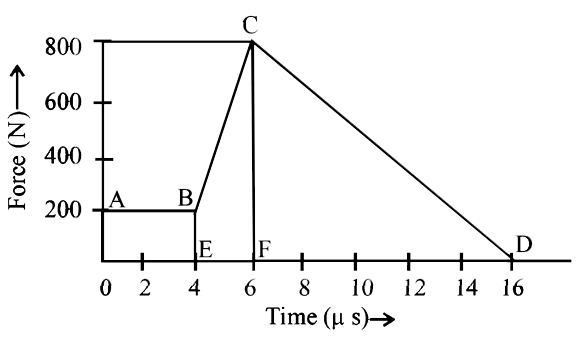
Area under the F−t graph gives the
impulse imparted to the body.
The magnitude of total impulse of force on the body from
t=4μs→t=16μs
=area(BCDFEB)
=areaofBCDFEB+areaCDFC
=12(200+800)×2×10−6+12×10×800×10−6
=0.001+0.004=0.005Ns
Topper's Solved these Questions
Similar Questions
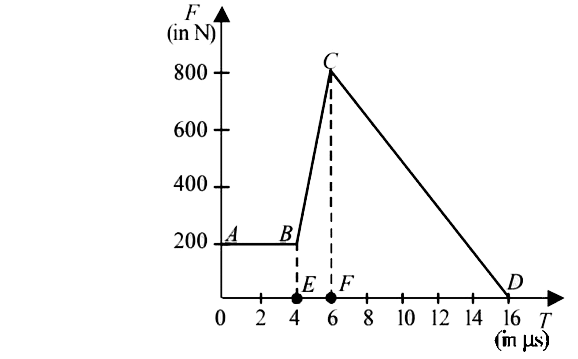
The magnitude of force (in Newtons) acting on a body varies with time (in micro second) as shown in the figure. The magnitude of total impulse of the force on the body from t = 4μs to t = 16μs is –
 View Solution
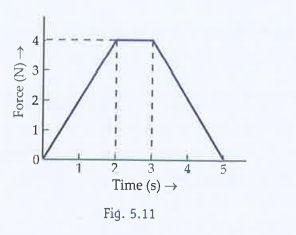
View SolutionA force acting on a body of mass 2 kg varies with time as shown in fig.5.11. Find impulse of the force.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
The displacement y (in metre) of a body varies with time t (in seconds) as y=−23t216t+2. The body comes to rest in a time
A8sB10sC12sD14s - Question 2 - Select One or More
Power of a force acting on a block varies with time t as shown in figure. Then, angle between force acting on the block and its velocity is
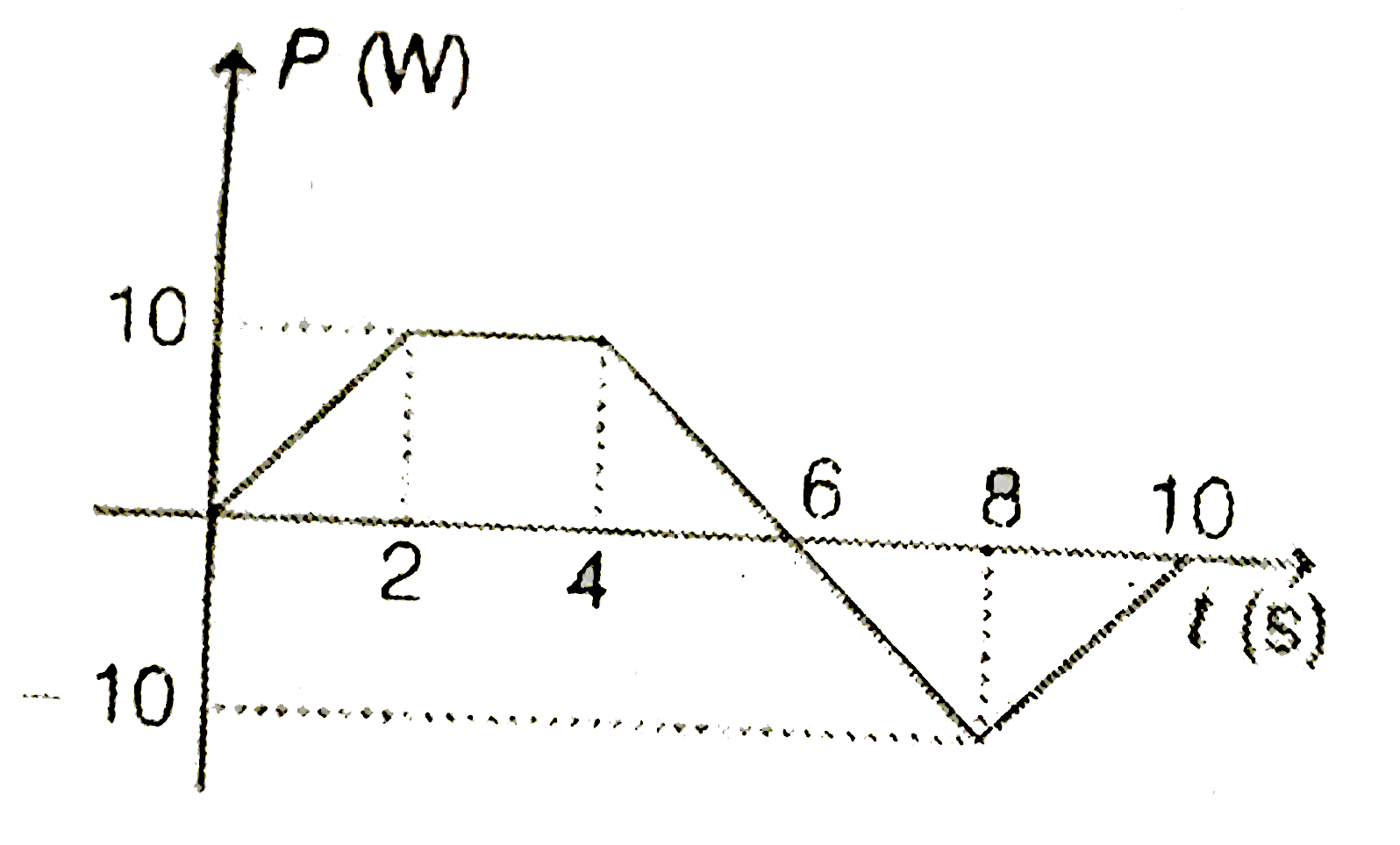 Aacute at = 1 sB90∘ at t = 3 sCobtuse at t = 7 sDchange in kinetic energy from t = 0, to t = 10 s is 20 J
Aacute at = 1 sB90∘ at t = 3 sCobtuse at t = 7 sDchange in kinetic energy from t = 0, to t = 10 s is 20 J - Question 3 - Select One or More
Kinetic energy of a particle moving in a straight line is proportional to the time t. The magnitude of the force acting on the particle is :
ADirectly proportional to the speed of the particleBInversely proportional to √tCInversely proportional to the speed of the particleDDirectly proportional to √t
The magnitude of force acting on a particle moving along x-axis varies with time (t) as shown in figure. If at t = 0 the velocity of particle is v0, then its velocity at t=T0 will be
 View Solution
View SolutionA force acting on a body of mass 2 kg varies with time as shown in fig . 20 find impulse of the force and final velocity of the body.
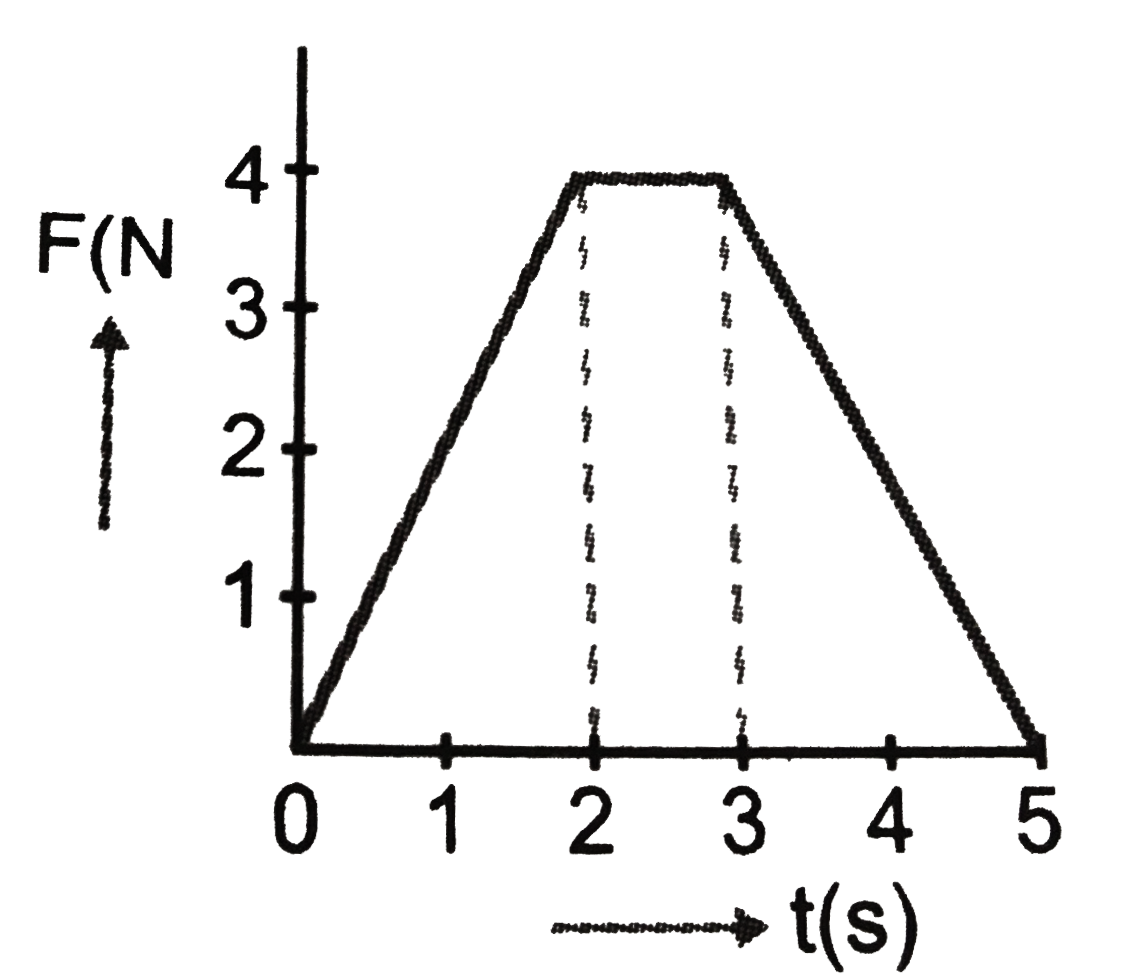 View Solution
View SolutionIf the kinetic energy of a body is directly proportional to time t, the magnitude of the force acting on the body is
View SolutionThe displacement y (in metres) of a body varies with time t ( in seconds ) as y=−23t2+16t−12 . How long does the body take to come to rest ?
View SolutionA unidirectional force F varying with time t as shown in the Fig. acts on a body initially at rest for a short duration 2T. Then the velocity acquired by the body is
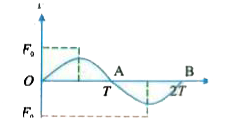 View Solution
View Solution

