Question

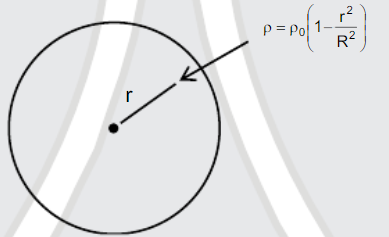
The mass density of a planet of radius R varie with the distance r form its centre as $\rho \left(r\right)=p_0(1-\frac{r^2}{R^2})$. Then the graviational field is maximum at :

Options

A. ` r = sqrt((5)/(9))R`
B. ` r= (1)/(sqrt(3)) R`
C. `r = R`
D. ` r = sqrt((3)/(4))R`

Explanation

To find the radius at which the gravitational field is maximum for a planet with a given density variation, we can follow these steps:

Step 1: Understand the Density Function
The mass density of the planet varies with the distance \( r \) from its center as:
\( \rho(r) = \rho_0 \left( 1 - \frac{r^2}{R^2} \right) \)
where \( \rho_0 \) is the density at the center and \( R \) is the radius of the planet.

Step 2: Calculate the Mass Enclosed within Radius \( r \)
To find the gravitational field, we first need to calculate the mass enclosed within a radius \( r \):
\( M(r) = \int_0^r \rho(r') \cdot dV \)
The volume element in spherical coordinates is \( dV = 4\pi r'^2 dr' \). Thus,
\( M(r) = \int_0^r \rho_0 \left( 1 - \frac{(r')^2}{R^2} \right) 4\pi (r')^2 dr' \)

Step 3: Evaluate the Integral
Now, we evaluate the integral:
\( M(r) = 4\pi \rho_0 \int_0^r \left( (r')^2 - \frac{(r')^4}{R^2} \right) dr' \)
Calculating the two parts of the integral:
1. \(\int_0^r (r')^2 dr' = \frac{r^3}{3}\)
2. \(\int_0^r (r')^4 dr' = \frac{r^5}{5}\)

Thus,
\( M(r) = 4\pi \rho_0 \left( \frac{r^3}{3} - \frac{r^5}{5R^2} \right) \)

Step 4: Calculate the Gravitational Field
The gravitational field \( g(r) \) at a distance \( r \) from the center is given by:
\( g(r) = \frac{G M(r)}{r^2} \)
Substituting \( M(r) \):
\( g(r) = \frac{G \cdot 4\pi \rho_0 \left( \frac{r^3}{3} - \frac{r^5}{5R^2} \right)}{r^2} \)
Simplifying this gives:
\( g(r) = \frac{4\pi G \rho_0}{3} r - \frac{4\pi G \rho_0}{5R^2} r^3 \)

Step 5: Find the Maximum Gravitational Field
To find the radius \( r \) at which \( g(r) \) is maximum, we differentiate \( g(r) \) with respect to \( r \) and set it to zero:
\( \frac{dg}{dr} = \frac{4\pi G \rho_0}{3} - \frac{12\pi G \rho_0}{5R^2} r^2 = 0 \)
Solving for \( r \):
\( \frac{4\pi G \rho_0}{3} = \frac{12\pi G \rho_0}{5R^2} r^2 \)
\( r^2 = \frac{4R^2}{5} \)
\( r = \frac{2R}{\sqrt{5}} \)

Conclusion
The gravitational field is maximum at:
\( r = \frac{2R}{\sqrt{5}} \)