The resultant R of two forces P and Q acting at an angle θ (obtuse angle i.e. θ>90∘) is given by
R2=p2+q2+2PQcosθ
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - NUMERICAL EXXERCISE (LEVEL -2)21videos
ELEMENTS OF VECTORS
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS10videos
ELEMENTS OF VECTORS
- BOOK - AAKASH SERIESCHAPTER - ELEMENTS OF VECTORSEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS12videos
ELEMENTS OF VECTORS
- BOOK - AAKASH SERIESCHAPTER - ELECTROSTATICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)5videos
ELECTROSTATICS
- BOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)10videos
GEOMETRICAL OPTICS
Similar Questions
Knowledge Check
- Question 1 - Select One
The resultant of two forces acting at an angle of 120∘ is 10 N. If one of the force is 10 N. The other force is
A20/√3 NB10√3 NC10 ND20√3 N
If the resultannt of two forces of magnitudes P and Q acting at a point at an angle of 60∘ is √7Q, then P/Q is
View SolutionThe resultant of two forces acting at an angle of 150° 10 kgwt, and is perpendicular to one of the forces. The magnitude of smaller force is
View SolutionTwo forces of magnitudes P and Q are inclined at an angle (θ). The magnitude of their resultant is 3Q. When the inclination is changed to (180∘−θ), the magnitude of the resultant force becomes Q. Find the ratio of the forces.
View SolutionWhen two vectors of magnitudes P and Q are inclined at an angle θ, the magnitudes of their resultant is 2P. When the inclination is changed to 180∘−θ, the magnitudes of the resultant is halved. Find the ratio of P and Q.
View SolutionThe greatest & the least magnitude of the resultant of two forces of constant magnitudes are P & Q respectively. If the forces act at an angle 2α, then prove that the magnitude of their resultant is given by √P2cos2α+Q2sin2α.
View SolutionThe reflecting surface of two intersecting flat mirrors are at an angle θ(0∘<θ<90∘). As shown in Figure. For a light ray that strikes the horizontal mirror, the emerging ray intersects the incident ray at an angle β . If one of the mirrors is rotated at dθdt=1∘/s, then the angle β changes aat the rate of
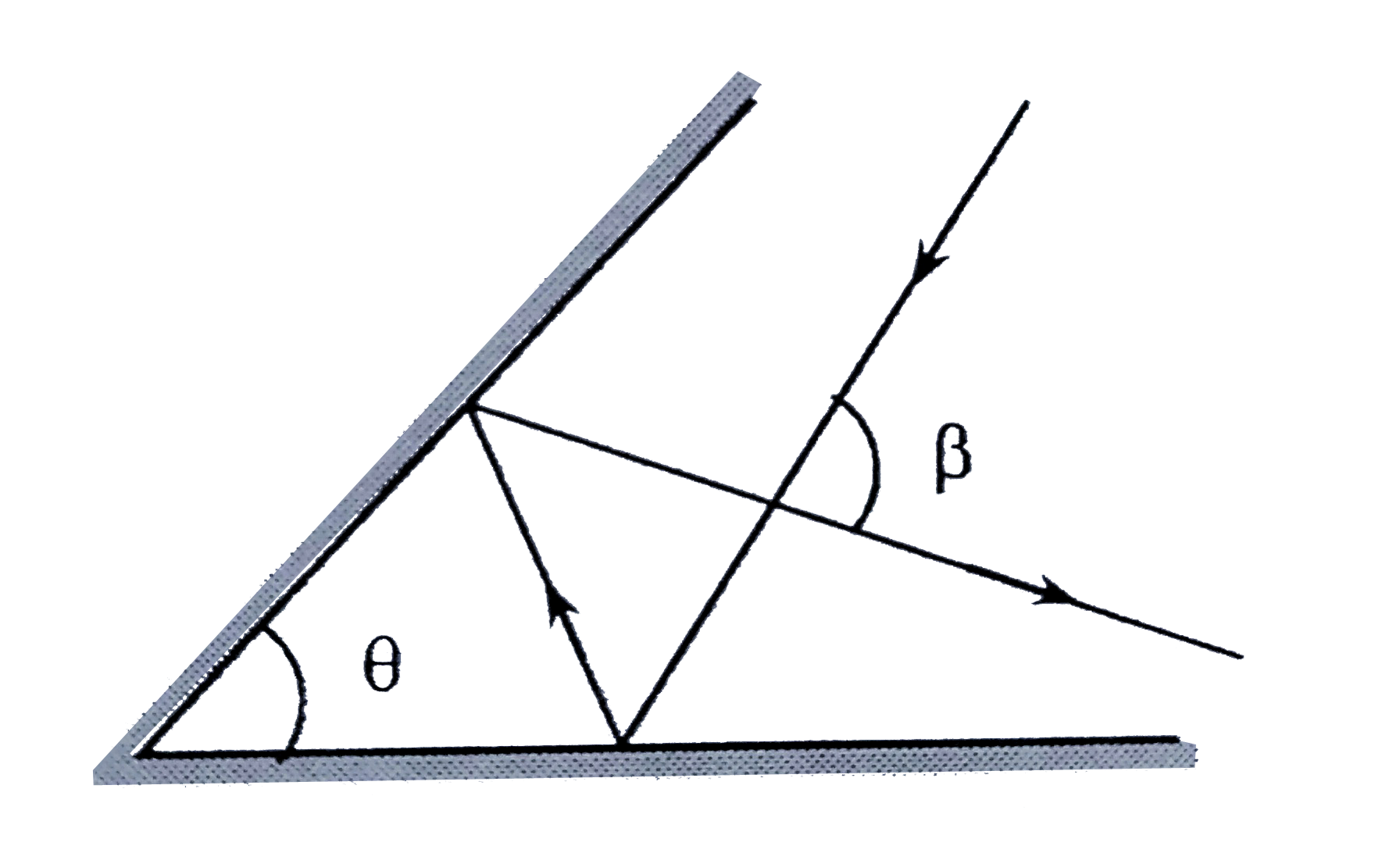 View Solution
View SolutionThe resultant R of vector P andQ is perpendicular to P and R=P both , then angle betwwen |P|and|Q|is
View Solution
