The slope of kinetic energy displacement curve of a particle in motion is
equal to the acceleration of the particle
directly proportional to the acceleration of the particle
inversely proportional to the acceleration of the particle
None of the above
The correct Answer is:B
To solve the question regarding the slope of the kinetic energy displacement curve of a particle in motion, we can follow these steps:
1. Understand Kinetic Energy Formula:
The kinetic energy (KE) of a particle is given by the formula:
KE=12mv2
where m is the mass of the particle and v is its velocity.
2. Relate Velocity to Displacement:
Velocity can be expressed as the derivative of displacement with respect to time:
v=dxdt
3. Differentiate Kinetic Energy with Respect to Displacement:
To find the slope of the kinetic energy displacement curve, we need to differentiate the kinetic energy with respect to displacement x:
d(KE)dx=ddx(12mv2)
4. Apply the Chain Rule:
Using the chain rule, we can differentiate v2 with respect to x:
d(KE)dx=12m⋅2v⋅dvdx=mvdvdx
5. Relate dvdx to Acceleration:
We know that acceleration a is defined as:
a=dvdt
and from the chain rule, we can express dvdx as:
dvdx=dvdt⋅dtdx=a⋅1v
6. Substitute Back into the Equation:
Now substitute dvdx back into the equation for the slope:
d(KE)dx=mv(a⋅1v)=ma
7. Conclusion:
The slope of the kinetic energy displacement curve is:
d(KE)dx=ma
This shows that the slope is directly proportional to the acceleration a of the particle.
Final Answer:
The slope of the kinetic energy displacement curve of a particle in motion is directly proportional to the acceleration of the particle.
The kinetic energy (KE) of a particle is given by the formula:
KE=12mv2
where m is the mass of the particle and v is its velocity.
Velocity can be expressed as the derivative of displacement with respect to time:
v=dxdt
To find the slope of the kinetic energy displacement curve, we need to differentiate the kinetic energy with respect to displacement x:
d(KE)dx=ddx(12mv2)
Using the chain rule, we can differentiate v2 with respect to x:
d(KE)dx=12m⋅2v⋅dvdx=mvdvdx
We know that acceleration a is defined as:
a=dvdt
and from the chain rule, we can express dvdx as:
dvdx=dvdt⋅dtdx=a⋅1v
Now substitute dvdx back into the equation for the slope:
d(KE)dx=mv(a⋅1v)=ma
The slope of the kinetic energy displacement curve is:
d(KE)dx=ma
This shows that the slope is directly proportional to the acceleration a of the particle.
The slope of the kinetic energy displacement curve of a particle in motion is directly proportional to the acceleration of the particle.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MATCH THE COLUMNS
7 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
A particle moves in a straight line with acceleration proportional to x2, ( Where x is displacement). The gain of kinetic energy for any displacement is proportional to
View SolutionAssertion : The graph of potential energy and kinetic energy of a particle in SHM with respect to position is a parabola.
Reason : Potential energy and kinetic energy do not vary linearly with position.
View Solution
Knowledge Check
The kinetic energy and potential energy of a particle executing simple harmonic motion will be equal when displacement (amplitude = a) is
Aa/2Ba√2Ca/√2Da√2/3
A particle moves in a straight line with retardation proportional to its displacement. Its loss in kinetic energy for any displacement x is proportional to
View SolutionA particle starts simple harmonic motion from the mean position. Its amplitude is a and total energy E . At one instant its kinetic energy is 3E/4 . Its displacement at that instant is
View SolutionThe phase difference between displacement and acceleration of particle in a simple harmonic motion is
View SolutionA particle moves along a straight line with a variable acceleration given in the acceleration - displacement (a−S) curve as shown in the figure. Determine the velocity (in ms−1) of the particle after it has travelled a distance of 30 m. The initial velocity is 10 ms−1.
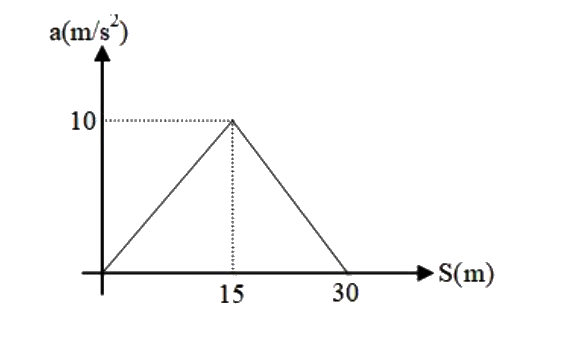
View SolutionAs given in the acceleration displacement (a-S) curve the particle moves in the rectilinear motion. Determine the velocity after the particle has traveled 20 m if the initial velocity is 10 m/s.
View SolutionThe displacement x of a particle in a straight line motion is given by x=1−t−t2. The correct representation of the motion is
View SolutionIn position A kinetic energy of a particle is 60 J and potential energy is -20 J. In position B, kinetic energy is 100 J and potential energy is 40 J. Then, in moving the particle from A to B
View Solution
