The total energy of rolling of mass 'm' and radius 'R' is
3/2mv2
1/2mv2
mv2
5/2mv2
The correct Answer is:C
E=12mv2[1+K2/R2]
=12mv2[1+1]=mv2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The total energy of rolling ring of mass m and radius R is
A3/2mv2B1/2mv2Cmv2D5/2mv2A body is mass m is rotating in a vertical circle of radius 'r' with critical speed. The difference in its K.E at the top and at the bottom is
A92 mgrB6 mgrC4 mgrD2 mgrA half section of thin circular pipe of mass m and radius 'r' is released from rest in the position shown. Pipe rolls without sliding. The change in potential energy of pipe after it has rolled through 90∘ :
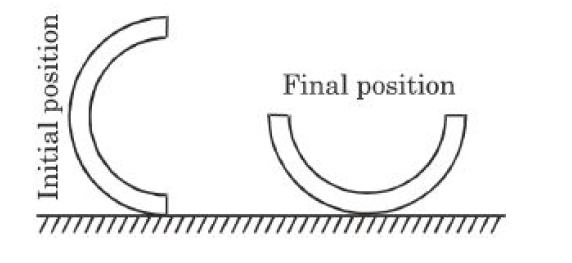 A4mgrπB2mgrπCmgrD√2mgr
A4mgrπB2mgrπCmgrD√2mgr
A satellite of mass m is Launched from the earth's surface in a circular orbit. If M and R denote the mas and radius of the earth respectively, then the total energy of the satellite at an altitude 2R is
View SolutionThe potential energy of a satellite of mass m revolving at height R above the surface of the earth where R= radius of earth is
View SolutionWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 3R ?
View SolutionWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
View SolutionWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
View Solution
