The ultimate resolving power $λ / δ λ$ of the spectrograph's trihedral prism is determined by diffraction of light at the prism edges (as in the case of a slit). When the prism is oriented to the least deviation angle in accordance with Rayleigh's criterion,
$λ / δ λ = b | d n / d λ |,$
where $b$ is the width of the prism's base (Fig.) and $d n / d λ$ is the dispersion of its material. Derive this formula.
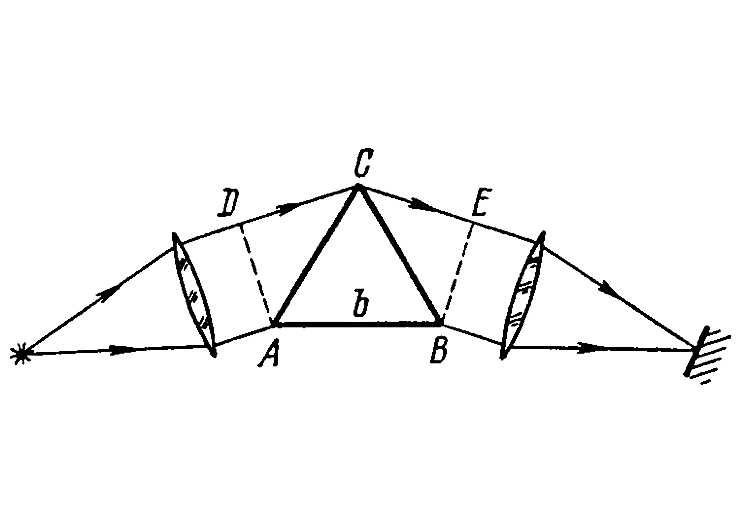
$The ultimate resolving power lambda//delta lambda of the spectrograph's trihedral prism is determined by diffraction of light at the prism edges (as in the case of a slit). When the prism is oriented to the least deviation angle in accordance with Rayleigh's criterion, lambda//delta lambda = b|dn//d lambda|, where b is the width of the prism's base (Fig.) and dn//d lambda is the dispersion of its material. Derive this formula.$

Video Solution

Text Solution

Verified by Experts

Suppose the incident light consists of two wavelength $λ$ and $λ + δ λ$ which are just resolved by the prism. Then by Rayleight's criterion, the maximum of the line of wavelength $λ$ must coincide with the first minimum of the line of wavelength $λ + δ λ$ . Let us write both conditions in terms of the opticak path diferences for the exterme rays:
For the light of wavelength $λ$
$b n - (D C + C E) = 0$
For the light of wavelength $λ + δ λ$
$b (m + δ n) - (D C + C E) = λ + δ λ$
beacuse the path difference bwteen extreme rays equal $λ$ for the first minimum in a single slit diffraction (from the fromula $a sin θ = λ$ ).
Hence $b d \leq t a n \approx λ$
and $R = \frac{λ}{δ λ} = B ∣ ∣ ∣ \frac{δ n}{δ λ} ∣ ∣ ∣ = b ∣ ∣ ∣ \frac{d n}{d λ} ∣ ∣ ∣$

If $A = {λ, (λ, μ)}$ , then the power set of A is
A ${ϕ, {ϕ}, {λ}, {λ, μ}}$
B ${ϕ, {λ}, {{λ, μ}}, {λ, {λ, μ}}}$
C ${ϕ, {λ}, {λ, μ}, {λ, {λ, μ}}}$
D ${{λ}, {λ, μ}, {λ, {λ, μ}}}$
A trihedral prism with refracting angle $60^{\circ}$ deviates a light ray by $30^{\circ}$ . The refractive index of the material of prism
Amay be equal to $\sqrt{2}$
Bcan not be greater than $\sqrt{2}$
Ccan not be less than $\sqrt{2}$
Dnone of these
In a single slit diffraction of light of wavelength $λ$ by a slit of width e, the size of the central maximum on a screen at a distance b is
A(a) $2 b λ + e$
B(b) $\frac{2 b λ}{e}$
C(c) $\frac{2 b λ}{e} = e$
D(d) $\frac{2 b λ}{e} - e$