The velocity of a body moving in a straight line is increased by applying a constant force F, for some distance in the direction of the motion. Prove that the increase in the kinetic energy of the body is equal to the work done by the force on the body.
To prove that the increase in kinetic energy of a body is equal to the work done by a constant force F applied over a distance, we can follow these steps:
Step 1: Define Initial and Final Velocities
Let the initial velocity of the body be u and the final velocity after applying the force be v.
Step 2: Write the Expression for Kinetic Energy
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
where m is the mass of the body.
- Initial kinetic energy when the velocity is u:
KEinitial=12mu2
- Final kinetic energy when the velocity is v:
KEfinal=12mv2
Step 3: Calculate the Change in Kinetic Energy
The increase in kinetic energy (ΔKE) is given by:
ΔKE=KEfinal−KEinitial=12mv2−12mu2
This simplifies to:
ΔKE=12m(v2−u2)
Step 4: Relate Force, Mass, and Acceleration
According to Newton's second law, the force F applied to the body is equal to the mass m multiplied by the acceleration a:
F=ma
Step 5: Use the Kinematic Equation
From the equations of motion, we know:
v2−u2=2as
where s is the distance over which the force is applied.
Step 6: Substitute for Acceleration
Rearranging the kinematic equation gives us:
a=v2−u22s
Step 7: Calculate Work Done by the Force
The work done W by the force when it acts over a distance s is given by:
W=F⋅s
Substituting F=ma gives:
W=ma⋅s
Now substituting a from the previous step:
W=m(v2−u22s)s
This simplifies to:
W=12m(v2−u2)
Step 8: Equate Work Done and Change in Kinetic Energy
From Steps 3 and 7, we have:
W=ΔKE
Thus, we can conclude that the increase in kinetic energy of the body is equal to the work done by the force on the body:
ΔKE=W
Conclusion
Therefore, we have proved that the increase in kinetic energy of the body is equal to the work done by the force on the body.
---
Let the initial velocity of the body be u and the final velocity after applying the force be v.
The kinetic energy (KE) of an object is given by the formula:
KE=12mv2
where m is the mass of the body.
KEinitial=12mu2
KEfinal=12mv2
The increase in kinetic energy (ΔKE) is given by:
ΔKE=KEfinal−KEinitial=12mv2−12mu2
This simplifies to:
ΔKE=12m(v2−u2)
According to Newton's second law, the force F applied to the body is equal to the mass m multiplied by the acceleration a:
F=ma
From the equations of motion, we know:
v2−u2=2as
where s is the distance over which the force is applied.
Rearranging the kinematic equation gives us:
a=v2−u22s
The work done W by the force when it acts over a distance s is given by:
W=F⋅s
Substituting F=ma gives:
W=ma⋅s
Now substituting a from the previous step:
W=m(v2−u22s)s
This simplifies to:
W=12m(v2−u2)
From Steps 3 and 7, we have:
W=ΔKE
Thus, we can conclude that the increase in kinetic energy of the body is equal to the work done by the force on the body:
ΔKE=W
Therefore, we have proved that the increase in kinetic energy of the body is equal to the work done by the force on the body.
Topper's Solved these Questions
- WORK AND ENERGYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - LONG ANSWER TYPE QUESTIONS
8 Videos
- WORK AND ENERGYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - LONG ANSWER TYPE QUESTIONS
8 Videos
- SOUNDBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SOUNDEXERCISE - Long Answer Type Questions
3 Videos
Similar Questions
Knowledge Check
When the kinetic energy of a body is increased by there its momentum is increased by:
A9 timesB3 timesC√2 timesD√3 times
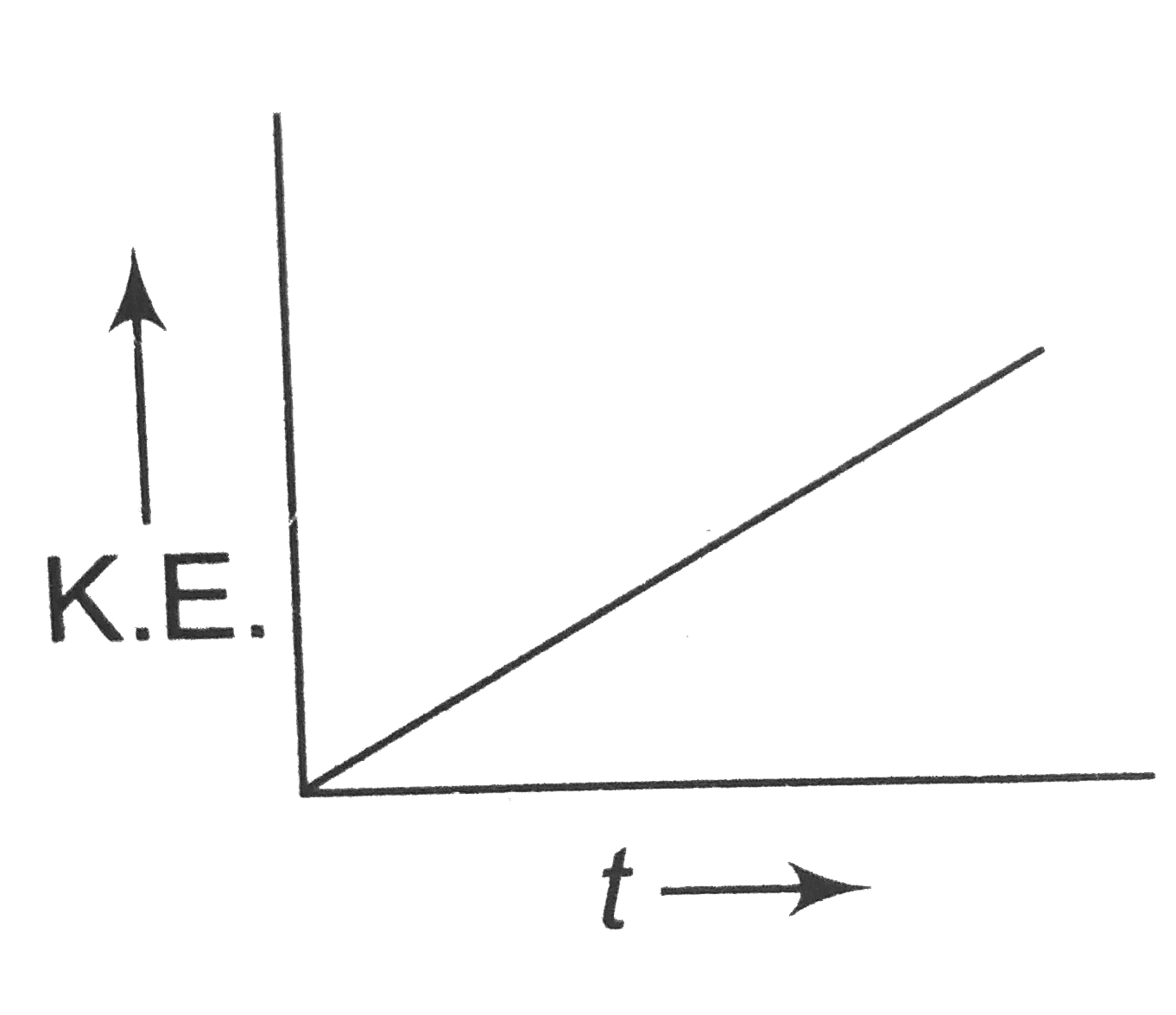
The kinetic energy of a body moving along a straight line varies with time as shown in figure. The force acting on the body:
View SolutionIf the force acting on a body is inversely proportional to its speed, the kinetic energy of the body is
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
View SolutionA body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
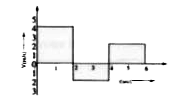
View SolutionThe velocity time graph of a body moving in a straight line is shown in the figure. The displacement and distance travelled by the body in 6 sec are respectively.
View SolutionA body is moved in a direction opposite to the direction of force acting on it.State whether the work is done by the work in done against the force.
View Solution