A body is moved along a straight line by a machine delivering constant power . The distance moved by the body is time t is proptional to
(a) t1/2
(b) t3/4
(c) t3/2
(d) t2
The correct Answer is:C
To solve the problem, we need to analyze the relationship between power, force, velocity, and displacement when a body is moved by a machine delivering constant power.
1. Understand the relationship between power, force, and velocity:
P=F⋅v
where P is power, F is force, and v is velocity.
2. Express force in terms of mass and acceleration:
F=m⋅a
where m is mass and a is acceleration.
3. Relate acceleration to velocity and displacement:
Acceleration can be expressed as:
a=dvdt=d2xdt2
where x is displacement.
4. Substitute acceleration into the power equation:
Replacing F in the power equation gives:
P=m⋅a⋅v
Substituting a with dvdt and v with dxdt:
P=m⋅d2xdt2⋅dxdt
5. Rearranging the equation:
This can be rearranged to:
P=m⋅dxdt⋅d2xdt2
or
P=m⋅v⋅a
6. Express velocity in terms of displacement and time:
Velocity can be expressed as:
v=dxdt
7. Substituting velocity into the power equation:
Thus, we can write:
P=m⋅dxdt⋅d2xdt2=m⋅dxdt⋅ddt(dxdt)
8. Using the relationship between displacement and time:
We can express v as dxdt and substitute back into the power equation:
P=m⋅dxdt⋅d2xdt2=m⋅dxdt⋅ddt(dxdt)
9. Integrating to find displacement:
By integrating and rearranging, we find:
P=m⋅x2t3
Rearranging gives:
x2=P⋅t3m
10. Taking the square root to find displacement:
x=√P⋅t3m=√P√m⋅t3/2
11. Conclusion:
Thus, we find that the displacement x is proportional to t3/2:
x∝t3/2
Final Answer:
The distance moved by the body in time t is proportional to t3/2.
---
P=F⋅v
where P is power, F is force, and v is velocity.
F=m⋅a
where m is mass and a is acceleration.
Acceleration can be expressed as:
a=dvdt=d2xdt2
where x is displacement.
Replacing F in the power equation gives:
P=m⋅a⋅v
Substituting a with dvdt and v with dxdt:
P=m⋅d2xdt2⋅dxdt
This can be rearranged to:
P=m⋅dxdt⋅d2xdt2
or
P=m⋅v⋅a
Velocity can be expressed as:
v=dxdt
Thus, we can write:
P=m⋅dxdt⋅d2xdt2=m⋅dxdt⋅ddt(dxdt)
We can express v as dxdt and substitute back into the power equation:
P=m⋅dxdt⋅d2xdt2=m⋅dxdt⋅ddt(dxdt)
By integrating and rearranging, we find:
P=m⋅x2t3
Rearranging gives:
x2=P⋅t3m
x=√P⋅t3m=√P√m⋅t3/2
Thus, we find that the displacement x is proportional to t3/2:
x∝t3/2
The distance moved by the body in time t is proportional to t3/2.
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives(multiple Correct)
3 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (assertion-reasoning)
1 Video
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Integer
14 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A body is moved from rest along a straight line by a machine delivering constant power. The ratio of displacement and velocity (s/v) varies with time t as
View SolutionA body is moved from rest along a straight line by a machine delivering constant power. The ratio of displacement and velocity (s/v) varies with time t as
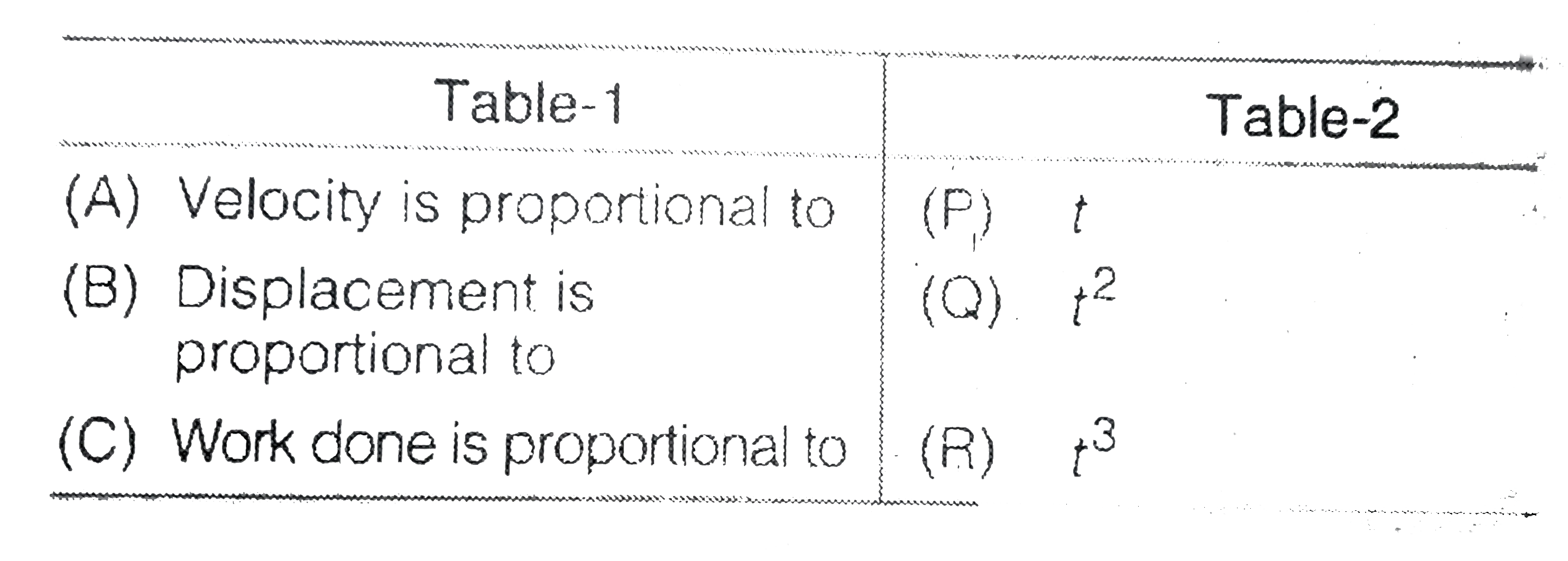
View SolutionA body is moved along a straight line by a machine delivering a power proportional to time (P∝t). Then, match the following.
View SolutionA machine is delivering constant power to drive a body along a straight line. What is the relation between the distance travelled by the body against time ?
View SolutionA motor drives a body along a straight line with a constant force. The power P developed by the motor must vary with time t as
View SolutionThe initial velocity of a body moving along a straight lines is 7m/s. It has a uniform acceleration of 4m/s2 the distance covered by the body in the 5th second of its motion is-
View SolutionThe velocity of a body moving in a straight line is increased by applying a constant force F, for some distance in the direction of the motion. Prove that the increase in the kinetic energy of the body is equal to the work done by the force on the body.
View SolutionA body of mass 2 kg is driven by an engine delivering a constant power of 1 J /s. The body starts from rest and moves in a straight line. After 9 seconds, the body has moved a distance ( in m) ……….. .
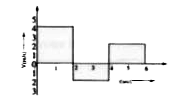
View SolutionThe velocity time graph of a body moving in a straight line is shown in the figure. The displacement and distance travelled by the body in 6 sec are respectively.
View SolutionA machine delivers power to a body which is proportional to velocity of the body. If the body starts with a velocity which is almost negligible, then the distance covered by the body is proportional to
View Solution
