There are 7 identical capacitors. The equivalent capacitance when they are connected in series is C. The equivalent capacitance when they are connected in parallel is
C/49
C/7
7C
49 C
The correct Answer is:D
To solve the problem step by step, we will first determine the capacitance of each capacitor and then find the equivalent capacitance when they are connected in parallel.
Step 1: Determine the capacitance of each capacitor
Let the capacitance of each identical capacitor be C0. When the 7 capacitors are connected in series, the formula for equivalent capacitance C is given by:
1C=1C0+1C0+1C0+1C0+1C0+1C0+1C0
This simplifies to:
1C=7C0
Step 2: Solve for C0
From the equation above, we can rearrange it to find C0:
C0=7C
Step 3: Calculate the equivalent capacitance when connected in parallel
When the same 7 capacitors are connected in parallel, the equivalent capacitance Ceq is simply the sum of the individual capacitances:
Ceq=C0+C0+C0+C0+C0+C0+C0
This simplifies to:
Ceq=7C0
Step 4: Substitute the value of C0
Now, substitute the value of C0 that we found earlier:
Ceq=7(7C)=49C
Final Answer
Thus, the equivalent capacitance when the 7 identical capacitors are connected in parallel is:
Ceq=49C
---
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (B) Chapter exercises
17 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - Check point 2.5
20 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
Similar Questions
The equivalent capacitance between A and B is

View SolutionThe equivalent capacitance between A and B is
View SolutionFour capacitors of equal capacitance have an equivalent capacitance C1 when connected in series and an equivalent capacitance C2 when connected in parallel. The ratio C1C2 is
View SolutionThree capacitors each of capacitance C are connected in series. Their equivalent capacitance is Cs. The same three capacitors are now connected in parallel. Their equivalent capacitance becomes Cp. Find the ratio (CpCs)
(Working must be shown).
View SolutionSix identical capacitors each of capacitance 2μF are connected as shown below. The equivalent capacitance between the points A and B is
View SolutionTwo capacitors with capacitances C1andC2 are connected in series. Find the resultant capacitance.
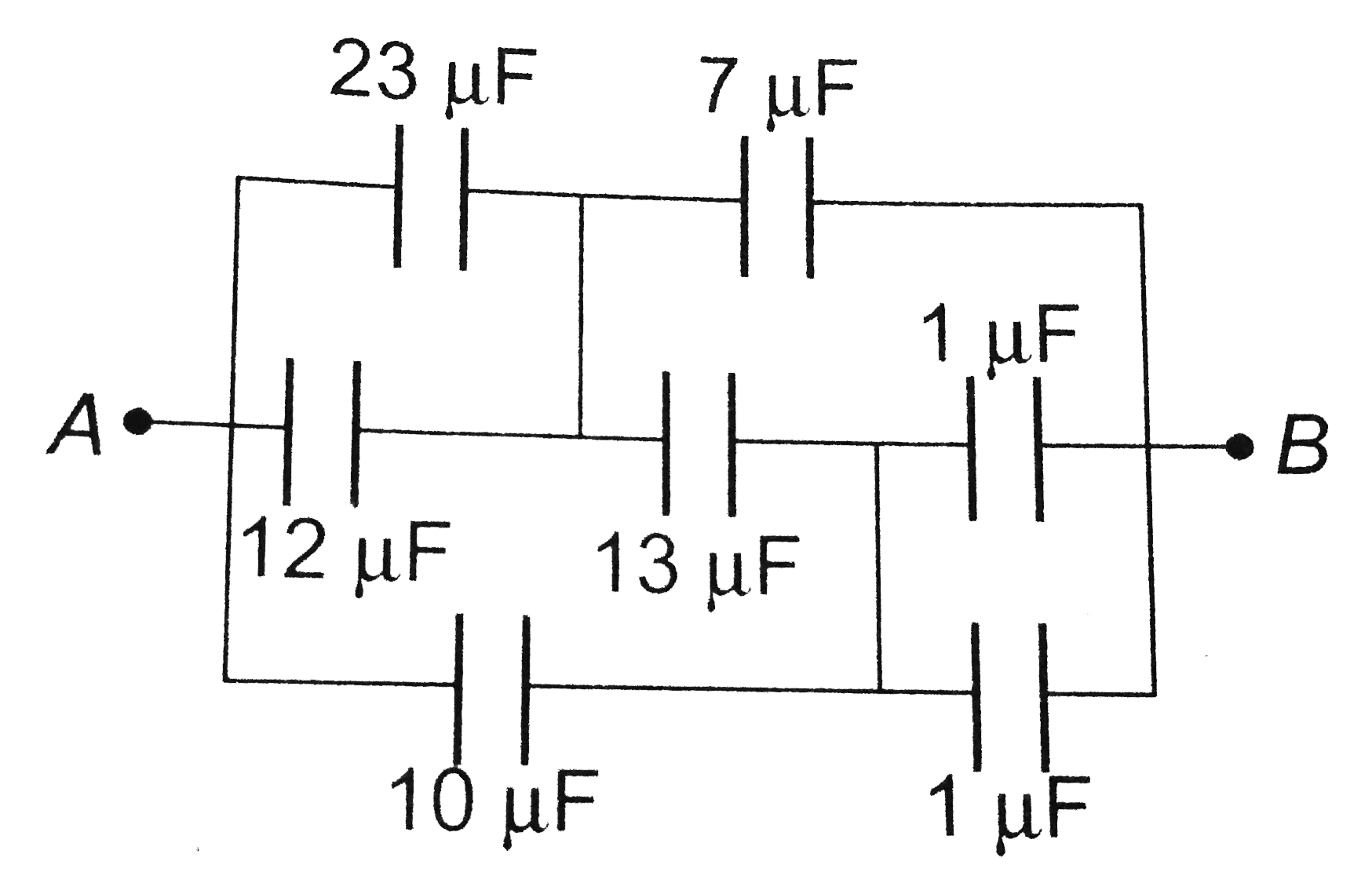
View SolutionFind the equivalent capacitance between C and B.
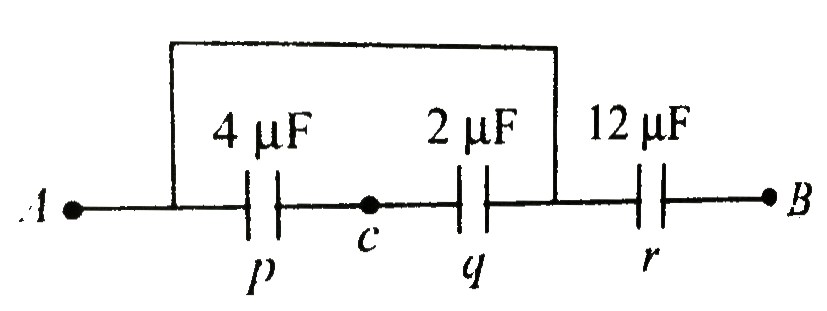
View SolutionEquivalent capacitance between A and B is
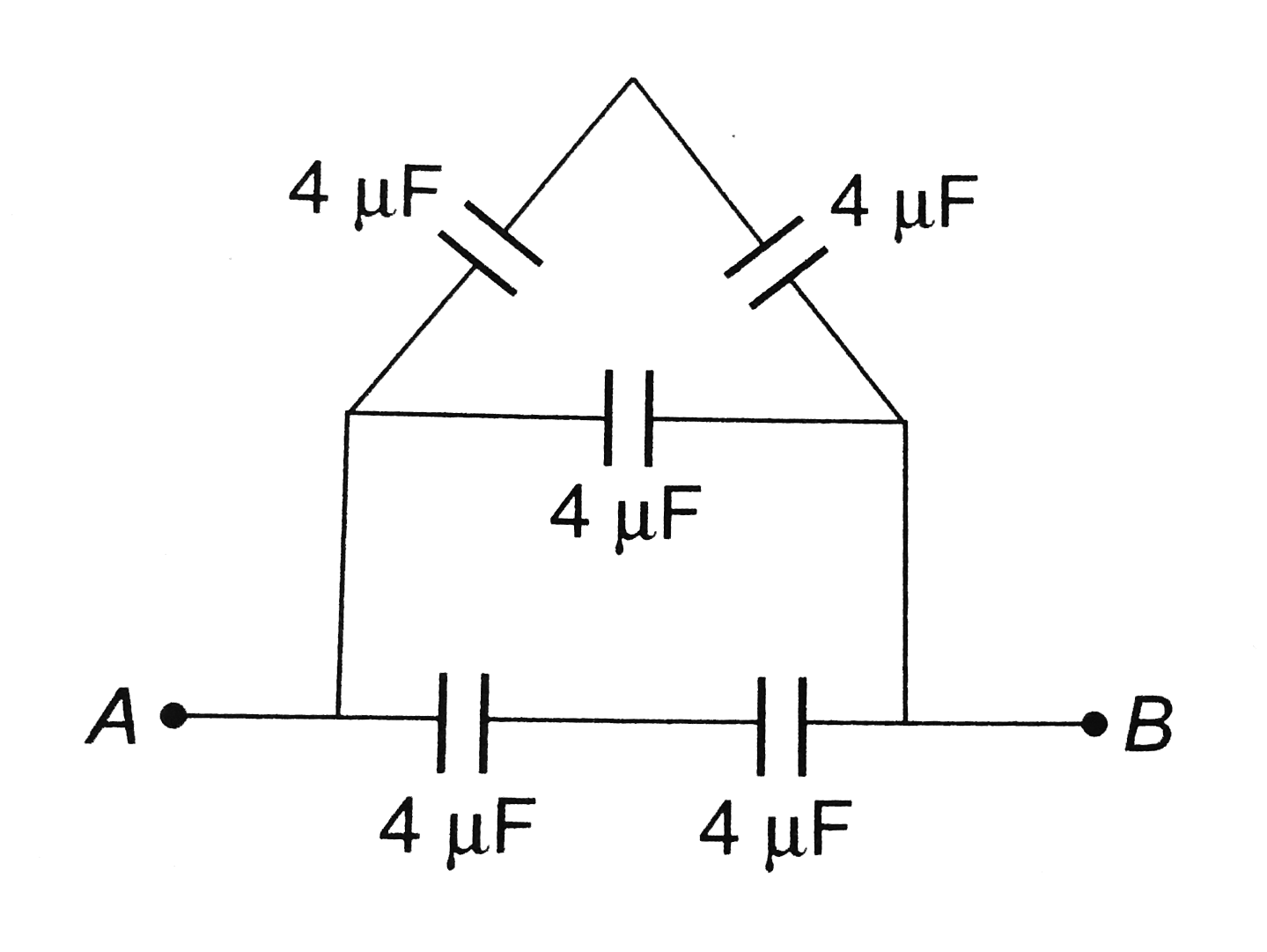
View SolutionThe equivalent capacitance between A and B will be
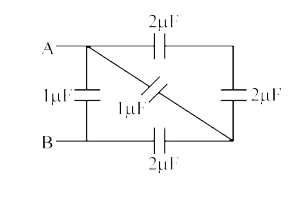
View SolutionEquivalent capacitance between A and B is:
View Solution

