There are two cavities (Fig.) with small holes of equal diameters $d = 1.0 c m$ and perfectly reflecting outer surfaces. The distance between the holes is $l = 10 c m$ . A constant temperature $T_{1} = 1700 K$ is maintained in cavity $1$ . Calculate the steady-state temperature inside cavity $2$ .

Text Solution

Verified by Experts

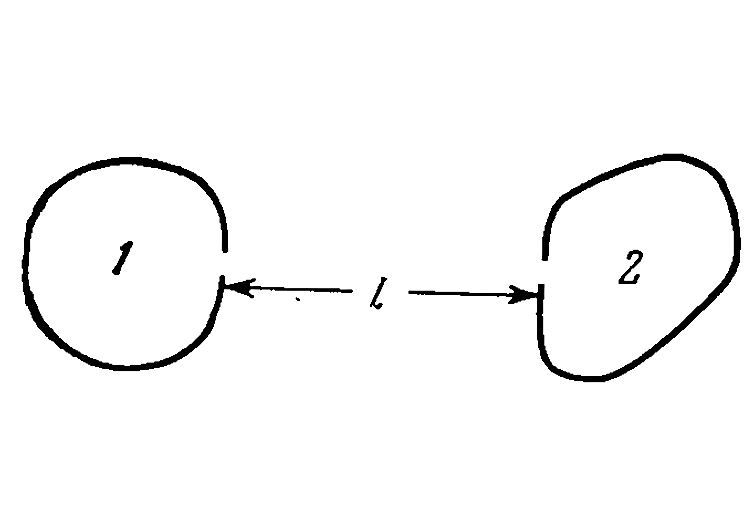
Taking account of cosine low of emission we write for the enegry radiated per second by the hole in cavity $# 1$ as
$d i (Ω) = A cos θ d Ω$
where $A$ is an consatnt, $d Ω$ is an element of solid angle around some direction defined by the symbol $Ω$ . Integrating over the whole forwed hemisphere we get
$I = A π / 2 \int 0 cos θ 2 π sin θ d θ = π 4$

We find $A$ by equating this to the quantity $σ T_{1}^{4} . \frac{π d^{2}}{4} σ$ is stefan-Boltzman constant and $d$ is the diameter of the hole.
Then $A = \frac{1}{4} σ d^{2} T_{1}^{4}$
Now energy reaching $2$ form $1$ is $(cos θ = 1)$
$\frac{1}{4} σ d^{2} T_{1}^{4} . Δ Ω$
where $Δ Ω = \frac{(π d^{2} / 4)}{l^{2}}$ is the solid angle substended by the hole of $2$ at $1$ . {We are assuming $d < < l$ so $Δ Ω =$ area of hole $/ {(disatnce)}^{2}}$ .
This must equal $σ T_{2}^{4} π d^{2} / 4$
which is the energy emitted by $2$ . Thus equating
$\frac{1}{4} σ d^{2} T_{1}^{4} \frac{π d^{2}}{4 l^{2}} = σ T_{2}^{4} \frac{π d^{2}}{4}$
or $T_{2} = T_{1} \sqrt{\frac{d}{2 l}}$
Substituting we get $T_{2} = 0.380 k K = 380 K$ .

The plane wall of an oven is of thickness L = 0.05 m and is exposed to the surrounding at 300 K. The outer surface of the oven exposed to the surrounding is at temperature 320 K in steady state. The energy radiated (In $W / m^{2}$ ) by outer surface is proportional to the temperature difference between the surface and the surrounding, with proportionality constant $20 W / m^{2} K$ . What is the temperature of inner surface of the oven in steady state if the wall has a thermal conductivity of K = 0.5 W/mK
A400 K
B340 K
C380 K
D360 K