There is a ring or mass m and radius R is pivoted at a point O on its periphery. It is free to rotate about an axis perpendicular to its plane. What is the period of ring?
T=2π((Rg))
T=2π√(2Rg)
T=π√(2Rg)
T=2π√(3Rg)
The correct Answer is:B
d= R and l = 2mR2
∵ T=2π√[lmgd]T=2π√[2mR2mgR]
⇒ T=2π√[2Rg]

Topper's Solved these Questions
Similar Questions
Knowledge Check
A disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
A2π√RgB2π√2RgC2π√2π3gD2π√3R2gAn arm making an angle of 120∘ at the center of ring of mass m and radius r is cut from the ring. The arc is made to rotate about z-axis perpendicular to its plane and passing through the center of the ring. The moment of inertia of the arc about the z-axis is
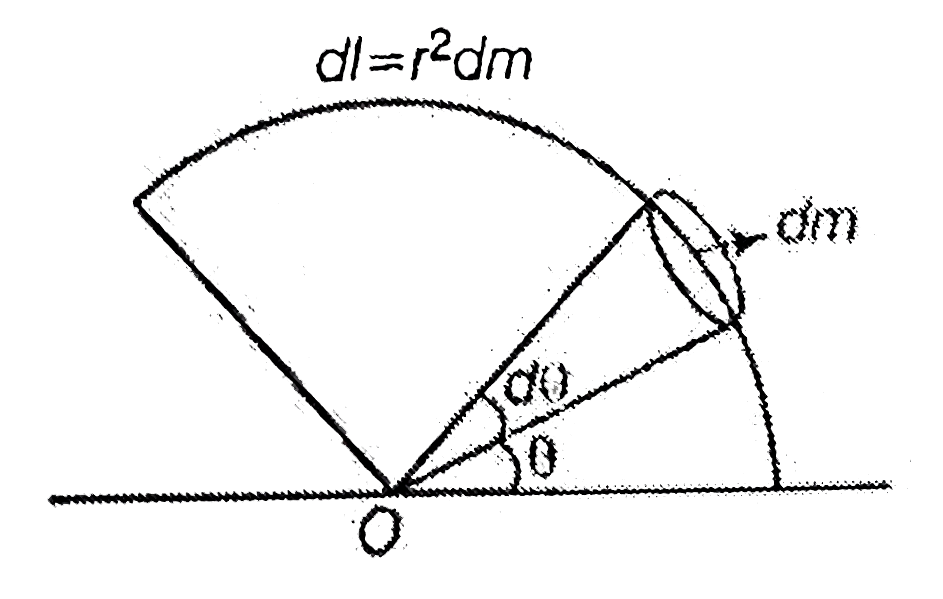 Amr2Bmr23Cmr22D(mr24
Amr2Bmr23Cmr22D(mr24The M.I. of a ring of mass M and radius R about a tangential axis perpendicular to its plane is :
A2MR2BMR2C3/2MR2D3/4MR2
Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is I. What is the moment of inertia about its diameter ?
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is I. What is the moment of inertia about its diameter?
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View SolutionA ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View Solution
