The M.I. of a ring of mass M and radius R about a tangential axis perpendicular to its plane is :
2MR2
MR2
3/2MR2
3/4MR2
The correct Answer is:A
To find the moment of inertia (M.I.) of a ring of mass M and radius R about a tangential axis perpendicular to its plane, we can use the Parallel Axis Theorem. Here’s a step-by-step solution:
Step 1: Understand the Geometry
We have a ring with mass M and radius R. The axis we are interested in is tangential to the ring and perpendicular to its plane.
Step 2: Identify the Moment of Inertia about the Center
The moment of inertia of a ring about an axis that passes through its center and is perpendicular to its plane is given by:
Icm=MR2
Step 3: Apply the Parallel Axis Theorem
The Parallel Axis Theorem states that if you want to find the moment of inertia about an axis that is parallel to an axis through the center of mass, you can use the formula:
I=Icm+Md2
where d is the distance between the two axes.
Step 4: Determine the Distance d
In this case, the distance d between the center of the ring and the tangential axis is equal to the radius R of the ring:
d=R
Step 5: Substitute into the Formula
Now substituting the values into the Parallel Axis Theorem:
I=Icm+Md2=MR2+MR2
I=MR2+MR2=2MR2
Conclusion
Thus, the moment of inertia of the ring about the tangential axis perpendicular to its plane is:
2MR2
We have a ring with mass M and radius R. The axis we are interested in is tangential to the ring and perpendicular to its plane.
The moment of inertia of a ring about an axis that passes through its center and is perpendicular to its plane is given by:
Icm=MR2
The Parallel Axis Theorem states that if you want to find the moment of inertia about an axis that is parallel to an axis through the center of mass, you can use the formula:
I=Icm+Md2
where d is the distance between the two axes.
In this case, the distance d between the center of the ring and the tangential axis is equal to the radius R of the ring:
d=R
Now substituting the values into the Parallel Axis Theorem:
I=Icm+Md2=MR2+MR2
I=MR2+MR2=2MR2
Thus, the moment of inertia of the ring about the tangential axis perpendicular to its plane is:
2MR2
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ENABLE
50 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EFFICIENT
50 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - IN - CHAPTER EXERCISE - B
10 Videos
Similar Questions
The M.I. of a thin ring of mass M and radius R about an axis through the diameter in its plane will be:
View SolutionThe ratio of the radii of gyration of a circular disc and a circular ring of the same radii about a tangential axis perpendicular to plane of disc or ring is
View SolutionThe moment of inertia of a disc of mass M and radius R about a tangent to its rim in its plane is
View SolutionA thin circular ring of mass m and radius R is rotating about its axis perpendicular to the plane of the ring with a constant angular velocity ω. Two point particleseach of mass M are attached gently to the opposite end of a diameter of the ring. The ring now rotates, with an angular velocityω2. Then the ratiomM is
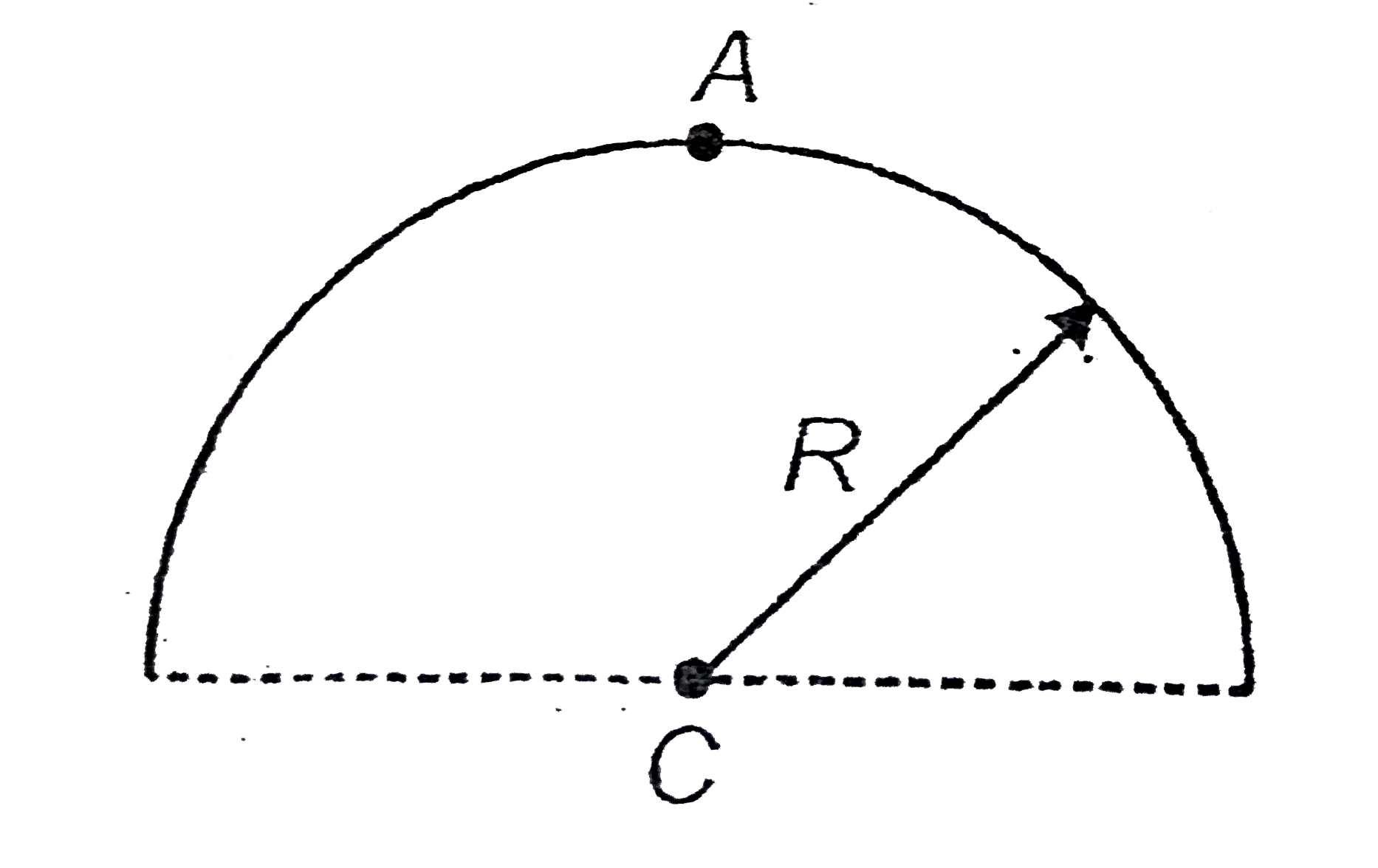
View SolutionMoment of inertia of a half ring of mass m and radius R about an axis passing through point A perpendicular to the plane of the paper is IA. If IC is the moment of inertia of the ring about an axis perpendicular to the plane of paper and passing through point C, then
View SolutionFor a uniform ring of mass M and radius R at its centre
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionA particle of mass m is placed at a distance R from centre of a uniformly dense thin ring of mass m rand radius R on a axis passing through its centre and perpendicular to its plane. Find the speed of the particle when it reaches at the centre fo the ring due to their mutual gravitation force.
View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
View SolutionThe moment of inertia of a uniform semicircularwire of mass M and radius r about a line perpendicular to the plane of the wire through the centre is :
View Solution