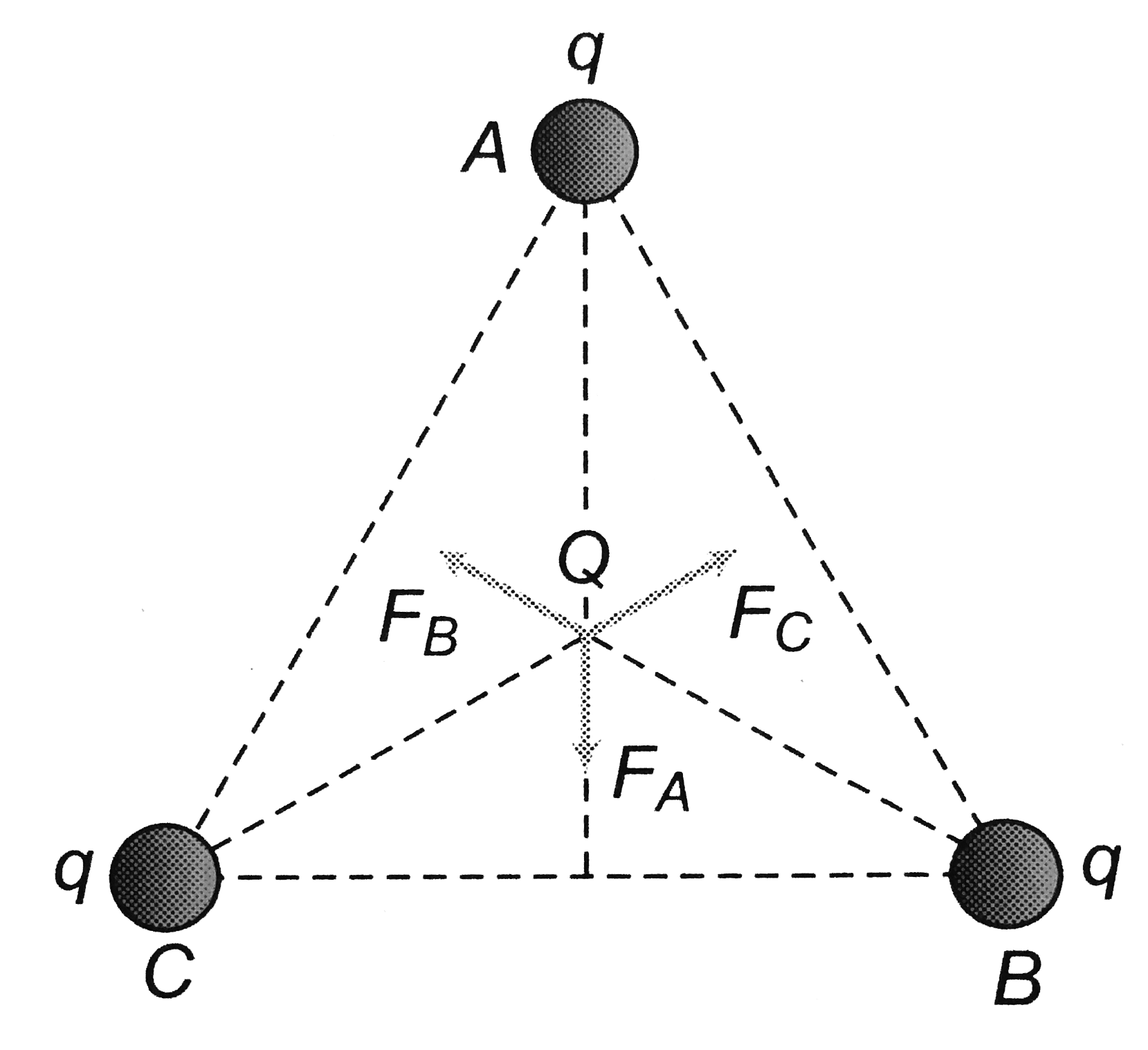
Three charges, each of value q, are placed at the comers of an equilateral triangle. A fourth charge Q is placed at the centre of the triangle. If the value of Q for which charges remain stationary is (−q√n), then find n.
The correct Answer is:3
To solve the problem, we need to find the value of n such that the charge Q placed at the center of an equilateral triangle formed by three charges q remains stationary.
1. Understanding the Configuration:
- We have three charges q located at the corners of an equilateral triangle with side length a.
- A fourth charge Q is placed at the center of the triangle.
2. Finding the Distance from the Center:
- The distance from the center of the triangle to any vertex (corner) is given by a√3.
3. Calculating the Force on Charge Q:
- Each charge q exerts a force on charge Q. The force F between two point charges is given by Coulomb's law:
F=k|qQ|r2
- Here, r=a√3, so the force due to one charge q on Q is:
F=kqQ(a√3)2=kqQ⋅3a2=3kqQa2
4. Resultant Force Calculation:
- Since there are three identical charges q at the corners, the forces will have a symmetry. The net force Fnet on charge Q due to the three charges can be calculated.
- The angle between any two forces due to the charges is 60∘. Therefore, the resultant force can be calculated using the formula for the resultant of two forces:
Fnet=√F2+F2+2F⋅F⋅cos(60∘)=√F2+F2+F2=√3F2=F√3
- Hence, the net force acting on Q due to the three charges is:
Fnet=3⋅3kqQa2⋅12=9kqQ2a2
5. Condition for Equilibrium:
- For charge Q to remain stationary, the net force acting on it must be zero. Therefore, we set the net force equal to zero:
Fnet=0
- This implies that the force due to the charge Q must balance out the forces from the three charges q:
9kqQ2a2=0
6. Substituting the Value of Q:
- Given that Q=−q√n, we substitute this into the equilibrium condition:
9kq(−q√n)2a2=0
- This leads to:
−9kq22a2√n=0
- Since k, q, a are not zero, we conclude that:
√n=9⟹n=81
Final Answer:
Thus, the value of n is 81.
- We have three charges q located at the corners of an equilateral triangle with side length a.
- A fourth charge Q is placed at the center of the triangle.
- The distance from the center of the triangle to any vertex (corner) is given by a√3.
- Each charge q exerts a force on charge Q. The force F between two point charges is given by Coulomb's law:
F=k|qQ|r2
- Here, r=a√3, so the force due to one charge q on Q is:
F=kqQ(a√3)2=kqQ⋅3a2=3kqQa2
- Since there are three identical charges q at the corners, the forces will have a symmetry. The net force Fnet on charge Q due to the three charges can be calculated.
- The angle between any two forces due to the charges is 60∘. Therefore, the resultant force can be calculated using the formula for the resultant of two forces:
Fnet=√F2+F2+2F⋅F⋅cos(60∘)=√F2+F2+F2=√3F2=F√3
- Hence, the net force acting on Q due to the three charges is:
Fnet=3⋅3kqQa2⋅12=9kqQ2a2
- For charge Q to remain stationary, the net force acting on it must be zero. Therefore, we set the net force equal to zero:
Fnet=0
- This implies that the force due to the charge Q must balance out the forces from the three charges q:
9kqQ2a2=0
- Given that Q=−q√n, we substitute this into the equilibrium condition:
9kq(−q√n)2a2=0
- This leads to:
−9kq22a2√n=0
- Since k, q, a are not zero, we conclude that:
√n=9⟹n=81
Thus, the value of n is 81.
Topper's Solved these Questions
Similar Questions
Three charges, each of value q, are placed at the vartices of an equilateral triangle. A fourth charge Q is placed at the centre of the triangle. (i) If Q=−q, will the charge at the vertices move towards the centre of fly away from it? (ii) For what value of Q will the charges remain stationary?
View SolutionThree charges each of value q are placed at the corners of an equilateral triangle. A fourth charge Q is placed at the center of the triangle.
a. Find the net force on charge q.
b. If Q=−q, will the charges at the corners move toward the center or fly away from it ?
c. For what value of Q and O will the charges remain stationary ?
d. In situation (c), how much work is done in removing the charges to infinity ?
View Solution
Knowledge Check
Three charges each of value q are placed at the corners of an equilateral triangle. A fourth charge Q is placed at the centre of the triangle. If Q=−q:
Athe charges will move towards the centreBthe charges will move away from the centreCthe charges will remain stationaryDthe charges may move in any directionIn the above problem , the value of Q for which charges will remain stationary is
Aq√3Bq√3C−q√3D−q√3At each corner of an equilateral triangle identical charges are placed. Then at the centre of the triangle
Athe resultant electric intensity is zeroBthe net potential is zeroCboth electric intensity and potential are zeroDneither electric intensity nor potential are zero
Three equal charges, each +q are placed on the corners of an equilatreal triangel . The electric field intensity at the centroid of the triangle is
View SolutionThree charges each of magnitude q are placed at the corners of an equilateral triangle, the electrostatic force on the charge place at the centre is (each side of triangle is L)
View SolutionThree charges 2q,−q,and−q are located at the vertices of an equilateral triangle. At the center of the triangle,
View SolutionThree charges +Q each are placed at the corners A,B and C of an equilateral triangle. At the circumcenre, O the electric field wil be
View SolutionThree charge −q,+q and −q are placed at the cornors of an equilateral triangle of side a The resultant electric froce an a charge +q placed at a the centroid O of the triangle is
View Solution
