Three insects A,B and C are situated at the vertices of an equillateral triangle of side 1. the insect A heads towards B,B towards C,C towards A with constant speeds v such that they always remain at the vertices of an equilateral triangle. Find the (a) time of their meeting (b) equation of path traced by one insect relative to the other.
After a time t, let the distance of spearation between the insects be r. the velocity of approach (component of relative velocity urel=v+vcos60∘=3v2
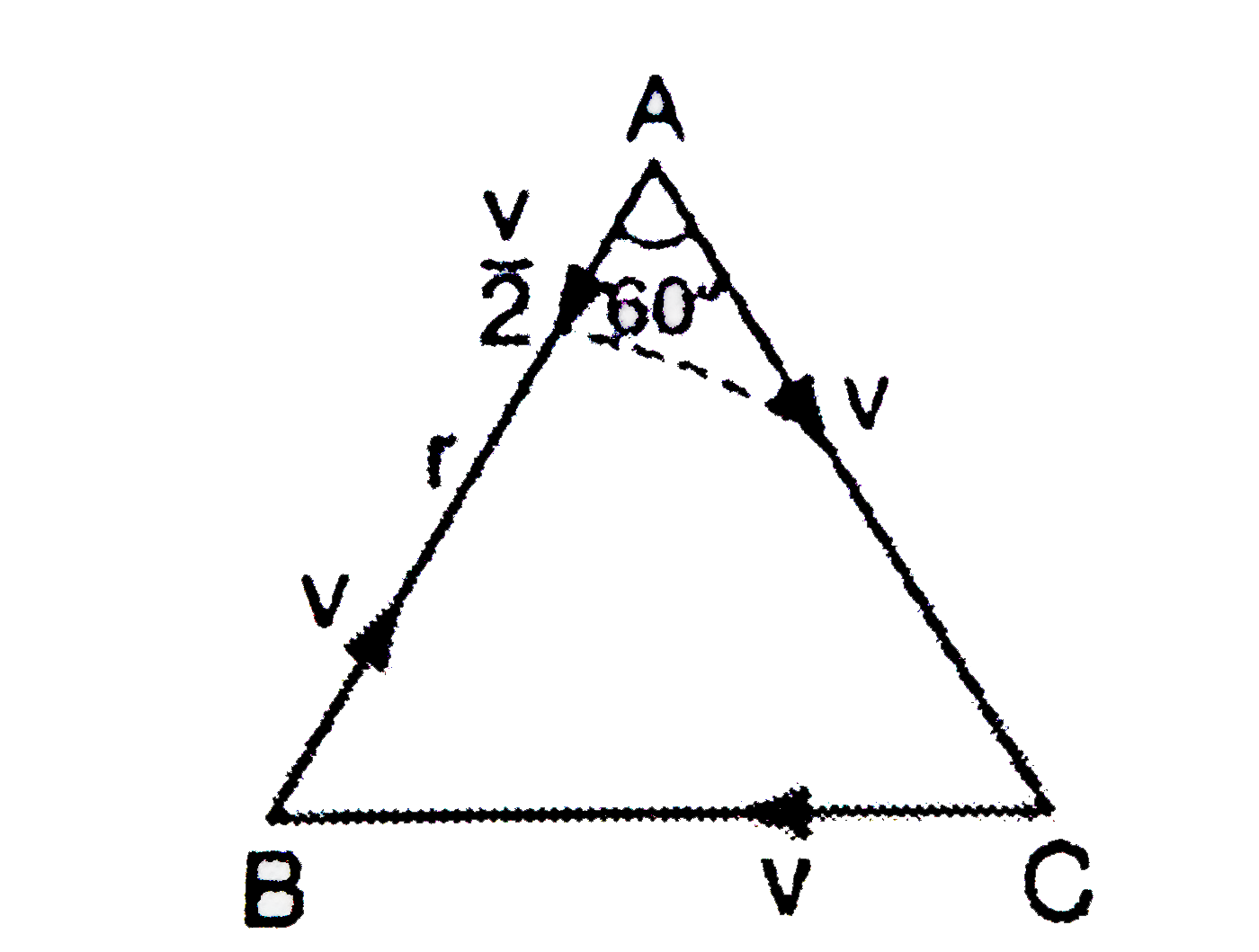
Since, (vrel)∣∣dt=−dr, submitting (vRel)∣∣=3v2, we obtain
3v2dt=−dr ......(i)
As ,r=latt=0 and r=0att=τ (time of meeting ), integrating both sides, we have
3v2∫τ0dt=−∫0ldr
This gives, τ=2l3v
Topper's Solved these Questions
Similar Questions
Three particles A,B,andC are situated at the vertices of an equilateral triangle of side r at t=0. The particle A heads towards B, B towards C, C towards A with constant speeds v. Find the time of their meeting.
View SolutionThree boys A,B and C are situated at the vertices of an equilateral triangle of side d at t=0. Each of the boys moves with constant speed v. A always moves towards C and C towards A, At what time and where they meet each other?
View Solution
Knowledge Check
Three particles A,B and C and situated at the vertices of an equilateral triangle ABC of side of length l at time t=0,Each of the particles move with constant speed u. A always has its velocity along AB,B along BC and C along CA.
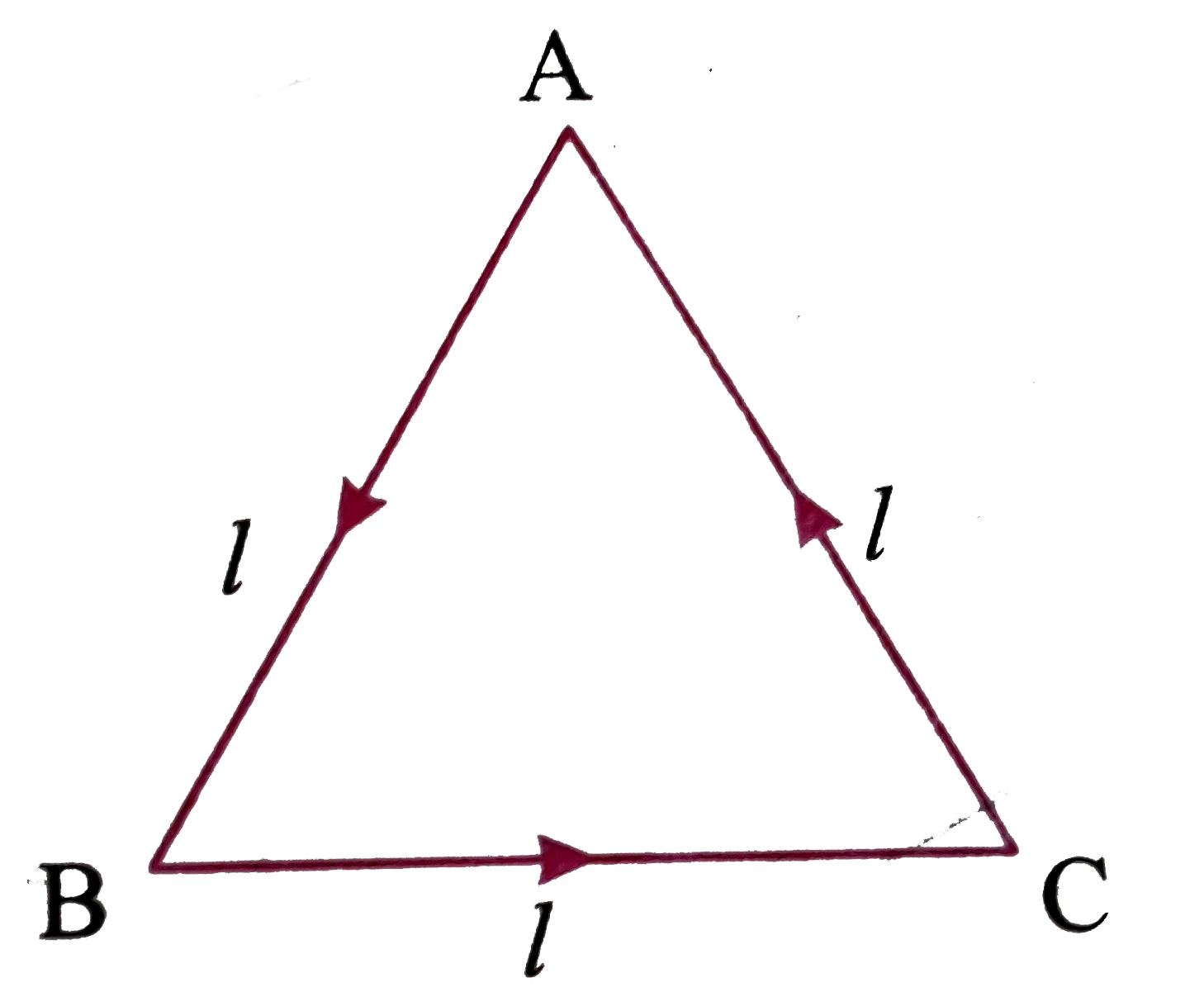 AThe time after which they meet is 2l3uBTotal distance travelled by each particle before they meet is 2l3CAverage velocity during the motion is √3u2DRelative velocity of apporach between any two particles is 3u2
AThe time after which they meet is 2l3uBTotal distance travelled by each particle before they meet is 2l3CAverage velocity during the motion is √3u2DRelative velocity of apporach between any two particles is 3u2Three particles A, B and C are situated at the vertices of an equilateral triangle ABC of side d at time t=0. Each of the particles moves with constant speed v. A always has its velocity along AB, B along BC and C along CA. At what time will the particles meet each other?
At=dvBt=3d2vCt=2d3vDt=0IF →a,→b,→c are the position vectors of the vertices of an equilateral triangle whose orthocentre is at the origin, then
Aa+b+c=0Ba2=b2+c2Ca+b=cDnone of these
If the points A(z),B(−z) and C(z+1) are vertices of an equilateral triangle then
View SolutionShow that the points (a,b,c),(b,c,a),(c,a,b) are the vertices of an equilateral triangle.
View Solutionshow that the points A(1,-1,-5), B(3,1,3) and C(9,1,-3) are the vertices of an equilateral triangle.
View SolutionShow that the points A(1,2),B(1,6), C(1+2√3,4) are the
vertices of an equilateral triangle .
View SolutionIf the points A(z), B(-z) and C(1-z) are the vertices of an equilateral triangle, then value of z is
View Solution
