Three particles of masses 1 g, 2g and 3 g are kept at points (2cm,0), (0.6 cm), (4cm, 3cm) find moment of inertia of all three particles (in gm−cm(2)) about (a) x-axis
(b). Y-axis
(c). Z-axis.
To find the moment of inertia of three particles about the x-axis, y-axis, and z-axis, we will use the formula for the moment of inertia I=∑mir2i, where mi is the mass of the particle and ri is the perpendicular distance from the axis of rotation to the particle.
Given Data:
- Masses and positions of the particles:
- Particle 1: Mass = 1 g, Position = (2 cm, 0 cm)
- Particle 2: Mass = 2 g, Position = (0 cm, 6 cm)
- Particle 3: Mass = 3 g, Position = (4 cm, 3 cm)
(a) Moment of Inertia about the x-axis (Ix):
1. For Particle 1:
- Distance from x-axis = y-coordinate = 0 cm
- I1x=m1⋅(0)2=1⋅0=0
2. For Particle 2:
- Distance from x-axis = y-coordinate = 6 cm
- I2x=m2⋅(6)2=2⋅36=72
3. For Particle 3:
- Distance from x-axis = y-coordinate = 3 cm
- I3x=m3⋅(3)2=3⋅9=27
4. Total Moment of Inertia about x-axis:
Ix=I1x+I2x+I3x=0+72+27=99 gm-cm2
(b) Moment of Inertia about the y-axis (Iy):
1. For Particle 1:
- Distance from y-axis = x-coordinate = 2 cm
- I1y=m1⋅(2)2=1⋅4=4
2. For Particle 2:
- Distance from y-axis = x-coordinate = 0 cm
- I2y=m2⋅(0)2=2⋅0=0
3. For Particle 3:
- Distance from y-axis = x-coordinate = 4 cm
- I3y=m3⋅(4)2=3⋅16=48
4. Total Moment of Inertia about y-axis:
Iy=I1y+I2y+I3y=4+0+48=52 gm-cm2
(c) Moment of Inertia about the z-axis (Iz):
1. For Particle 1:
- Distance from z-axis = √(2)2+(0)2=2 cm
- I1z=m1⋅(2)2=1⋅4=4
2. For Particle 2:
- Distance from z-axis = √(0)2+(6)2=6 cm
- I2z=m2⋅(6)2=2⋅36=72
3. For Particle 3:
- Distance from z-axis = √(4)2+(3)2=√16+9=5 cm
- I3z=m3⋅(5)2=3⋅25=75
4. Total Moment of Inertia about z-axis:
Iz=I1z+I2z+I3z=4+72+75=151 gm-cm2
Final Results:
- Moment of Inertia about x-axis: Ix=99 gm-cm2
- Moment of Inertia about y-axis: Iy=52 gm-cm2
- Moment of Inertia about z-axis: Iz=151 gm-cm2
- Masses and positions of the particles:
- Particle 1: Mass = 1 g, Position = (2 cm, 0 cm)
- Particle 2: Mass = 2 g, Position = (0 cm, 6 cm)
- Particle 3: Mass = 3 g, Position = (4 cm, 3 cm)
1. For Particle 1:
- Distance from x-axis = y-coordinate = 0 cm
- I1x=m1⋅(0)2=1⋅0=0
- Distance from x-axis = y-coordinate = 6 cm
- I2x=m2⋅(6)2=2⋅36=72
- Distance from x-axis = y-coordinate = 3 cm
- I3x=m3⋅(3)2=3⋅9=27
Ix=I1x+I2x+I3x=0+72+27=99 gm-cm2
1. For Particle 1:
- Distance from y-axis = x-coordinate = 2 cm
- I1y=m1⋅(2)2=1⋅4=4
- Distance from y-axis = x-coordinate = 0 cm
- I2y=m2⋅(0)2=2⋅0=0
- Distance from y-axis = x-coordinate = 4 cm
- I3y=m3⋅(4)2=3⋅16=48
Iy=I1y+I2y+I3y=4+0+48=52 gm-cm2
1. For Particle 1:
- Distance from z-axis = √(2)2+(0)2=2 cm
- I1z=m1⋅(2)2=1⋅4=4
- Distance from z-axis = √(0)2+(6)2=6 cm
- I2z=m2⋅(6)2=2⋅36=72
- Distance from z-axis = √(4)2+(3)2=√16+9=5 cm
- I3z=m3⋅(5)2=3⋅25=75
Iz=I1z+I2z+I3z=4+72+75=151 gm-cm2
- Moment of Inertia about x-axis: Ix=99 gm-cm2
- Moment of Inertia about y-axis: Iy=52 gm-cm2
- Moment of Inertia about z-axis: Iz=151 gm-cm2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Solved Examples
25 Videos
- ROTATIONAL MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Miscellaneous Examples
2 Videos
- ROTATIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONEXERCISE - (C) Chapter Exercises
39 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
Similar Questions
Particles of masses 1g, 2g, 3g ….100g are kept at the marks 1cm, 2cm, 3cm …., 100 cm respectively on a metre scale. Find the moment of inertia of the system of particles about a perpendicular bisector of the metre scale.
View SolutionThree particles, each of mass 200 g are kept at the corners of an equilateral triangle of side 10 cm. Find the moment of inertia of the system about an axis
a. joining two of the particles
b. passing through one of the particle perpendicular to the plane of the particles.
View SolutionA particle of mass 1 kg is kept at (1m,1m,1m). The moment of inertia of this particle about Z-axis would be
View SolutionThe moment of inertia of a cylinder of mass 1000gm and radius 20cm about the axis AB shown in figure is

View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
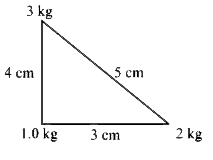
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :

View SolutionTwo identical spheres each of mass 1.20 kg and radius 10.0 cm are fixed at the ends of a light rod so that the separation between the centers is 50.0 cm. Find the moment of inertia of the system about an axis perpendicular to the rod passing through its middle point.
View SolutionThree particles of masses m,m and 4kg are kept at a verticals of triangle ABC. Coordinates of A, B and C are (1,2) , (3,2) and (−2,−2) respectively such that the centre of mass lies at origin. Find the value of mass m.
Hint. xcm=∑3i−1mixi∑3i=1mi, ycm=∑3i=1miyi∑3i=1mi
View SolutionThree particles of masses m,m and 4kg are kept at a verticals of triangle ABC. Coordinates of A, B and C are (1,2) , (3,2) and (−2,−2) respectively such that the centre of mass lies at origin. Find the value of mass m.
Hint. xcm=∑3i−1mixi∑3i=1mi, ycm=∑3i=1miyi∑3i=1mi
View SolutionA particle of mass 200g is attached to an ideal string of length 1.30 m whose upper end is fixed to ceiling. The particle is made to revolve in a horizontal circle of radius 50 cm. The tension in the string is (g=10ms2)
View Solution
