Three studends X,Y and Z performed an experiment for studying variation of a.c. with angular frequency in series LCR circuit, and obtained the graphs shown in Fig. They all used a.c. soucre of same rms value and inductance of same value. What can we conclude about (i) Capacitance value (ii) Resistance value used by them? In which case will the quality factor be maximum? What we conclude about nature of impedance of set up at frequency ω0 ?
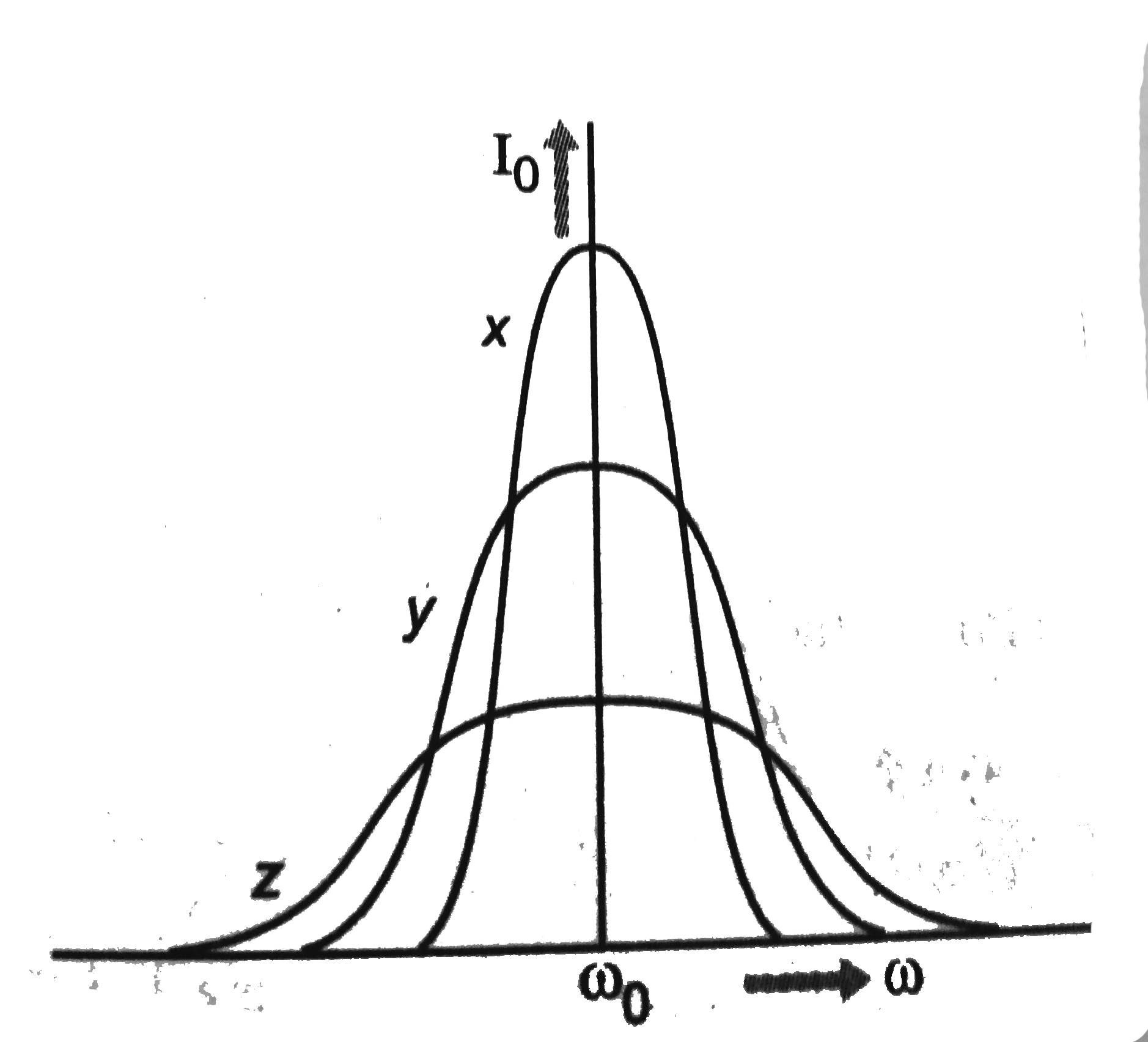
For resonance, XL=XC. As L is same, therefore, capactance used by all the three students must be the same for same value of ω0.
Maximum value of current is inversely proportional to R. Therefore, resistance used by students X is lowest and resistance used by student Z is the highest.
At ω0, impedance is equal to ohmic resistance only.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Solved Examples (b)1videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Short Answer Qusetions2videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
- BOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise191videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - II Focus multiple choice question5videos
ELECTROMAGNETIC WAVES
Similar Questions
Mention the factors on which the resonant frequency of a series LCR circuit depends. Plot a graph showing variation of impedance of a series LCR circuit with the frequency of the applied a.c. source.
View SolutionIn a series LCR circuit, how can we achieve the maximum power by choosing appropriate value of capacitance of the capacitor in the circuit ?
View Solution
Knowledge Check
- Question 1 - Select One
What can be concluded about the values of ΔH and ΔS from this graph ?
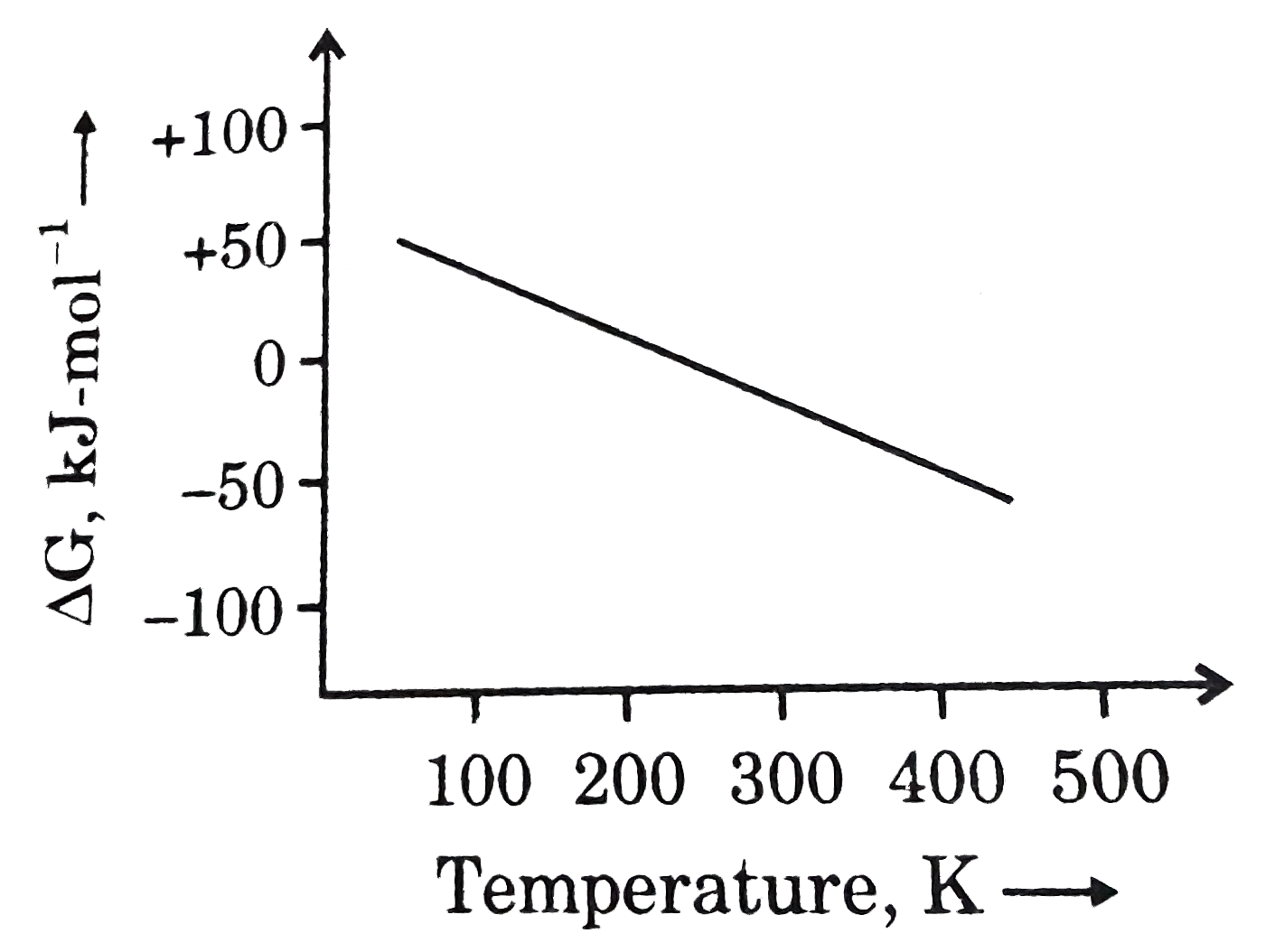 AΔH>0,ΔS>0BΔH>0,ΔS<0CΔH<0,ΔS>0DΔH<0,ΔS<0
AΔH>0,ΔS>0BΔH>0,ΔS<0CΔH<0,ΔS>0DΔH<0,ΔS<0 - Question 2 - Select One
From the matrix equation AB=AC, we conclude B=C provided.
AA is singularBA is non-singularCA is symmetricDA is a square - Question 3 - Select One or More
In an ac circuit shown in fig , the supply voltage has a constant rms value v but variable frequency f. At resonance, the circuit
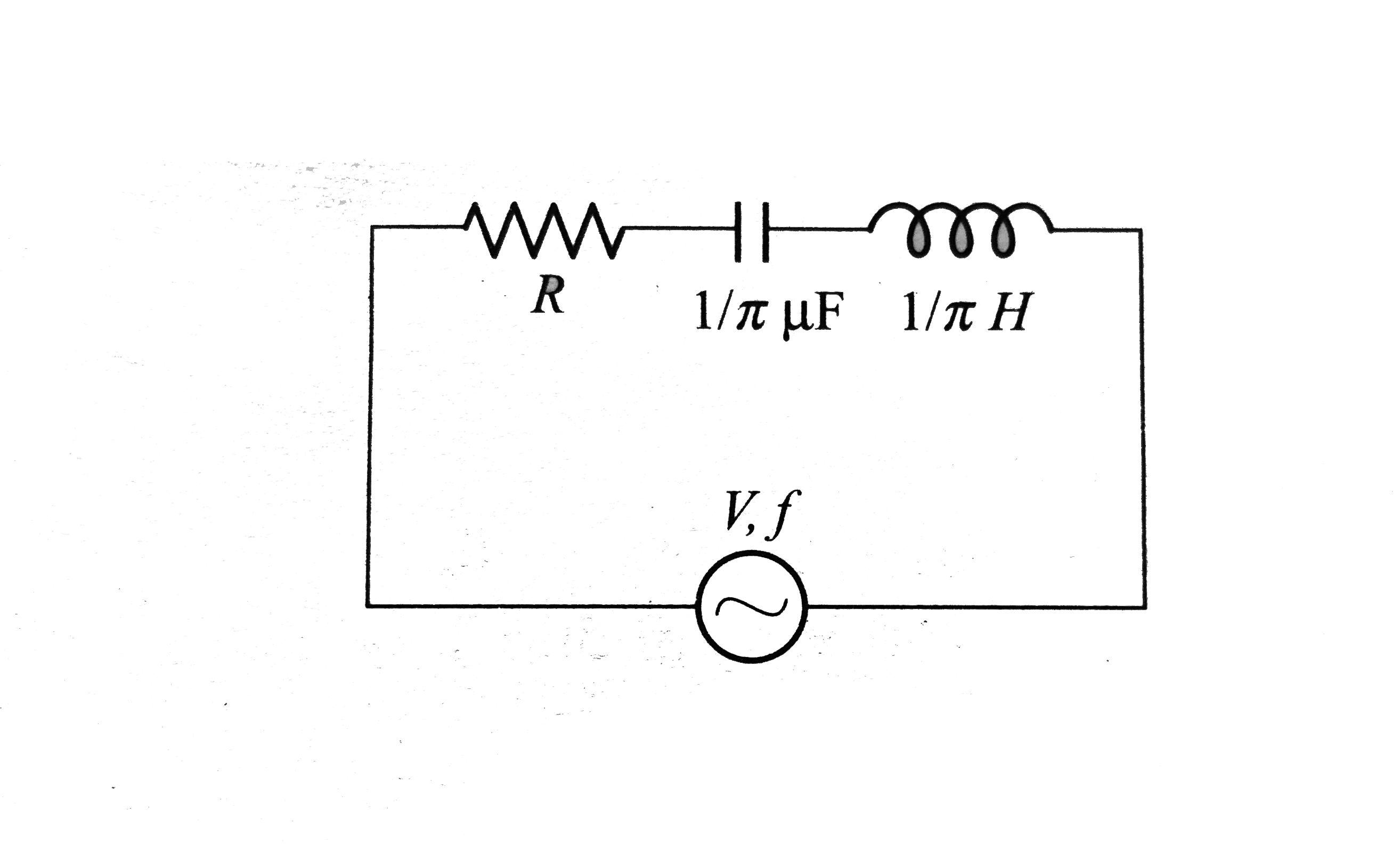 Ahas current I given by I=VRBhas a resonance frequenv=cy 500 HzChas a voltage across the capacitor which is 180∘ out of phase with that across the inductorDHas a current given by I=V√R2+(1π+1π)2
Ahas current I given by I=VRBhas a resonance frequenv=cy 500 HzChas a voltage across the capacitor which is 180∘ out of phase with that across the inductorDHas a current given by I=V√R2+(1π+1π)2
What is the maximum value of an ac voltage whose rms value is 220 V?
View SolutionA series LCR circuit is shown in the figure below.

Determine the source frequency which drives the circuit in resonance . Also obtain the value of Q - factor of the circuit.View SolutionA resistance R and an inductance L are connected in series in an a.c. circut. If ω is the angular frequency of the source, then the power factor is given by
View SolutionIn LCR circuit, capacitor C is changed to 4C, then what should be the value of L to keep resonance frequency same
View SolutionWhen the frequency of the AC voltage applied to a series LCR circuit is gradually increased from a low value, the impedance of the circuit
View Solution

