To required kinetic energy of an object of mass m, so that it may escape, will be
14mgR
12mgR
mgR
2mgR
The correct Answer is:C
KE=12mv2e=12m(2gR)=mgR
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (B) Chapter Exercises
31 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Check Point 10.6
20 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
What is the kinetic energy of an object ?
View SolutionThe kinetic energy of an object of mass m moving with a velocity of 5 m//s is 25 J. What will be its kinetic energy when its velocity is doubled ? What will be its kinetic energy - when its velocity si increased three times ?
View SolutionA satellite with kinetic energy Ek is revolving round the earth in a circular orbit. How much more kinetic energy should be given to it so that it may just escape into outer space
View SolutionWrite an expression for kinetic energy of an object.
View SolutionIf Me is the mass of earth and Mm is the mass of moon (Me=81Mm). The potential energy of an object of mass m situated at a distance R from the centre of earth and r from the centre of moon, will be :-
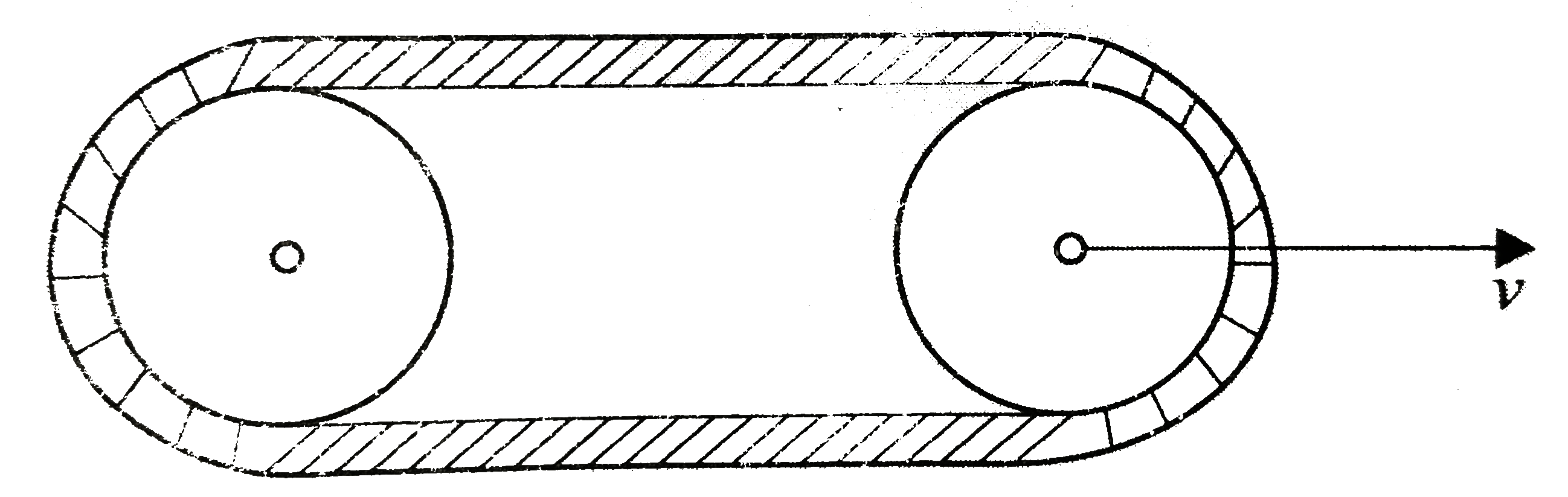
View SolutionCalculate the kinetic energy of a tractor crawler belt of mass m if the tractor moves with a velocity v. There is no slipping. Neglect the size of the wheels.
View SolutionThe total mechanical energy of an object of mass m projected from surface of earth with escape speed is
View SolutionThe kinetic energy of a mass connected to a spring will be maximum in :
View SolutionIf M be the mass of the earth, R its radius (assumed spherical) and G gravitational constant, then the amount of work that must be done on a body of mass m, so that it completely escapes from the gravity of the earth of the earth is given by
View SolutionA particle of mass m has half the kinetic energy of another particle of mass m2. If the speed of the heavier particle is increased by 2ms−1 its new kinetic energy becomes equal to t he original kinetic energy of the lighter particle. The ratio of the orighinal speeds of the lighter and heavier particle is
View Solution
