Two bar magnets placed together in a vibration magnetometer take 3 seconds for 1 vibration. If one magnet is reversed, the combination takes 4 seconds for 1 vibration. Find the ratio of their magnetic moments.
To solve the problem, we need to analyze the behavior of two bar magnets in a vibration magnetometer under two different configurations. Let's break it down step by step.
Step 1: Understand the Initial Setup
We have two bar magnets with magnetic moments m1 and m2. When placed together in the same direction, the total magnetic moment M is given by:
M=m1+m2
Step 2: Write the Expression for the Time Period
The time period T of the vibration magnetometer is given by the formula:
T=2π√IM⋅B
where:
- I is the moment of inertia (which remains constant),
- B is the magnetic field (which also remains constant).
For the first configuration (both magnets in the same direction), the time period T1 is:
T1=2π√I(m1+m2)⋅B
Given that T1=3 seconds, we can write:
3=2π√I(m1+m2)⋅B
Step 3: Analyze the Second Configuration
When one of the magnets is reversed, the total magnetic moment becomes:
M′=m1−m2
The time period T2 for this configuration is:
T2=2π√I(m1−m2)⋅B
Given that T2=4 seconds, we can write:
4=2π√I(m1−m2)⋅B
Step 4: Set Up the Equations
From the two equations for T1 and T2, we can square both sides to eliminate the square root:
1. For T1:
9=4π2I(m1+m2)⋅B
2. For T2:
16=4π2I(m1−m2)⋅B
Step 5: Derive the Ratio
Now, we can express these equations in terms of m1+m2 and m1−m2:
916=(m1−m2)⋅B(m1+m2)⋅B
This simplifies to:
916=m1−m2m1+m2
Step 6: Cross-Multiply and Rearrange
Cross-multiplying gives:
9(m1+m2)=16(m1−m2)
Expanding both sides:
9m1+9m2=16m1−16m2
Rearranging terms:
9m1+9m2+16m2=16m1
This simplifies to:
9m1+25m2=16m1
Thus, we have:
7m1=25m2
Step 7: Find the Ratio
Dividing both sides by m2:
m1m2=257
Thus, the ratio of their magnetic moments is:
25:7
We have two bar magnets with magnetic moments m1 and m2. When placed together in the same direction, the total magnetic moment M is given by:
M=m1+m2
The time period T of the vibration magnetometer is given by the formula:
T=2π√IM⋅B
where:
- I is the moment of inertia (which remains constant),
- B is the magnetic field (which also remains constant).
T1=2π√I(m1+m2)⋅B
Given that T1=3 seconds, we can write:
3=2π√I(m1+m2)⋅B
When one of the magnets is reversed, the total magnetic moment becomes:
M′=m1−m2
The time period T2 for this configuration is:
T2=2π√I(m1−m2)⋅B
Given that T2=4 seconds, we can write:
4=2π√I(m1−m2)⋅B
From the two equations for T1 and T2, we can square both sides to eliminate the square root:
1. For T1:
9=4π2I(m1+m2)⋅B
2. For T2:
16=4π2I(m1−m2)⋅B
Now, we can express these equations in terms of m1+m2 and m1−m2:
916=(m1−m2)⋅B(m1+m2)⋅B
This simplifies to:
916=m1−m2m1+m2
Cross-multiplying gives:
9(m1+m2)=16(m1−m2)
Expanding both sides:
9m1+9m2=16m1−16m2
Rearranging terms:
9m1+9m2+16m2=16m1
This simplifies to:
9m1+25m2=16m1
Thus, we have:
7m1=25m2
Dividing both sides by m2:
m1m2=257
Thus, the ratio of their magnetic moments is:
25:7
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - MAGNETISMEXERCISE - EXERCISE (LONG ANSWER QUESTIONS )6videos
MAGNETISM
- BOOK - AAKASH SERIESCHAPTER - MAGNETISMEXERCISE - EXERCISE (SHORT ANSWER QUESTIONS )6videos
MAGNETISM
- BOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)10videos
LOGIC GATES
- BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANEEXERCISE - EXERCISE-3 (Circular Motion)7videos
MOTION IN A PLANE
Similar Questions
The time period of viberation of two magnets in sum position (magnets placed with similar poles on one sides one above the other) is 3s. When polarity of weaker magnet is reversed the combination makes 12 oscillations per minutes. What is the ratio of magnetic moments of two magnets?
View SolutionTime periods of vibration of two bar magnets in sum and difference positions are 4 s and 6 s respectively . The ratio of their magnetic moments M1M2 is
View SolutionFor a vibration magnetometer, the time period of suspended bar magnet can be reduced by -
View SolutionThe combination of two bar magnets makes 10 oscillations per second in an oscillation magnetometer when like poles are tied together and 2 oscillations per second when unlike poles are tied together. Find the ratio of the magnetic moments of the magnets. Neglect any induced magnetism.
View SolutionThe time period of the magnetic in an oscillation pmagnetometer in the earth magnetic field is 2s. A short bar magnet is placed to the north of the magnetometer, at a separation 10cm from the oscillation magnet, with its north pole pointing towards north. The time period beceomes half. Calculate the magnetic moment of this short magnet.
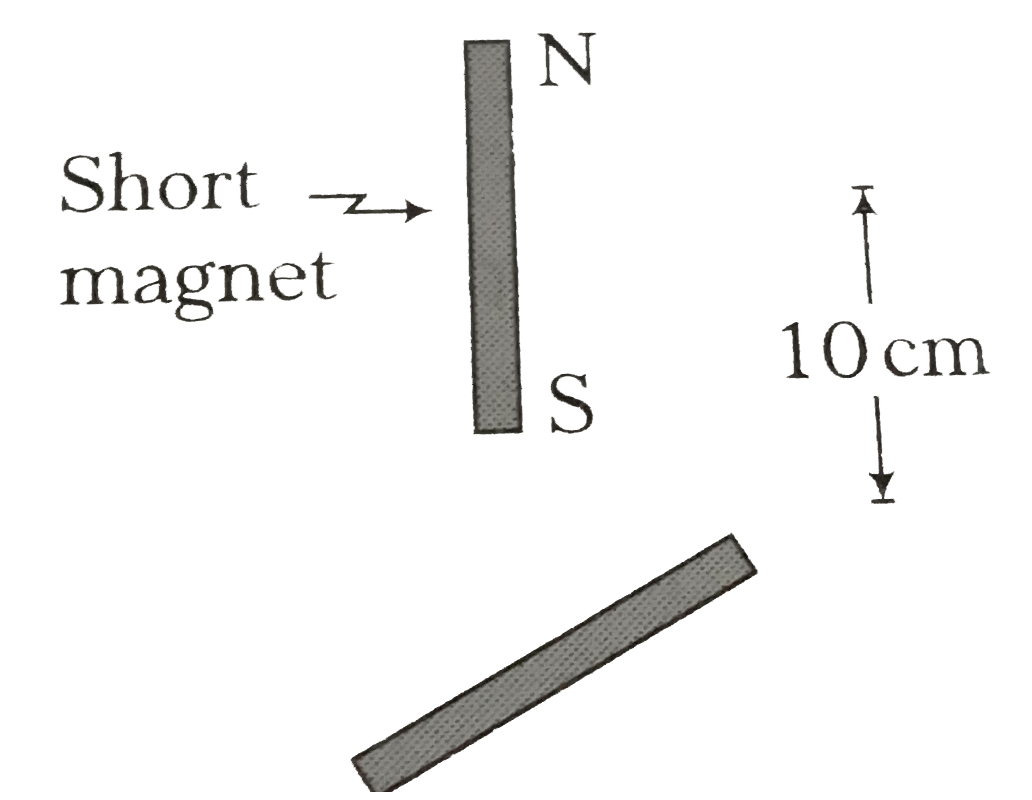 View Solution
View SolutionTwo magnets are held together in a vibration magnetometer and are allowed to oscillate in the earth's magnetic field with like poles togather, 12 oscillations per minute are made but for unlike poles togather only 4 oscillations per minute are axecuted. The ratio of their magnetic miments is
View SolutionThe perio of oscillation of a magnet in vibration magnetometer is 2 sec. The period of oscillation of a magnet whosr magnetic moment is four times that of the first magnet is
View SolutionThe combination of two bar magnets makes 20 oscillations per minute in an oscillation magnetometer when like poles are tied together and 5 oscillations per minute when unlike poles are kept together. Then ratio of the magnetic moment of magnets is
View SolutionThe time period of oscillation of a magnet in a vibration magnetometer is 1.5 seconds. The time period of oscillation of another of another magnet similar in size, shap and mass but having one-fourth magnetic moment than that of first magnet, oscillating at same place will be
View SolutionWith a standard rectangular bar magnet the time period of a vibration magnetometer is 4 s. The bar magnet is cut parallel to its length into four equal pieces. The time period of vibration magnetometer when one piece is used (in second) (bar magnet breadth is small) is
View Solution

