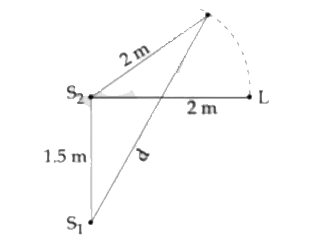
Two coherenet source of sound S1 and S2 produce sound waves of the same wavelength λ=1 m , in phase , S1 and S2 are placed 1.5 m a part ( see fig). A listener , loacted at L , directly in fornt of S2 finds that the intensity is at a minimum when he is 2 m away form S2. The listener moves aways form S1 keeping his distance for S2 fixed . The adjacent maximum of inesity is observed when the listener is at a distane d from S1 . Then d is
12m
5m
2m
3m
Step by step video & image solution for Two coherenet source of sound S_(1) and S_(2) produce sound waves of the same wavelength lambda = 1 m , in phase , S_(1) and S_(2) are placed 1.5 m a part ( see fig). A listener , loacted at L , directly in fornt of S_(2) finds that the intensity is at a minimum when he is 2 m away form S_(2) . The listener moves aways form S_(1) keeping his distance for S_(2) fixed . The adjacent maximum of inesity is observed when the listener is at a distane d from S_(1) . Then d is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two source of sound S1 and S2 produce sound waves of same frequency 660 Hz. A listener is moving from source S1 towards S2 with a constant speed um/s and he hears 10 beat/s. Then, u is equal to ________ (in m/s).
View SolutionThe time period of a vibrating source of sound is 0.01s. If speed of sound is 330m/s, what is the wavelength?
View Solution
Knowledge Check
Two sound waves of wavelengths λ1 and λ2(λ2>λ1) produces nbeats/s , the speed of sound is
Anλ1λ2λ2−λ1Bn(1λ1−1λ2)Cn(λ2−λ1)Dn(λ2+λ1)Two sound waves of wavelength 1m and 1.01m in a gas produce 10 beats in 3s. The velocity of sound in the gas is
A330m/sB337m/sC360m/sD300m/sTwo monochromatic coherent point sources S1 and S2 are separated by a distance L. Each sources emits light of wavelength λ, where L>>λ. The line S1S2 when extended meets a screen perpendicular to it at point A. Then
Athe interference fringe on the screen are straight lines shapeBthe interference fringes on the screen are strainght lines perpendicular to the line S1S2ACpoint A is an intensity maxima if L=nλDpoint A is always an intensity maxima for any separation L
Two coherent point sources S1 and S2 vibrating in phase emit light of wavelength λ. The separation between the sources is 2λ. Consider a line passing through S1 and perpendicular to line S1S2. Find the position of farthest and nearest minima.
 .
.
View SolutionTwo source of sound, S1 and S2, emitting waves of equal wavelength 2cm, are placed with a separation of 3cm between them. A detector can be moved on a line parallel to S1S2 and at a distance 24cm from it. Initially, the detecor is equidistant from the two sources. Assuming that the waves emitted by the sources are in phase, find the minimum distance through which the detector should be shifted to detect a minimum of sound.
View SolutionS1 and S2 are two loudspeakers with the same frequency of 165 Hz and acoustic output 1.2×10−3 and 1.8×10−3 watts respectively/ They vibrate in the same phase. P is a point at a distance 4m from S1 and 3m from S2
What is the intensity of sound at P if S1 is turned off (S2) on)
View SolutionS1andS2 are two coherent sources. The intensity of both sources are same. If the intensity at the point of maxima is 4Wm−2, the intensity of each source is
View SolutionTwo sound waves each of wavelength λ and having the same amplitude A form two sources S1andS2 interfere at a point P. If the path difference S2P−S1P=λ3, then the amplitude of the resultant were at P will be
View Solution
