Two coils are being moved out of magnetic field, one coil is moved rapidly and the other slowly. In which case is more work done and why?
To solve the question of which coil does more work when moved out of a magnetic field, we can analyze the situation step by step.
1. Understanding the Scenario:
- We have two coils being moved out of a magnetic field: one coil is moved rapidly, and the other is moved slowly.
2. Applying Faraday's Law of Electromagnetic Induction:
- According to Faraday's law, the induced electromotive force (emf) in a coil is given by the formula:
emf=−dΦdt
where Φ is the magnetic flux through the coil.
3. Rate of Change of Magnetic Flux:
- When the coil is moved rapidly out of the magnetic field, the rate of change of magnetic flux (dΦ/dt) is greater compared to when the coil is moved slowly. This means that:
- Rapid movement → Higher dΦdt
- Slow movement → Lower dΦdt
4. Induced EMF:
- Since the induced emf is directly proportional to the rate of change of magnetic flux, a higher rate of change results in a greater induced emf:
- Rapid movement → Higher induced emf
- Slow movement → Lower induced emf
5. Work Done:
- The work done (W) in moving the coil out of the magnetic field can be expressed as:
W=emf×Q
where Q is the charge moved through the coil. A greater induced emf means that more work is done when moving the coil rapidly.
6. Conclusion:
- Therefore, more work is done when the coil is moved rapidly out of the magnetic field compared to when it is moved slowly. The reason is that the induced emf is greater in the case of rapid movement, leading to more work being done.
Final Answer:
More work is done when the coil is moved rapidly out of the magnetic field because the induced emf is greater due to a higher rate of change of magnetic flux.
- We have two coils being moved out of a magnetic field: one coil is moved rapidly, and the other is moved slowly.
- According to Faraday's law, the induced electromotive force (emf) in a coil is given by the formula:
emf=−dΦdt
where Φ is the magnetic flux through the coil.
- When the coil is moved rapidly out of the magnetic field, the rate of change of magnetic flux (dΦ/dt) is greater compared to when the coil is moved slowly. This means that:
- Rapid movement → Higher dΦdt
- Slow movement → Lower dΦdt
- Since the induced emf is directly proportional to the rate of change of magnetic flux, a higher rate of change results in a greater induced emf:
- Rapid movement → Higher induced emf
- Slow movement → Lower induced emf
- The work done (W) in moving the coil out of the magnetic field can be expressed as:
W=emf×Q
where Q is the charge moved through the coil. A greater induced emf means that more work is done when moving the coil rapidly.
- Therefore, more work is done when the coil is moved rapidly out of the magnetic field compared to when it is moved slowly. The reason is that the induced emf is greater in the case of rapid movement, leading to more work being done.
More work is done when the coil is moved rapidly out of the magnetic field because the induced emf is greater due to a higher rate of change of magnetic flux.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problems (Level-I)28videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problems (Level-II)14videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise (Short Answer Questions)21videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)26videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II22videos
ELECTROMAGNETIC WAVES
Similar Questions
To make the field radial in a moving coil galvanometer
View SolutionTwo circular coils are made of two identicle wires of length 20 cm. One coil has number of turns 9 and the other has 3. If the same current flows through the coils then the ratio of magnetic fields of induclion at their centres is
View SolutionA flip coil consits of N turns of circular coils which lie in a uniform magnetic field. Plane of the coils is perpendicular to the magnetic field as shown in figure. The coil is connected to a current integrator which measures the total charge passing through it. The coil is turned through 180∘ about the diameter. The charge passing through the coil is
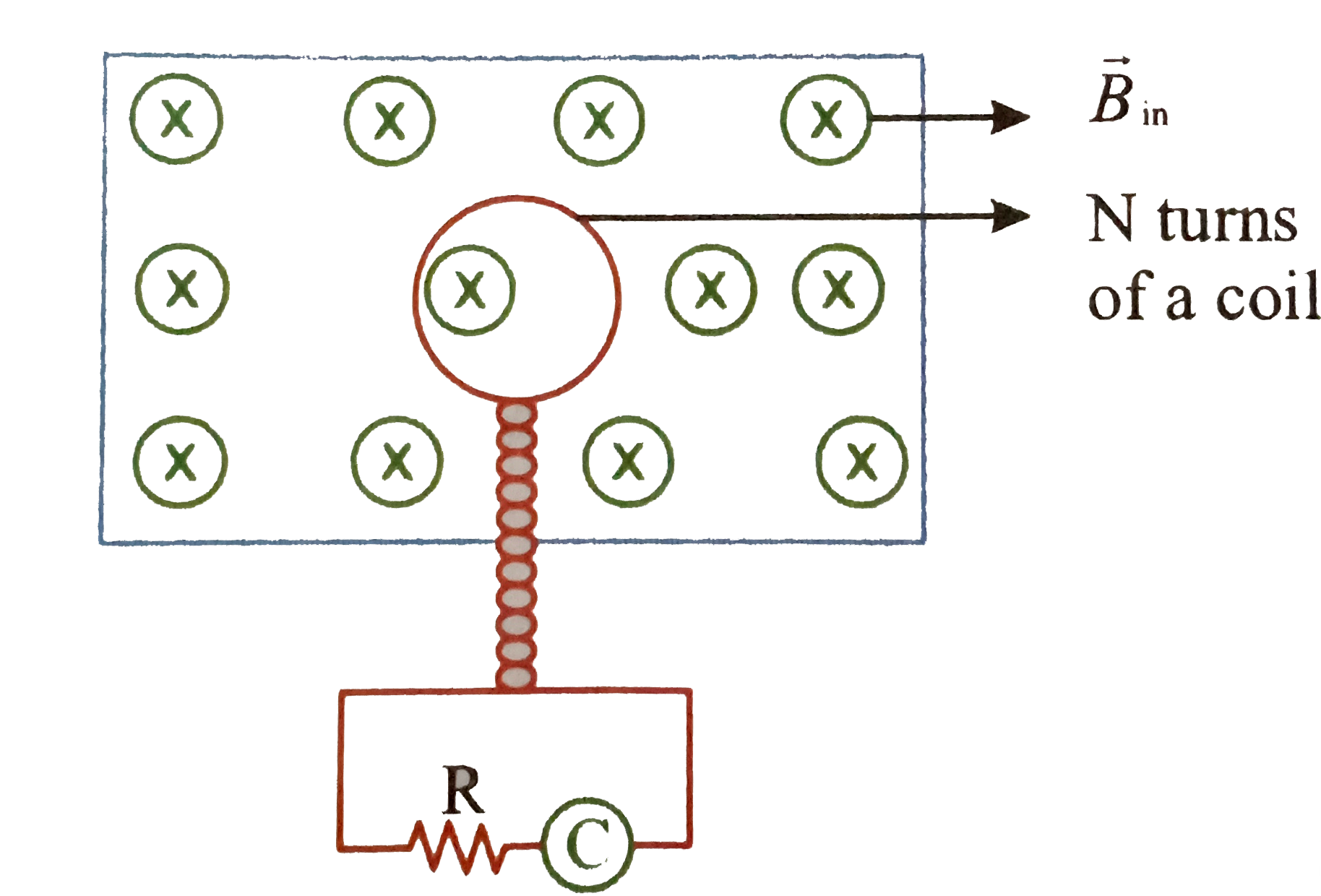 View Solution
View SolutionWhat is the function of the radial magnetic field in the moving coil galvanometer?
View SolutionTwo similar coils are kept mutually perpendicular such that their centres coincide. At the centre, find the ratio of the magnetic field due to one coil and the resultant magnetic field by both coils, if the same current is flown
View SolutionTwo similar coils are kept mutually perpendicular such that their centres coincide. At the centre, find the ratio of the magnetic field due to one coil and the resultant magnetic field by both coils, if the same current is flown
View SolutionTwo heater coils made of the same material are connected in parallel across the mains : the length and the diameter of one coil is double that of the other . Which of them will produce more heat?
View SolutionA magnetic dipole with magnetic moment M is placed at right angles to a magnetic field B. If it is rotated by an angle of 180∘ , the total work done is
View SolutionTwo identical coils, one of copper and the other of iron, are rotated with the same angular velocity ω in a uniform magnetic field. In which case the induced e.m.f. is more and why?
View SolutionA magnet is parallel to a uniform magnetic field. If it is rotated by 60∘, the work done is 0.8 J. How much work is done in moving it 30∘ further
View Solution

