Two concentric circular coils, one of small radius and the other of large radius , such that , are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
 Solution in Gujrati
Solution in Gujrati
Consider two extremely long co-axial circular coils (or solenoids) of equal length l, with smaller one inside the bigger one. Let small and big coils be coil no. 1 and coil no. 2 respectively. Their common area of cross section is suppose .
When amount of current is passed through turns of small coil, magnetic flux linked with big coil is,
(Here, magnetic field is present only inside the small coil and so cross-sectional area of small coil is considered).
Here =mutual inductance of second coil towards first coil.
....(1)
Similarly when amount of current is passed through no. of turns of big coil, magnetic flux linked with small coil is,
Here =mutual inductance of first coil towards second coil.
....(2)
Equations (1) and (2) prove that
...(3)
From equations (1),(2) and (3)
(where =cross-sectional area of small coil)
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Numerical From Textual Exercise17videos
ELECTROMAGNETIC INDUCTION
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Numerical From DARPAN Based on Textbook10videos
ELECTROMAGNETIC INDUCTION
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section A HOTS2videos
ELECTROMAGNETIC INDUCTION
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION D MCQS ASKED IN COMPETITIVE EXAMES (MCQS AKSED IN BOARD EXAM AND GUJCET)14videos
ELECTRIC CHARGES AND FIELDS
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - BOARD.S QUESTION PAPER MARCH - 2020 (PART - B) SECTION - C5videos
ELECTROMAGNETIC WAVES
Similar Questions
Predict the direction of induced current in the situations described by the following figures (a) to (d).
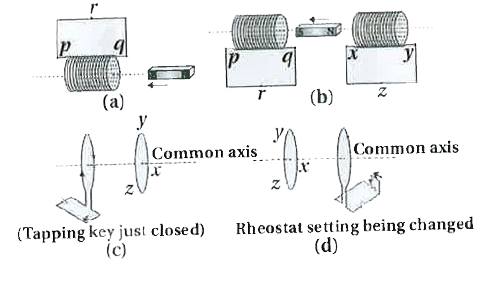 02:09View Solution
02:09View SolutionA conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the field. Some how, the radius of the loop starts shrinking at a constant rate of 2 mm/s. Find the induced emf in the loop at an instant when the radius becomes 2 cm solution.
03:01View SolutionGive the explanation of reason behind the origin of motional induced emf.
View SolutionWhen air is replaced by dielectric medium of constant K, the maximum force of attraction between two charges separated by distance d, ..........
02:37View SolutionDetermine the current in each branch of the given network.
 02:38View Solution
02:38View SolutionTwo concentric sphereical shels have masses M1,M2andradiiR1,R2(R1+R2). What is the force exerted by this system on a particle of mass m1 if it is placed t a distance R1+R22 from the centre?
04:02View SolutionTwo circular coils, one of smaller radius r1 and the other of very large radius r2 are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
04:22View SolutionIn a △ABC if r1<r2<r3, then
05:17View SolutionIn ΔABC, if r1<r2<r3 then
03:19View SolutionIf r1andr2 represent radii of two concentric coil (r2>>r1) then give the expression for the coefficient of mutual inductance for the pair of coils.
View SolutionWhen two soap bubbles of radii r_1 and r_2 (r_2 lt r_1) adjoin, the radius of curvature of the common surface is
04:49View Solutionকোনাে তড়িৎবর্তনীতে e তড়িৎচালক বলবিশিষ্ট দুটি তড়িৎকোশকে শ্রেণি সমবায়ে বহিস্থ R রােধের সাথে যুক্ত করা হল কৌশদ্বয়ের অভ্যন্তরীণ রােধ যথাক্রমে r1এবং r2 | প্রবাহ চলাকালীন যদি প্রথম কোশের বিভবপার্থক্য শূন্য হয় তবে R এবং r1 ও r2 এর মধ্যে সম্পর্ক হবে R=r1+r2 R=r1−r2 R=½(r1+r2) R=½(r1−r2)
View SolutionTwo circular coils, one of smaller radius r_(1) and the other of very large radius r_(2) are placed co-axially with centres coinciding. Obtain the mutual inductance of the arrangement.
View Solutionत्रिज्या R1 एवं R2 के दो चालक लूपों को उनके सम्पाती केन्द्रों से समान तल रखा जाता है। यदि R1>R2 तो उनके मध्य अन्योन्य प्रेरकत्व M अनुक्रमानुपाती होगा -
03:38View SolutionTwo concentric spherical shells have masses M1,M2 and radii R1,R2(R1<R2). The force exerted by this system on a particle of mass m. If it is placed at a distance (R1+R22) from the centre.
03:46View SolutionThe magnitudes of the gravitational fields at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M are F1 and F2 respectively then
(a)F1F2=r1r2 if r1<R and r2<R
(b)F1F2=(r2r1)2 if r1>R and r2>R
(c)F1F2=r1r2 if r1>R and r2>R
(d)F1F2=(r1r2)2 if r1<R and r2<R02:41View Solution

