Two disc has same mass rotates about the same axis with their densities ρ1 and ρ2 respectively such that (ρ1>ρ2), then the relation between I1 and I2 will be
I1<I2
I1=I2
I1>I2
I1=2I2
The correct Answer is:A
I1I2=R21R22 …….(i)
M1=M2
ρ1πR21t=ρ2πR22t
ρ1ρ2=R22R21
∴ The equation (i) becomes,
I1I2=ρ2ρ1 as ρ1>ρ2
I1I2<1
∴I1<I2.
Topper's Solved these Questions
Similar Questions
V1 and V2 are the velocities of sound at the same temperature in two monoatomic gases of densities ρ1 and ρ2 respectively.If ρ1ρ2=14 then the ratio of velocities V1 and V2 is
View SolutionIf two liquids of same masses but densities ρ1andρ2 respectively are mixed, then the density of mixture is given by
View Solution
Knowledge Check
Two discs having same mass rotate about the same axes. If p1andp2 be the densities of two bodies (ρ1>ρ2), then what is the relation between I1andI2?
AI1<I2BI1=I2CI1>I2DI1=I2Two circular discs A and B have equal masses and uniform thickness but have densities ρ1 and ρ2 such that ρ1>ρ2 . Their moments of inertia is
AI1>I2BI1>>I2CI1<I2DI1=I2The velocities of sound at same temperature in two monoatomic gases of density ρ1 and ρ2 are v1 and v2 repectively , if ρ1ρ2=4, then the value of v1v2 will be
A4B2C12D14
If two liquids of same masses but densities ρ1andρ2, respectively are mixed, then the density of mixture is given by
View SolutionA solid uniform ball having volume V and density ρ floats at the interface of two unmixable liquids as shown in the figure. The densities of the upper and the lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2 . What fraction of the volume of the ball will be in the lower liquid ?

View SolutionIf two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
View SolutionA solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densityes ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
View SolutionA solid uniform ball having volume V and density ρ floats at the interface of two unmixible liquids as shown in Fig. 7(CF).7. The densities of the upper and lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2. What fractio9n of the volume of the ball will be in the lower liquid.
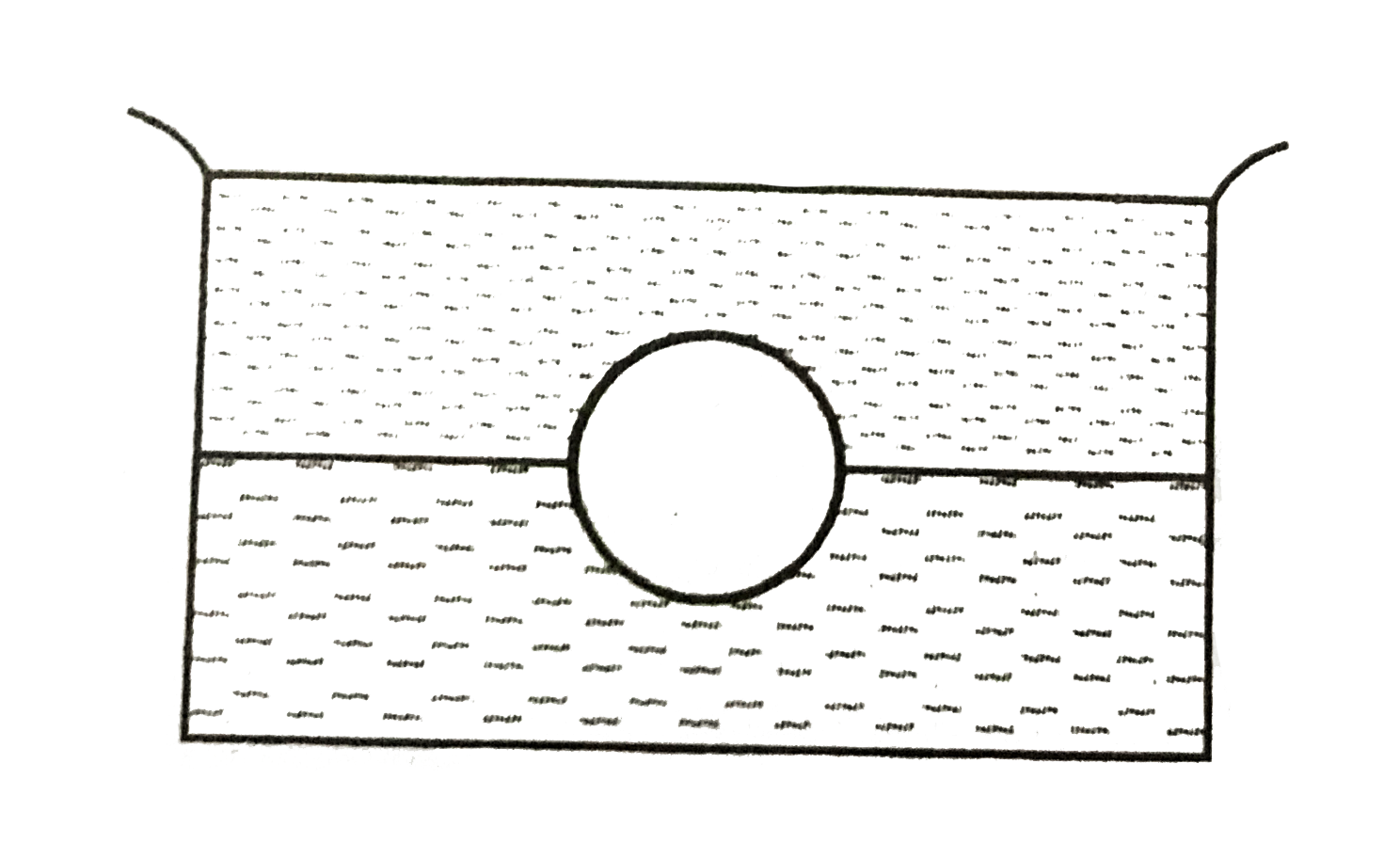
View Solution
