Two identical glass spheres filled with air are connected by a thin horizontal glass tube the glass tube contains a pellet of mercury at its mid-point Air in one sphere is at 0∘C and the other is at 20∘C if temperature of both the vessels are increased by 10∘C then neglecting the expansions of the bulbs and the tube
the mercury pellet gets displaced towards the sphere at lower temperature
the mercury pellet gets displaced towards the spehere at higher temperature
the mercury pellet does not get displaced at all
the temperature rise causes the pellet to expand without any displacement
The correct Answer is:C
To solve the problem step-by-step, we will analyze the situation involving the two glass spheres filled with air and connected by a glass tube containing a pellet of mercury.
Step 1: Understand the Initial Conditions
- We have two identical glass spheres.
- Sphere 1 is at a temperature of 0∘C (which is 273K).
- Sphere 2 is at a temperature of 20∘C (which is 293K).
- The glass tube contains a pellet of mercury at its midpoint.
Hint: Identify the initial temperatures and convert them to Kelvin for calculations.
Step 2: Use the Ideal Gas Law
The ideal gas law states that PV=nRT. Since the volume of the spheres and the tube are constant, we can express the pressures in terms of the number of moles of air and the respective temperatures.
For Sphere 1:
P1V=n1R(273)
For Sphere 2:
P2V=n2R(293)
Hint: Write down the ideal gas equations for both spheres.
Step 3: Relate the Number of Moles
From the equations above, we can express the pressures in terms of the number of moles:
P1=n1R(273)V
P2=n2R(293)V
Since the volume V and R are constant, we can relate n1 and n2:
n1n2=293273
Hint: Simplify the equations to find the relationship between n1 and n2.
Step 4: Increase the Temperature
Now, we increase the temperature of both spheres by 10∘C:
- New temperature of Sphere 1: 0+10=10∘C (which is 283K).
- New temperature of Sphere 2: 20+10=30∘C (which is 303K).
Hint: Update the temperatures after the increase.
Step 5: Calculate New Pressures
Using the ideal gas law again for the new temperatures:
For Sphere 1:
P′1=n1R(283)V
For Sphere 2:
P′2=n2R(303)V
Hint: Write down the new pressures after the temperature increase.
Step 6: Compare the New Pressures
Now, we can compare the new pressures:
P′1P′2=n1⋅283n2⋅303
Substituting n1/n2 from earlier:
P′1P′2=(293/273)⋅283303
Step 7: Analyze the Result
If P′1<P′2, the mercury pellet will move towards Sphere 2 (higher temperature). If P′1>P′2, it will move towards Sphere 1 (lower temperature). If they are equal, the pellet does not move.
Calculating the ratio:
P′1P′2=(293⋅283)(273⋅303)
After calculating, if this ratio equals 1, it indicates that the pressures are equal, meaning the mercury pellet does not get displaced.
Conclusion
After performing the calculations, we find that the mercury pellet does not get displaced at all.
Final Answer: The correct option is that the mercury pellet does not get displaced at all.
- We have two identical glass spheres.
- Sphere 1 is at a temperature of 0∘C (which is 273K).
- Sphere 2 is at a temperature of 20∘C (which is 293K).
- The glass tube contains a pellet of mercury at its midpoint.
The ideal gas law states that PV=nRT. Since the volume of the spheres and the tube are constant, we can express the pressures in terms of the number of moles of air and the respective temperatures.
P1V=n1R(273)
For Sphere 2:
P2V=n2R(293)
From the equations above, we can express the pressures in terms of the number of moles:
P1=n1R(273)V
P2=n2R(293)V
n1n2=293273
Now, we increase the temperature of both spheres by 10∘C:
- New temperature of Sphere 1: 0+10=10∘C (which is 283K).
- New temperature of Sphere 2: 20+10=30∘C (which is 303K).
Using the ideal gas law again for the new temperatures:
For Sphere 1:
P′1=n1R(283)V
For Sphere 2:
P′2=n2R(303)V
Now, we can compare the new pressures:
P′1P′2=n1⋅283n2⋅303
Substituting n1/n2 from earlier:
P′1P′2=(293/273)⋅283303
If P′1<P′2, the mercury pellet will move towards Sphere 2 (higher temperature). If P′1>P′2, it will move towards Sphere 1 (lower temperature). If they are equal, the pellet does not move.
P′1P′2=(293⋅283)(273⋅303)
After calculating, if this ratio equals 1, it indicates that the pressures are equal, meaning the mercury pellet does not get displaced.
After performing the calculations, we find that the mercury pellet does not get displaced at all.
Topper's Solved these Questions
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(NEET)
22 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(AIIMS)
28 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved Papers 2017(AIIMS)
26 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise 19.7
4 Videos
Similar Questions
Two vertical glass tibes filled with a liquid are connected at their lower ends by a horizontal capillary tube. One tube is surrounded by a bath containing ice and water at 0∘C and the other by hot water a t∘C. The difference in the height of the liquid in the two columns is Δh, and the height of the colume at 0∘C is h0. coefficient of volume expansion of the liquid is.

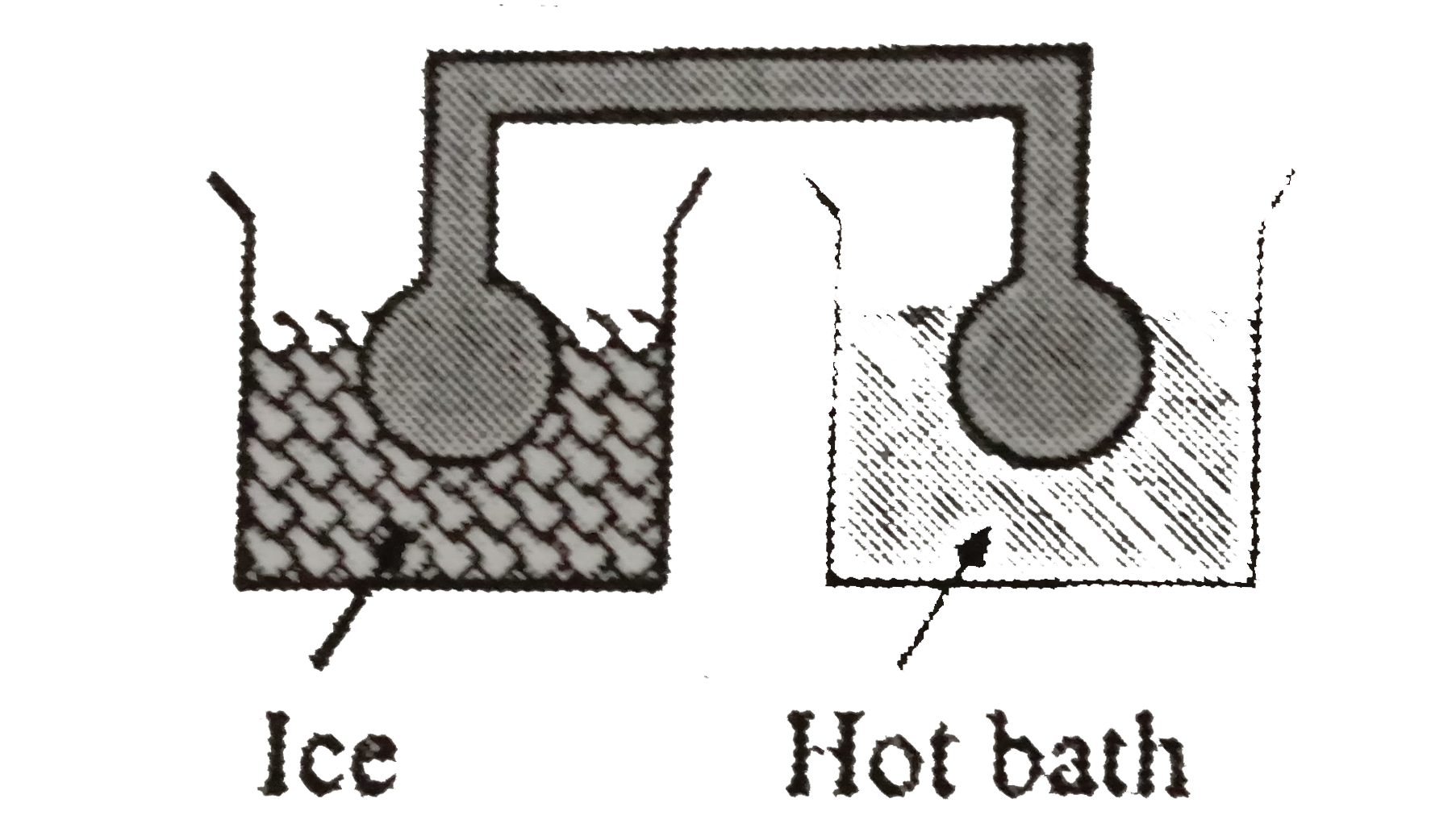
View SolutionTwo identical glass bulbs are interconnected by a thin glass tube. A gas is filled in these bulbs at N.T.P. If one bulb is placed in ice and another bulb is placed in hot bath, then the pressure of the gas becomes 1.5 times. The temperature of hot bath will be
View SolutionTwo glass bulbs of same volume containing a gas at NTP are connected by a very narrow tube. The pressure is 88.46cm of mercury when one bulb is kept in ice and the other in hot water. Calculate the temperature of the hot water.
View SolutionA long horizontal glass capillary tube open at both ends contains a mercury thread 1 m long at 0∘C. Find the length of the mercury thread, as read on this scale, at 100∘C.
View SolutionIn the given figure a glass tube lies horizontally with the middle 20cm containing mercury. The two ends of the tube contains air at 27∘C and at a pressure 76cm of mercuy. Now the air column on one side in maintained at 0∘C and the other side is maintained at 127∘C. Find the new length of the air column on the cooler side. Neglect the changes in the volume of mercury and of the glass.

View Solutiontwo glass bulbs of equal volume are connected by a narrow tube and are filled with a gas at 0(∘)C at a pressure of 76cm of mercury. One of the bulbs is then placed in melting ice and the other is placed in a water bath maintained at 62∘C.What is the new value of the pressure inside the bulbs? The volume of the connecting tube is neigligible.
View SolutionA glass tube sealed at both ends is 100cm long, It lies horizontally with the middle 10cm containing mercury. The two ends of the tube contain air at 27∘Cand at a pressure 76cm of mercury. The air column on one side is maintained at 0∘Cand the other side is maintained at 127∘C. Calculate the length of the air column on the cooler side. neglect the changes in the volume of mercury and of the glass.
View SolutionA cylindrical tube of uniform cross-sectional area A is fitted with two air tight frictionless position. The pistons are connected to each other by a metallic wire. Initially the pressure of the gas is po and temperature is To, atmospheric pressure is also po Now the temperature of the gas is increased to 2T0, the tension in the wire will be

View SolutionA sealed tube which can withstand a pressure of 3 atm sphere is filled with air at 27∘Cand760 mm pressure. The temperature above which the tube will burst will be:
View SolutionTwo spherical soap bubbles of diameters 10 cm and 6 cm are formed, one at each end of a narrow horizontal glass tube. If the surface tension of the soap solution is 0.03Nm−1, then the pressure difference in pascal between the two ends of the tube is
View Solution
