Two identical loudspeakers are driven in phase by a common oscillator at 800Hz and face each other at a distance of 1.25m. Locate the points along the line joining the two speakers where relative minimum of sound pressure amplitude would be expected. ( Use v=343m/s.)
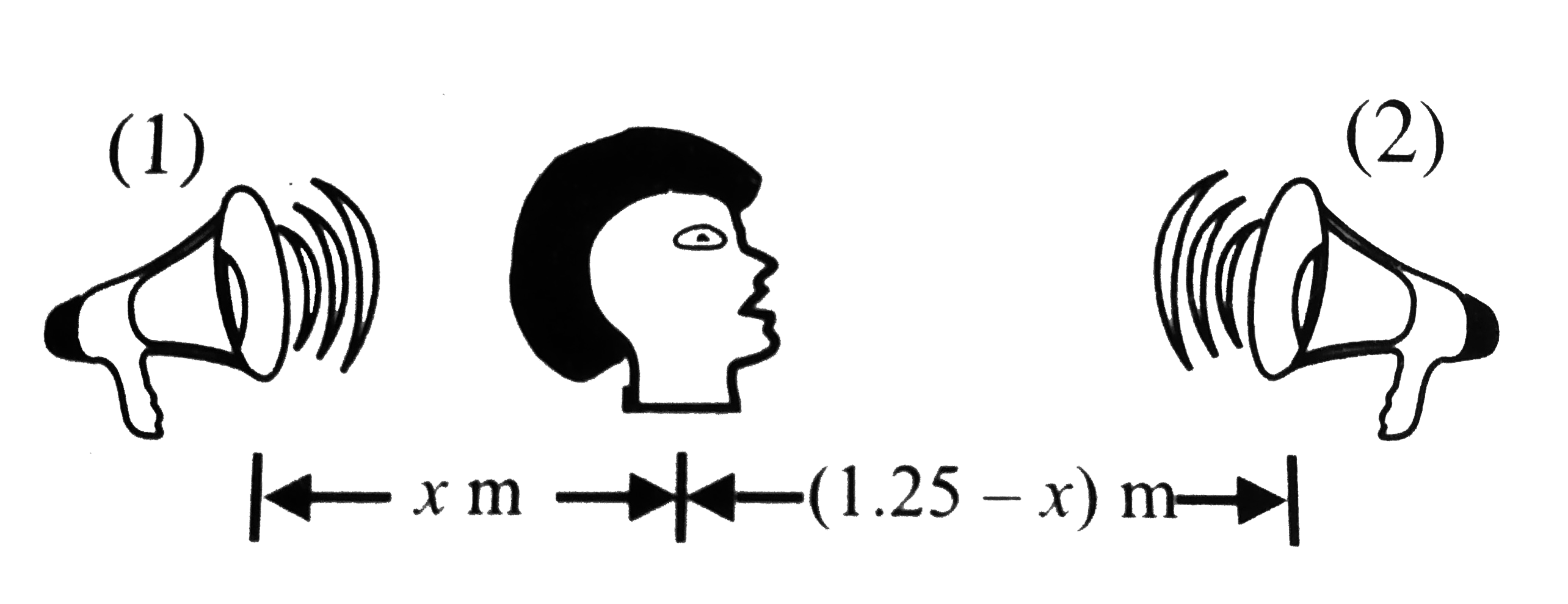
Two identical waves moving in opposite directions constitute a standing wave .We must find the nodes.
The wavelength is
λ=vf=343m/s800Hz=0.429m
The two waves moving in opposite directions along the line between the two speakers will add to produce a standing wave within this distance between nodes.
Distance node to node =λ/2=0.214m
Because the speakers vibrate in phase , air compressions from each will simultaneously reach the point halfway between the speakers , to produce antinode of pressure here . A node of pressure will be located at this distance on either side of the midpoint.
Distance node to antinode =λ/4=0.107m
Therefore nodes of sound pressure will appear at these distances from either speaker.
[1/2(1.25m)+0.107m=0.732m
and 1/2(1.25m)−0.107m=0.518m]
The standing wave contains a chain of equally spaced nodes at distances from either of the speakers
0.732m+0.214m=0.946m
0.947m+0.214m=1.16m
also at 0.518m−0.214m=0.303m
0.303m−0.214m=0.089m
The standing wave exists only along the line segment between the speakers . No nodes or antinodes appear at distances greater than 1.252m or less than 0, because waves add to gives standing wave only if they are travelling in opposite directions and not in the same direction . Thus , the distances from either of the speakers to the nodes of pressure between the speakers are 0.089m,0.303m,0.732m,0.946mand1.16m
Similar Questions
The distance between the two identical speakers shown in Fig. 14.3.3 are 4.00m. They are driven by the same osicllator. Initially a listner stays at L at a distance 8.0 m from the centre of the line connecting the two speakers. The listner then walks to the point A where AL=0.4m. The first minium is sound intensity in heard just the listener reaches the point A. Find the frequency of the oscillator. Speed of sound in air is 340 m/s.
View SolutionFind out the points on the line joining two charges +qand−3q(kept at as distance of 1.0m) where electric potential is zero.
View SolutionTwo identical loudspeakers are placed on a line at distance 4m and 6m from a microphone lying on the line joining them. The output of each speaker at the microphone is 2mW. If the two speakers are connected in series and excited by the same oscillator and they vibrate in phase with each other, the resultant ouput of the microphone will be
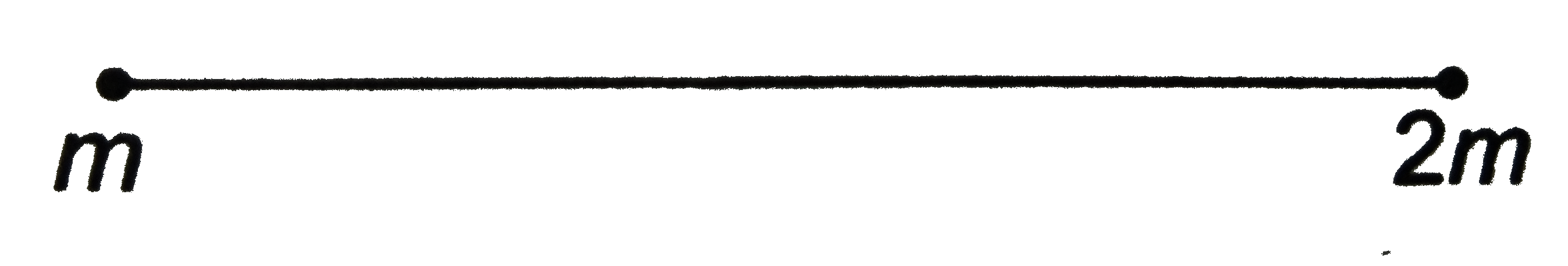
(frequency of oscillator =660Hz and speed of sound =330m/s)View SolutionTwo points masses 'm' and 2m are kept at certain distance as shows in figure. Draw E−r and V−r graphs along the line joining them corresponding to given mass system.
 View Solution
View SolutionTwo factories are sounding their sirens at 800 Hz. A man goes from one factory to the other at a speed of 2 m/s. The velocity of sound is 320 m/s. The number of beats heard by the person is 1 s will be
View SolutionA and B are two identical sound sources cachemitting a note of frequency 1000 Hz. A person moves from A to B along the line joining the two sources. How fast should be move to that he has 7 beats per second. velocity of sound 330 m/s ?
View SolutionTwo identical loudspeakers are located at point A & B,2m apart. The loudspeakers are driven by the same amplifier (coherent and are in phase). A small detector is moved out from point B along a line frequency below which there will be no position
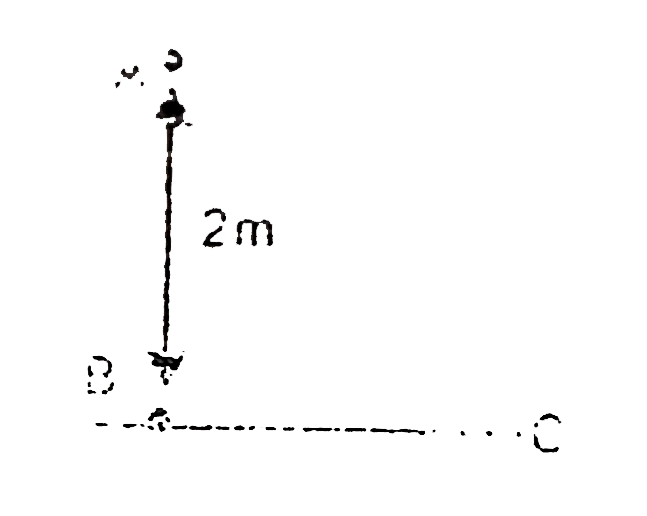 View Solution
View SolutionAn oscillator of frequency 680Hz drives two speakers . The speakers are fixed on a vertical pole at a distance 3m from each other as shown in Fig. 7.103. A person whose height is almost the same as that of the lower speaker walks towards the lower speaker in a direction perpendicular to the pole. Assuming that there is no reflection of sound from the ground and speed of sound is v=340m/s , answer the following questions.

At some instant , when the person is at a distance 4m from the pole , the wave function ( at the person's location) that describes the waves coming from the lower speaker y=Acos(kx−ωt) , where A is the amplitude , ω=2πv with v=680Hz (given) and k=2π/λ
Wave function ( at the person's location) that describes waves coming from the upper speaker can be expressed as :View SolutionTwo loudspeakers radiate in phase at 170HZ. An observer sits at 8m from one speaker and 11m from the other . The intensity level from either speaker acting alone is 60dB. The speed of sound is 340m/s. Find the observer intensity when both speakers are on together.
View SolutionAn oscillator of frequency 680Hz drives two speakers . The speakers are fixed on a vertical pole at a distance 3m from each other as shown in Fig. 7.103. A person whose height is almost the same as that of the lower speaker walks towards the lower speaker in a direction perpendicular to the pole. Assuming that there is no reflection of sound from the ground and speed of sound is v=340m/s , answer the following questions.

How far is the person from the pole when he hears a minimum in sound intensity a second time ?View Solution

