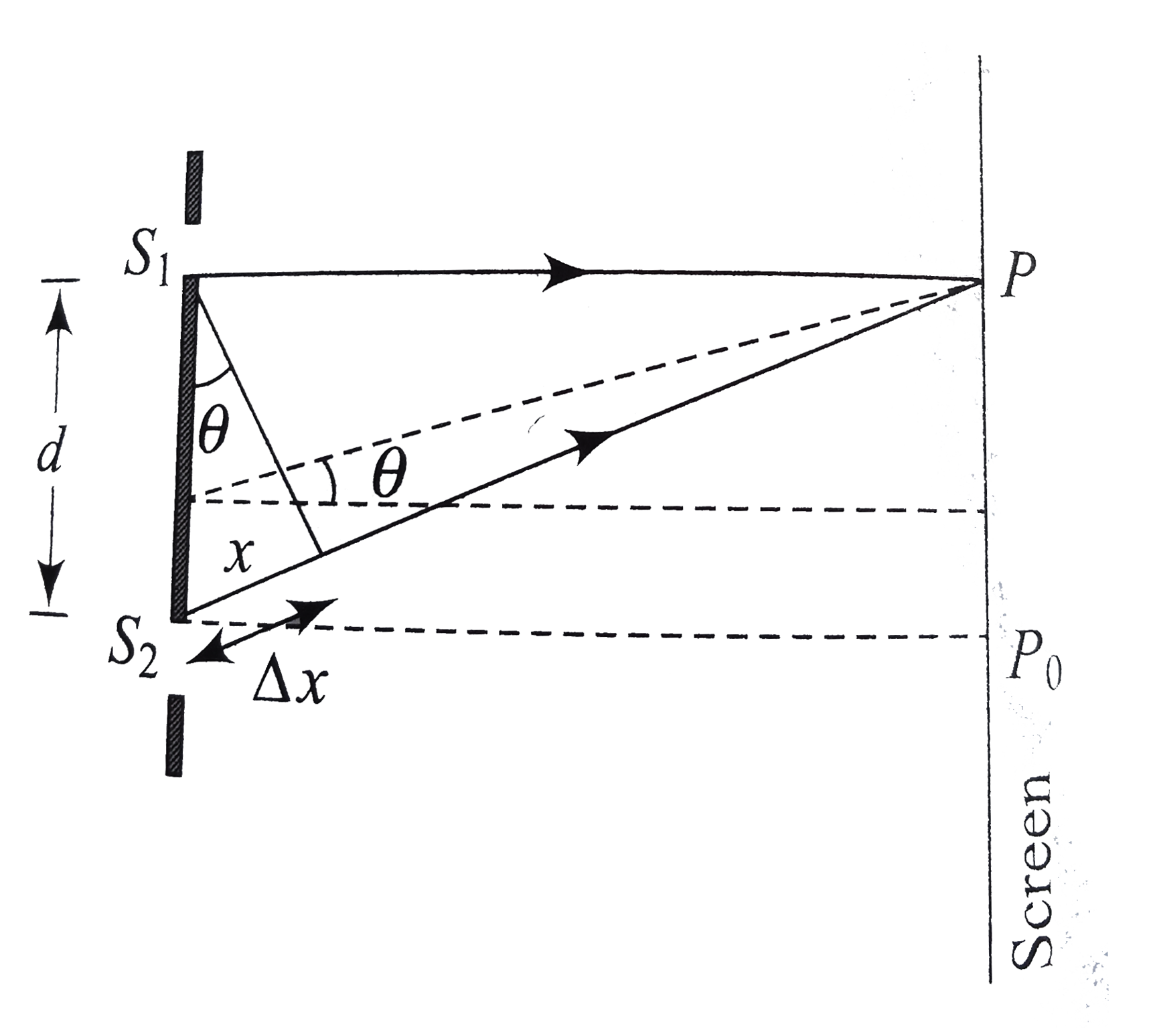
Two identical sources each of intensity $I_{0}$ have a separation $d = λ / 8$ , where $λ$ is the wavelength of the waves emitted by either source. The phase difference of the sources is $π / 4$ The intensity distribution $I (θ)$ in the radiation field as a function of $θ$ Which specifies the direction from the sources to the distant observation point P is given by

A

$I (θ) = I_{0} {cos}^{2} θ$

B

$I (θ) = \frac{I_{0}}{4} {cos}^{2} (\frac{π θ}{8})$

C

$I (θ) = 4 I_{0} {cos}^{2} [\frac{π}{8} (sin θ + 1)]$

D

$I (θ) = I_{0} {sin}^{2} θ$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:c

$Δ x = sin θ = \frac{λ}{8} sin θ$
$Δ ϕ =$ phase difference at P
$Δ ϕ = \frac{2 π}{λ} (Δ x) + Δ ϕ' = \frac{2 π}{λ} (\frac{λ}{8} sin θ) + \frac{π}{4}$
$I (θ) = 4 I_{0} {cos}^{2} (\frac{Δ ϕ}{2})$
$= 4 I_{0} {cos}^{2} [\frac{π}{8} (1 + sin θ)]$

|

Updated on:21/07/2023

Class 12 PHYSICS WAVE OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two identical radiators have a separation of $d = λ / 4$ where $λ$ is the wavelength of the waves emitted by either source. The initial phase difference between the sources is $λ / 4$ . Then the intensity on the screen at a distant point situated at an angle $θ = 30^{\circ}$ from the radiators is (here $I_{0}$ is intensity at that point due to one radiator alone)
A(a) $I_{0}$
B(b) $2 I_{0}$
C(c) $3 I_{0}$
D(d) $4 I_{0}$
Question 2 - Select One
Two sources of intensity 2I and 8I are used in an interference experiment. The intensity at a point where the waves from two sources superimpose with a phase difference of (a) zero (b) $π / 2 and (c) π$ is
A $18 I, 10 I, 2 I$
B $5 I, 4 I, I$
C $2 I, I, \frac{I}{2}$
D $2 I, 10 I, 18 I$
Question 3 - Select One
In Young's double slit experiment, the constant phase difference between two source is $\frac{π}{2}$ . The intensity at a point equidistant from theslits in terms of maximum intensity $I_{0}$ is
A $I_{0}$
B $I_{0} / 2$
C $3 I_{0} / 4$
D $3 I_{0}$

CENGAGE PHYSICS-WAVE OPTICS-Single Correct

Home
Ask Doubt
Profile