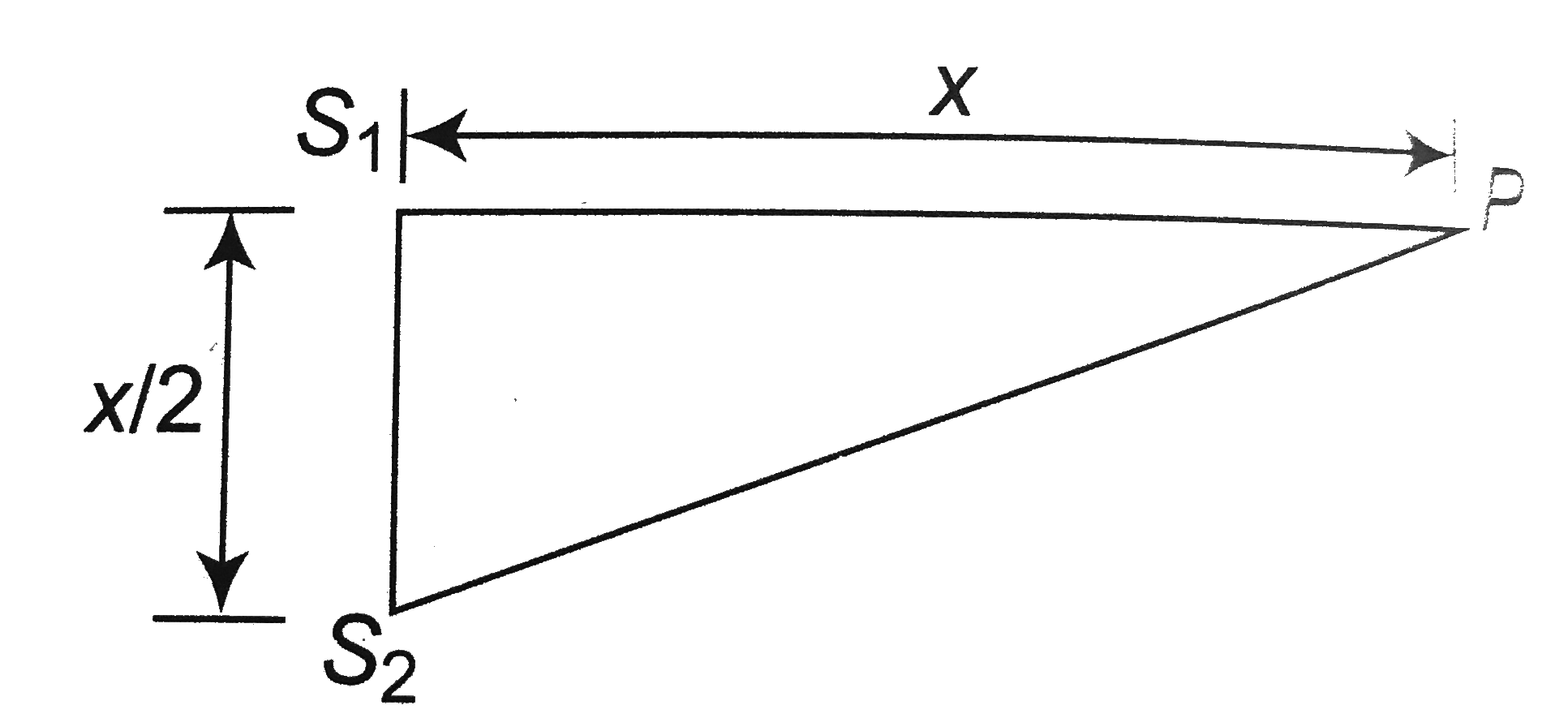
The wavelength of the waves arriving at P from two coherent sources $S_{1}$ and $S_{2}$ is 4m, while intensity of each wave is $I_{0}$ . The resultant intensity at $P$ is $2 I_{0}$ . Find the minimum value of $S_{2} P$

$\frac{x \sqrt{5}}{2}$

$2 x$

$(x + 1)$

$(x + \sqrt{2})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Updated on:21/07/2023

The wavelength of the waves arriving at P from two coherent sources $S_{1}$ and $S_{2}$ is 4m, while intensity of each wave is $I_{0}$ . The resultant intensity at $P$ is $2 I_{0}$ . Find the minimum value of $S_{2} P$
A $\frac{x \sqrt{5}}{2}$
B $2 x$
C $(x + 1)$
D $(x + \s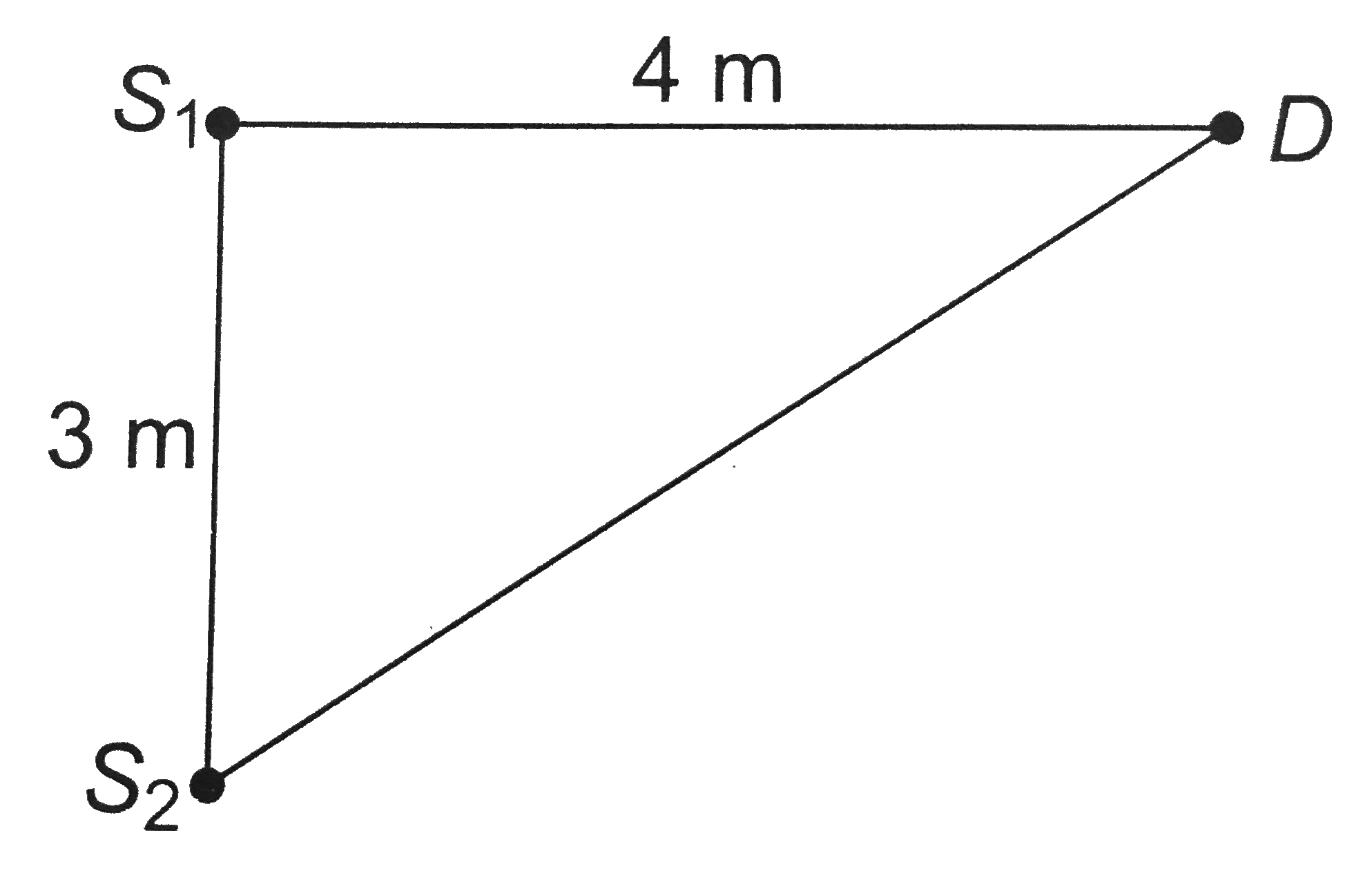qrt{2})$
In the figure the intensity of waves arriving at D from two coherent sources $S_{1}$ and $S_{2}$ is $I_{0}$ The wavelength of the wave is $λ = 4 m$ . Resultant intensity at D will be:
A $4 I_{0}$
B $I_{0}$
C $2 I_{0}$
Dzero
In the figure , the intensity of waves arriving at D from two coherent soucrces $s_{1} and s_{2} i s I_{0}$ . The wavelength of the wave is $λ = 4 m$ . Resultant intensity at D will be

A $4 l_{0}$
B $l_{0}$
C $2 l_{0}$
Dzero