Two lock gates of $7.5 m$ height are provided in a canal of $16 m$ width meeting at an angle of $120^{\circ}$ . Calculate the force acting on each gate when the depth of water in upstream side is $5 m$ .

A

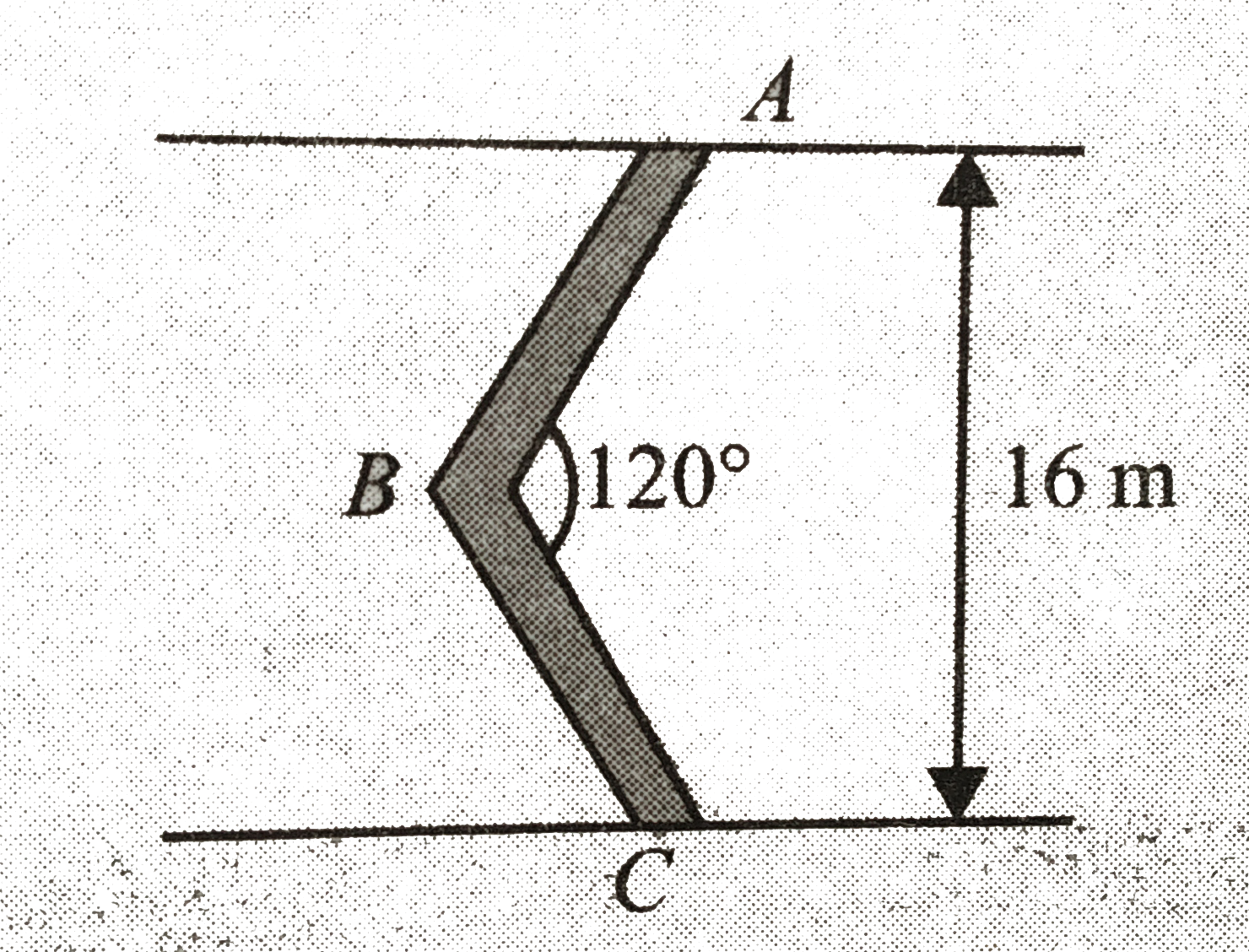
$1133 k N$

B

$1200 k N$

C

$578 k N$

D

$1566 k N$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Height of lock gates $= 7.5 m$
Width of canal $= 16 m$
Inclination of gates $= 120^{\circ}$ and $H = 5 m$
From the geometry of the lock gates, we find that inclination of the lock gates with the walls
$a = \frac{180^{\circ} - 120^{\circ}}{2} = 30^{\circ}$
and width of each gate $= \frac{\frac{16}{2}}{cos α} = \frac{8}{{cos 30}^{\circ}} = \frac{8}{0.866} = 9.24 m$
Therefore, wetted area of each gate
$A = 5 \times 9.24 = 46.2 m^{2}$
and force acting on each gate is
$F = g A \times (\frac{H}{2}) = 9.81 \times 46.2 \times (\frac{5}{2}) = 1133 k N$

|

Updated on:21/07/2023

Class 11 PHYSICS FLUID MECHANICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A water tank has the shape of an inverted right circular cone, whose semi-vertical angle is ${tan}^{- 1} (\frac{1}{2})$ . Water is poured into it at a constant rate of 5 cu m/min. Then, the rate (in m/min) at which the level of water is rising at the instant when the depth of water in the tank is 10 m is
A $\frac{2}{π}$
B $\frac{1}{5 π}$
C $\frac{1}{15 π}$
D $\frac{1}{10 π}$
Question 2 - Select One
Two forces each of magnitude $10 N$ act simultaneously on a body with their directions inclined to each other at an angle of $120^{\circ}$ and displaces the body over $10 m$ along the bisector of the angle between the two forces. Then the work done by force is
A5 J
B1 J
C50 J
D100 J
Question 3 - Select One
There are two charges +1 $μ C$ and $+ 5 μ C$ , separated by a distance of 0.2 m in air, the ratio of forces acting in them will be:
A $1 : 5$
B $5 : 1$
C $1 : 1$
D $1 : 25$

CENGAGE PHYSICS-FLUID MECHANICS-Exercise 4.1

Home
Ask Doubt
Profile