Two long wires PQR and MNP carry equal current Ias shown such that QR and NP are parallel. Find the magnetic field at origin O.

μ0I2√3πa into the page
μ0I2πa out of the page
μ0I2√3πa out of the page
μ0I2πa into the page
The correct Answer is:C
Due to PQ and MN, magnetic field at O will be zero.

r=asin60∘=√32a
BQR=μ04πIr[sin90∘−sin30∘]⊗
=μ04πI(√3a/2)×12⊗=μ0I4π√3a⊗
BNP=μ04πIr[sin90∘+sin30∘]⊙=3μ0I4π√3a⊙
Net field at O:
B=BNP−BQR=μ0I2√3πa⊙
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Magnetic Field Due To Circular Arc69videos
SOURCE AND EFFECT OF MAGNETIC FIELD
- BOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Problems Based On Mixed Concepts32videos
SOURCE AND EFFECT OF MAGNETIC FIELD
- BOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Magnetic Dipole32videos
SOURCE AND EFFECT OF MAGNETIC FIELD
- BOOK - A2ZCHAPTER - SEMICONDUCTOR ELECTRONICSEXERCISE - EXERCISE29videos
SEMICONDUCTOR ELECTRONICS
- BOOK - A2ZCHAPTER - WAVE OPTICSEXERCISE - Section D - Chapter End Test29videos
WAVE OPTICS
Similar Questions
A conducting circular loop of radius a is connected to two long, straight wires . The straight wires carry a current I as shown in figure . Find the magnetic field B at the centre of the loop.
 View Solution
View SolutionTwo parallel wires carry equal currents of 10A along the same direction and are separated by a distance of 2.0 cm. Find the magnetic field at a point which is 2.0 cm away from each of these wires.
View Solution
Knowledge Check
- Question 1 - Select One
Two long wires PQR and MNP carry equal current Ias shown such that QR and NP are parallel. Find the magnetic field at origin O.
 Aμ0I2√3πa into the pageBμ0I2πa out of the pageCμ0I2√3πa out of the pageDμ0I2πa into the page
Aμ0I2√3πa into the pageBμ0I2πa out of the pageCμ0I2√3πa out of the pageDμ0I2πa into the page - Question 2 - Select One
Two long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
Aμ0idπ(d2+x2)Bμ0ixπ(d2−x2)Cμ0ix(d2+x2)Dμ0id(d2+x2) - Question 3 - Select One
Two long parallel wires carry equal current I flowing in the same direction are at a distance 2d apart. The magnetic field B at a point lying on the perpendicular line joining the wires and at a distance x from the midpoint is
Aμ0idπ(d2+x2)Bμ0ixπ(d2−x2)Cμ0ix(d2+x2)Dμ0id(d2+x2)
(a) Two long wires at a distance 2d apart carry equal, antiparallel current i. Find magnitude and direction of magnetic field at point A as shown.For what value of x, magnetic field is maximum? Also calculate maximum magnetic field. Sketch B versus x graph.
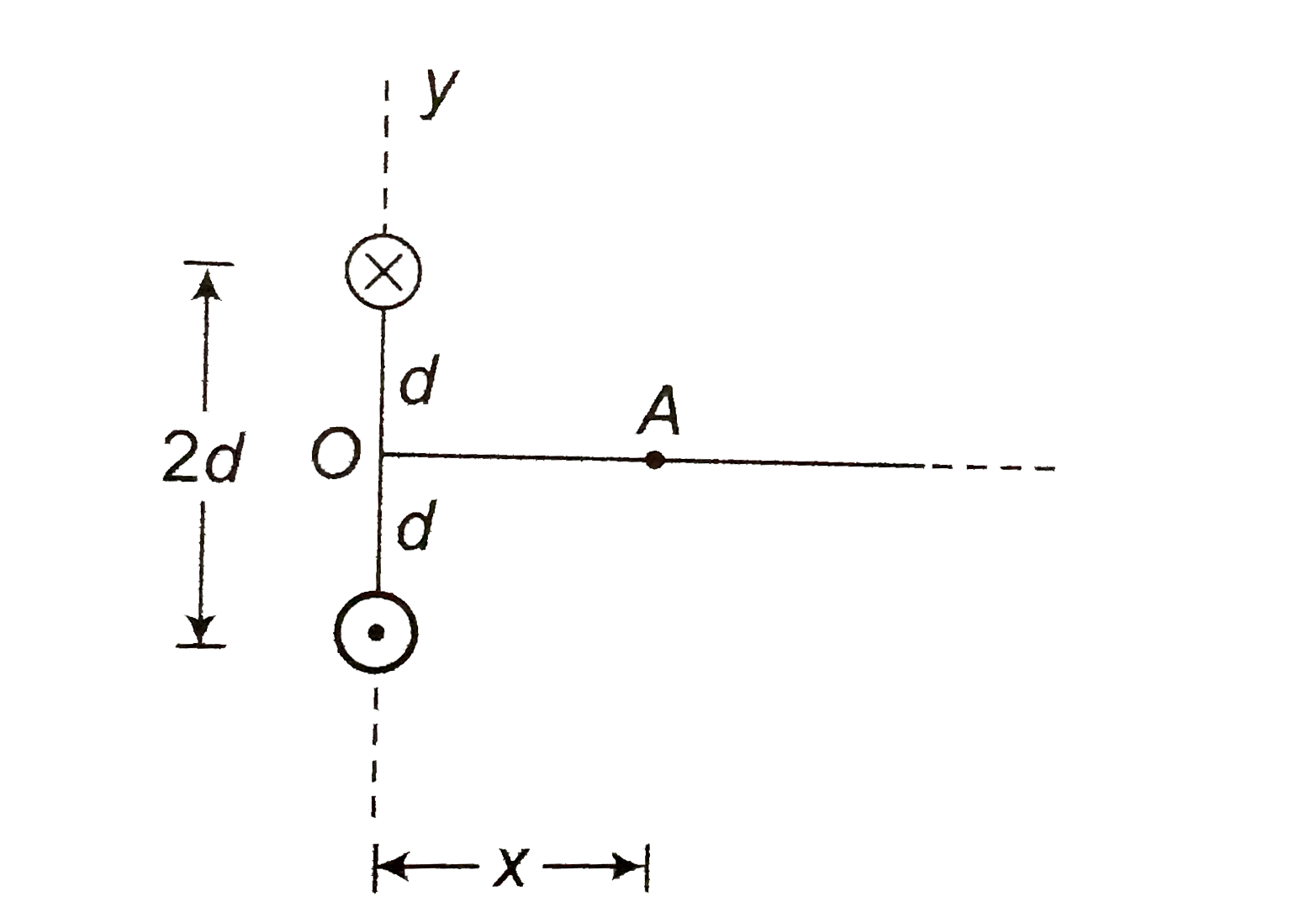 View Solution
View SolutionTwo long thin wires ABC and DEF are arranged as shown in Fig. They carry equal currents I as shown. The magnitude of the magnetic field at O is
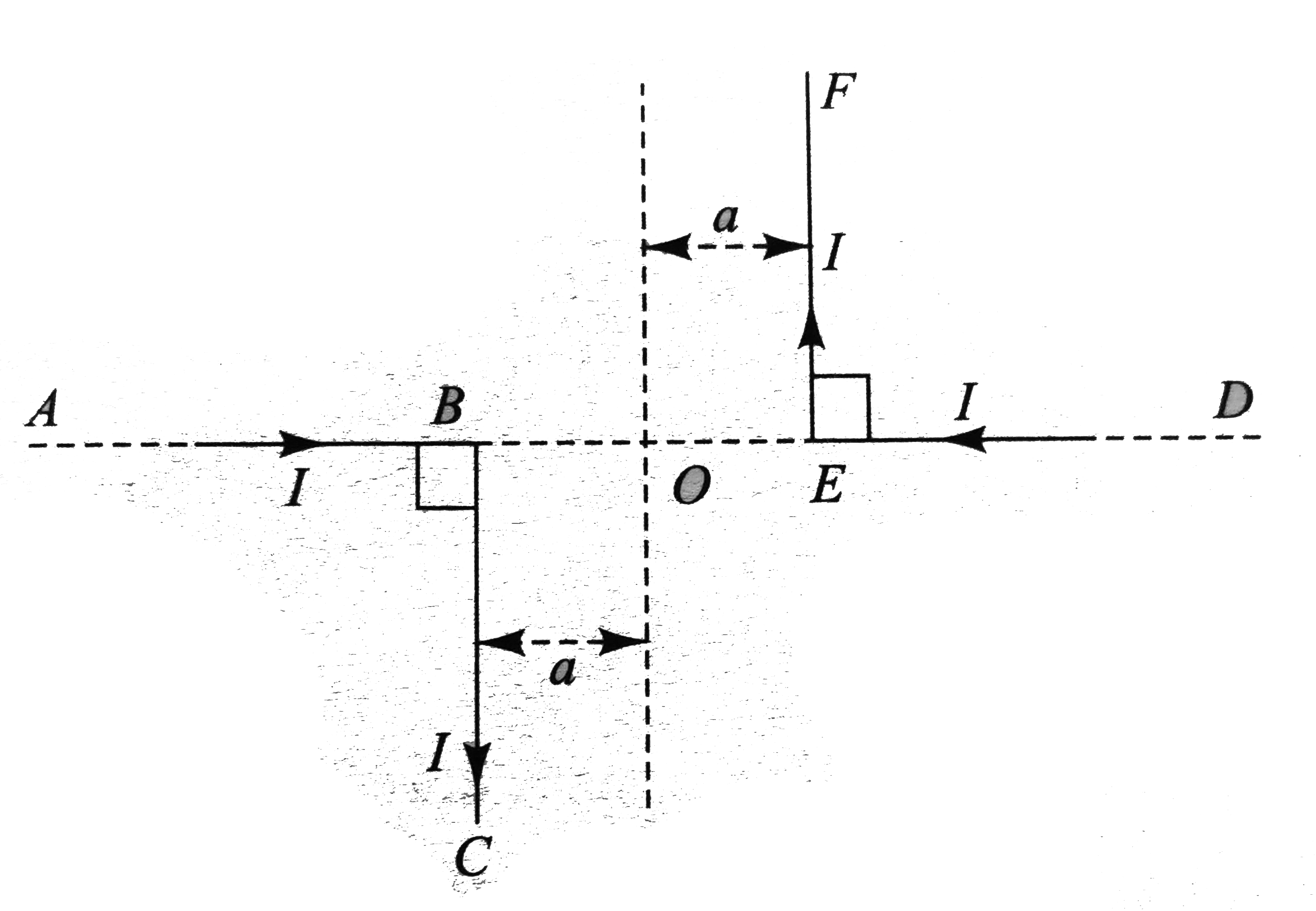 View Solution
View SolutionTwo long thin wires ABC and DEF are arranged as shown in the figure. They carry currents I. The magnitude of the magnetic field at O is
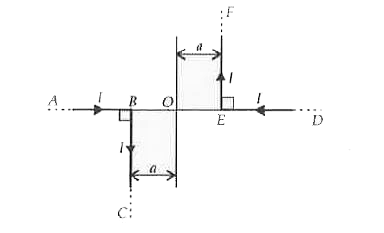 View Solution
View SolutionShown in the figure is a conductor carrying a current i. the magnetic field at the origin is :
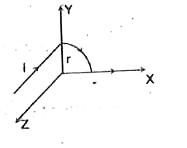 View Solution
View SolutionTwo very thin metallic wires placed X and Y-axis carry equal currents as shown here. AB and CD are lines at 45∘ with the axes with origin of axes at O. The magnetic field with be zero on the line
 View Solution
View Solution

