Two objects of masses and are moving along the same line in the same direction with velocities of and , respectively. They collide and after the collison, the first object moves at a velocity of in the same direction. Determine the velocity of the second object.
To solve the problem, we will use the principle of conservation of linear momentum. The momentum before the collision is equal to the momentum after the collision.
1. Identify the Given Data:
- Mass of the first object (m1) = 100 g = 0.1 kg (since 100 g = 100/1000 kg)
- Mass of the second object (m2) = 200 g = 0.2 kg (since 200 g = 200/1000 kg)
- Initial velocity of the first object (u1) = 2 m/s
- Initial velocity of the second object (u2) = 1 m/s
- Final velocity of the first object after collision (v1) = 1.67 m/s
- Final velocity of the second object after collision (v2) = ?
2. Apply the Conservation of Linear Momentum:
The principle of conservation of momentum states that:
m1u1+m2u2=m1v1+m2v2
Substituting the known values:
(0.1kg×2m/s)+(0.2kg×1m/s)=(0.1kg×1.67m/s)+(0.2kg×v2)
3. Calculate the Left Side of the Equation:
0.1×2=0.2kg m/s
0.2×1=0.2kg m/s
Therefore, the left side becomes:
0.2+0.2=0.4kg m/s
4. Calculate the Right Side of the Equation:
0.1×1.67=0.167kg m/s
So, the right side becomes:
0.167+0.2v2
5. Set the Left Side Equal to the Right Side:
0.4=0.167+0.2v2
6. Isolate v2:
Subtract 0.167 from both sides:
0.4−0.167=0.2v2
0.233=0.2v2
Now, divide both sides by 0.2:
v2=0.2330.2=1.165m/s
7. Final Result:
The velocity of the second object after the collision is approximately:
v2≈1.17m/s
- Mass of the first object (m1) = 100 g = 0.1 kg (since 100 g = 100/1000 kg)
- Mass of the second object (m2) = 200 g = 0.2 kg (since 200 g = 200/1000 kg)
- Initial velocity of the first object (u1) = 2 m/s
- Initial velocity of the second object (u2) = 1 m/s
- Final velocity of the first object after collision (v1) = 1.67 m/s
- Final velocity of the second object after collision (v2) = ?
The principle of conservation of momentum states that:
m1u1+m2u2=m1v1+m2v2
Substituting the known values:
(0.1kg×2m/s)+(0.2kg×1m/s)=(0.1kg×1.67m/s)+(0.2kg×v2)
0.1×2=0.2kg m/s
0.2×1=0.2kg m/s
Therefore, the left side becomes:
0.2+0.2=0.4kg m/s
0.1×1.67=0.167kg m/s
So, the right side becomes:
0.167+0.2v2
0.4=0.167+0.2v2
Subtract 0.167 from both sides:
0.4−0.167=0.2v2
0.233=0.2v2
Now, divide both sides by 0.2:
v2=0.2330.2=1.165m/s
The velocity of the second object after the collision is approximately:
v2≈1.17m/s
Topper's Solved these Questions
Similar Questions
Two equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
View SolutionTwo equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
View SolutionTwo elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
View SolutionTwo particles of mass 1 kg and 0.5 kg are moving in the same direction with speed of 2m/s and 6 m/s respectively on a smooth horizontal surface. The speed of centre of mass of the system is
View SolutionTwo particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo particles of mass 1kg and 2kg are moving along the same line with speeds 2m/s and 4m/s respectively. Calculate the speed of the centre of mass of the system if both the particles are moving in the same direction.
View SolutionTwo perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
View SolutionTwo perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
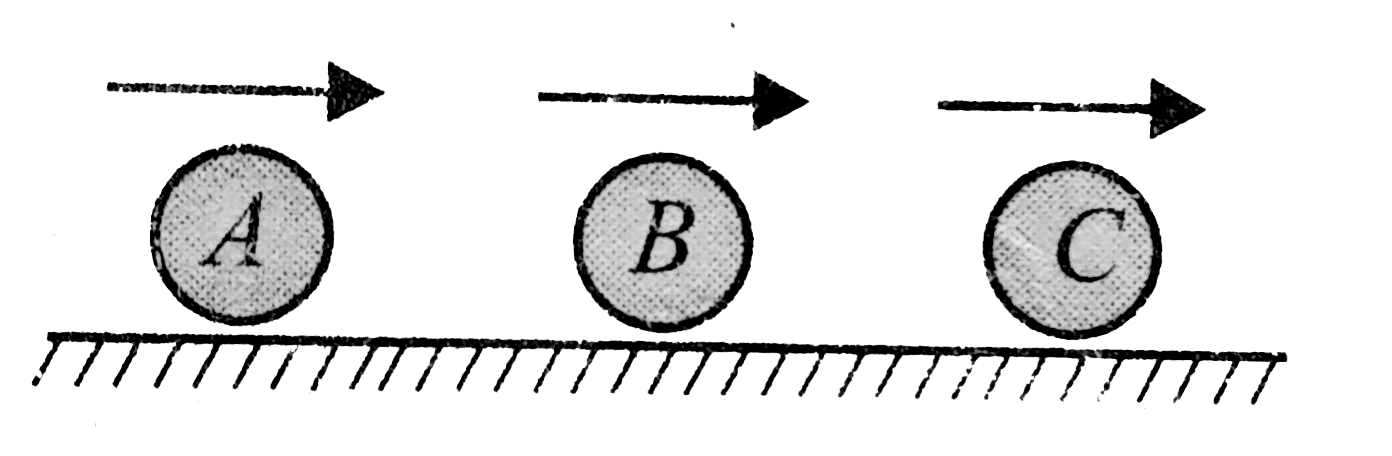
View SolutionThree balls A,B and C of masses 2kg,4kg and 8kg, respectively, move along the same straight line and in the same direction, with velocities 4m/s,1m/s,34m/s. If A collides with B and subse-quently B collides with C, find the velocity of ball A and ball B after collision, taking the coefficient of restitution as unity.
 View Solution
View SolutionTwo objects each of mass 5 kg are moving in the same straight line but in the opposite direction towards each other with some speed of 3 m/s. They stick to each other after collision. What will be the velocity of the combined object after collision?
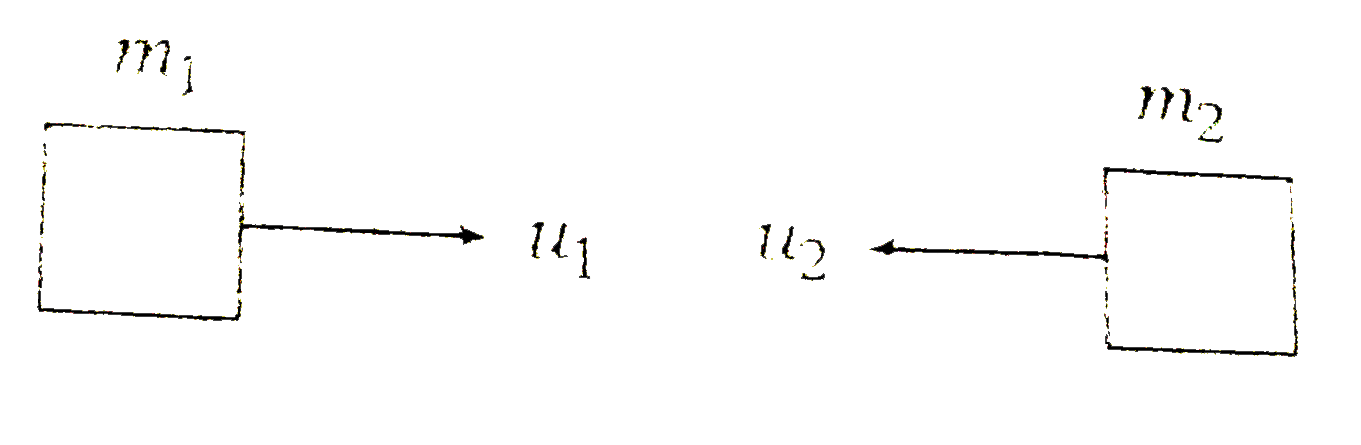 View Solution
View Solution

