Two perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
0, 25
3, 20
10, 15
20, 5
The correct Answer is:C
To solve the problem of finding the velocities of two perfectly elastic particles A and B after they collide, we can follow these steps:
1. Identify the Given Information:
- Mass of particle A (m_A) = m (let's assume mass = m for simplicity)
- Mass of particle B (m_B) = m
- Initial velocity of particle A (u_A) = 15 m/s
- Initial velocity of particle B (u_B) = 10 m/s
2. Understand the Nature of the Collision:
- The collision is perfectly elastic, which means both momentum and kinetic energy are conserved.
- Since the masses are equal, we can use the property of elastic collisions for equal masses.
3. Apply the Elastic Collision Formula:
- For two objects of equal mass, the velocities after the collision can be determined by simply exchanging their velocities.
- Therefore, after the collision:
- Final velocity of particle A (v_A) = u_B = 10 m/s
- Final velocity of particle B (v_B) = u_A = 15 m/s
4. State the Final Velocities:
- The final velocities of the particles after the collision are:
- v_A = 10 m/s (for particle A)
- v_B = 15 m/s (for particle B)
5. Conclusion:
- The velocities of particles A and B after the collision are 10 m/s and 15 m/s, respectively.
Final Answer:
The velocities after the elastic collision will be:
- Particle A: 10 m/s
- Particle B: 15 m/s
- Mass of particle A (m_A) = m (let's assume mass = m for simplicity)
- Mass of particle B (m_B) = m
- Initial velocity of particle A (u_A) = 15 m/s
- Initial velocity of particle B (u_B) = 10 m/s
- The collision is perfectly elastic, which means both momentum and kinetic energy are conserved.
- Since the masses are equal, we can use the property of elastic collisions for equal masses.
- For two objects of equal mass, the velocities after the collision can be determined by simply exchanging their velocities.
- Therefore, after the collision:
- Final velocity of particle A (v_A) = u_B = 10 m/s
- Final velocity of particle B (v_B) = u_A = 15 m/s
- The final velocities of the particles after the collision are:
- v_A = 10 m/s (for particle A)
- v_B = 15 m/s (for particle B)
- The velocities of particles A and B after the collision are 10 m/s and 15 m/s, respectively.
The velocities after the elastic collision will be:
- Particle A: 10 m/s
- Particle B: 15 m/s
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - B)
35 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - C)
80 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - EXERCISE
37 Videos
- WAVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Two elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
View SolutionTwo equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
View Solution
Knowledge Check
Two identical balls A and B moving with velocities + 0.5 m/s and −0.3.m/s respectively collide head-on elastically. The velocities of the ball A and B after collision will be respectively
A+0.5m/sand+0.3m/sB−0.3m/sand+0.5m/sC + 0.3 m//s and + 0.5 m//sD−0.5m/sand+0.3m/s
Two equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
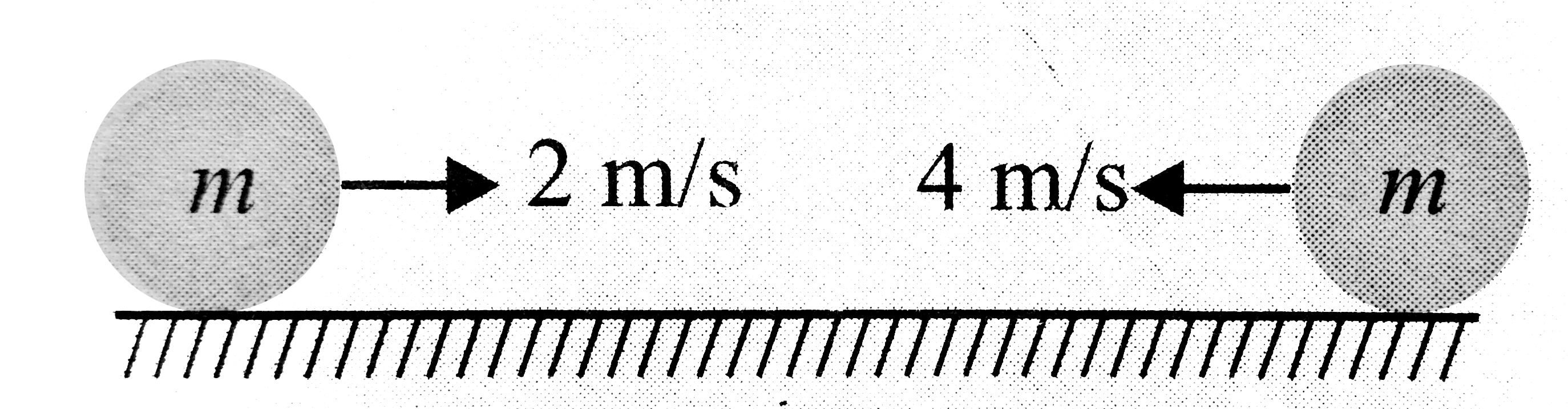
View SolutionTwo identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
View SolutionTwo identical balls AandB having velocity of 0.5m/sand−0.3m/s respectively collide elastically in one dimension. The velocities of BandA after the collision respectively will be
View SolutionTwo identiacal balls A and B having velocities of 0.5 m/s and 0.3 m/s respectively collide elastically in one dimension. The velocities of B and A after the collision respectively will be
View SolutionTwo particles of masses m and 2m moving in opposite directions collide elastically with velocity 2v and v, respectiely. Find their velocities after collision.

View SolutionTwo objects of masses 100g and 200g are moving along the same line in the same direction with velocities of 2m/s and 1m/s, respectively. They collide and after the collison, the first object moves at a velocity of 1.67m/s in the same direction. Determine the velocity of the second object.
View SolutionTwo balls of masses m each are moving at right angle to each other with velocities 6 m/s and 8 m/s respectively. If collision between them is perfectly inelastic, the velocity of combined mass is
View Solution
