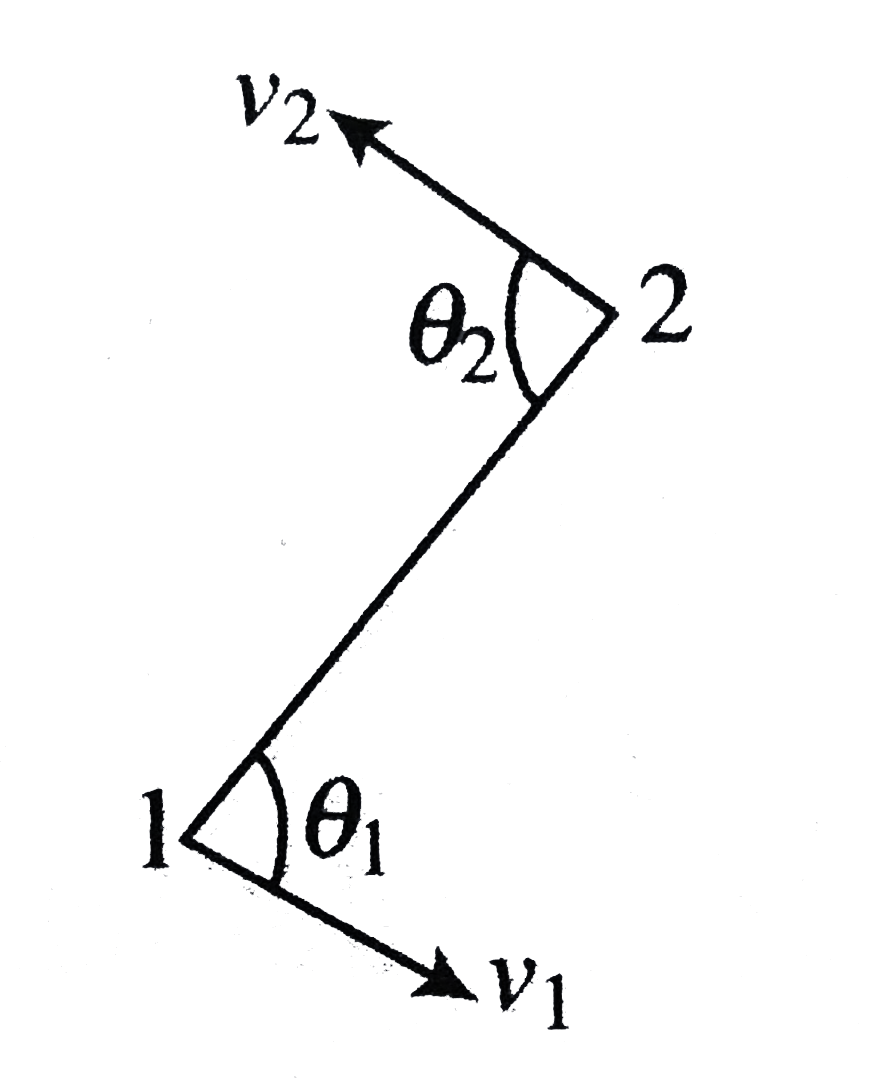
Two particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
Let us find the relative velocity between the particles perpendicular to the line joining them, the velocity of 1 relative to velocity of 2 is
→v12=→v1−→v2
Then, →v12y=→v1y−→v2y=−v1sinθ1ˆj−v2sinθ2ˆj
Hence, ω12=∣∣→v12y∣∣l
Substituting ∣∣→v12y∣∣, we have
ω12=v1sinθ1+v2sinθ2l.
 ,
, .
.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Subjective40videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Single Correct76videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.312videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer9videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression2videos
KINETIC THEORY OF GASES
Similar Questions
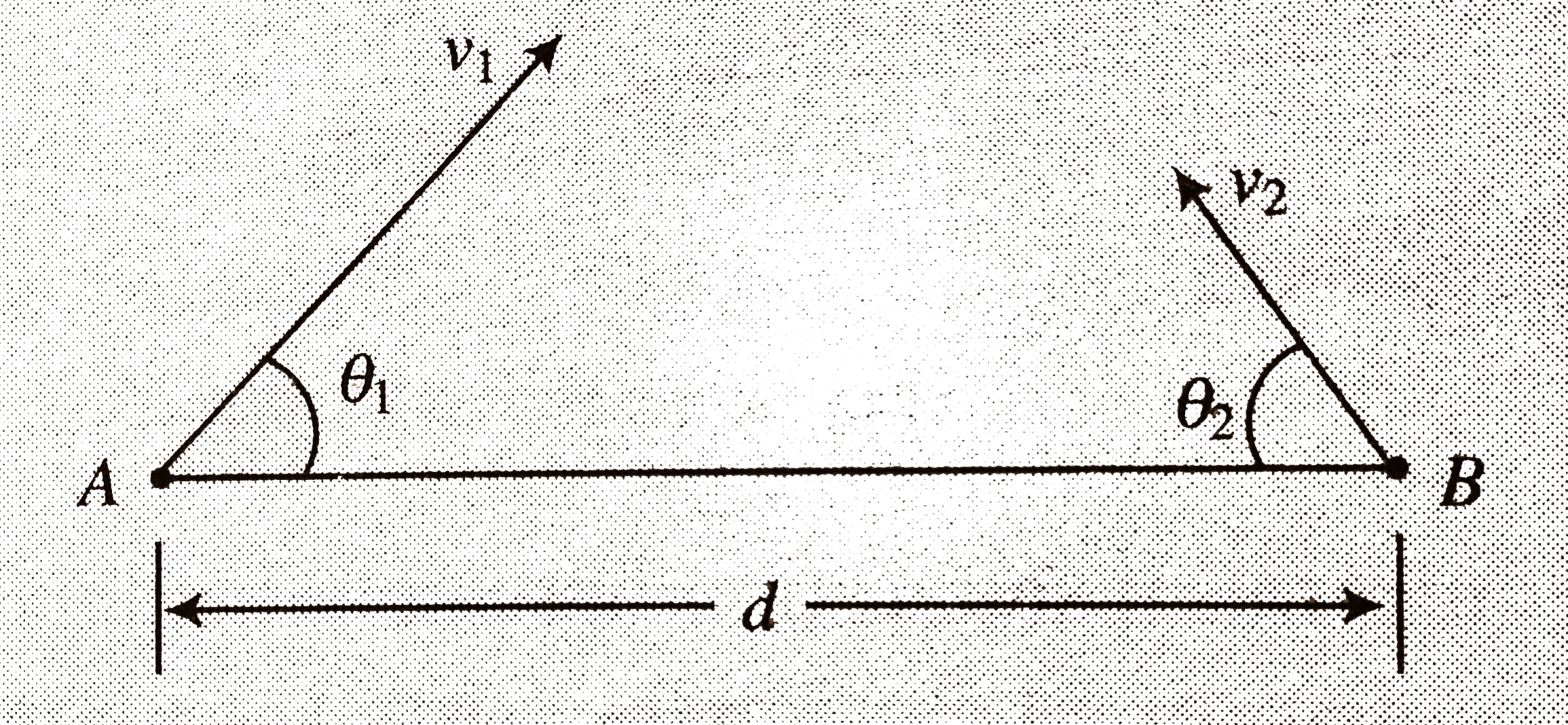
Two particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandB and v2 makes an angle θ2 with the line joining AandB. Find their velocity of approach.
 .View Solution
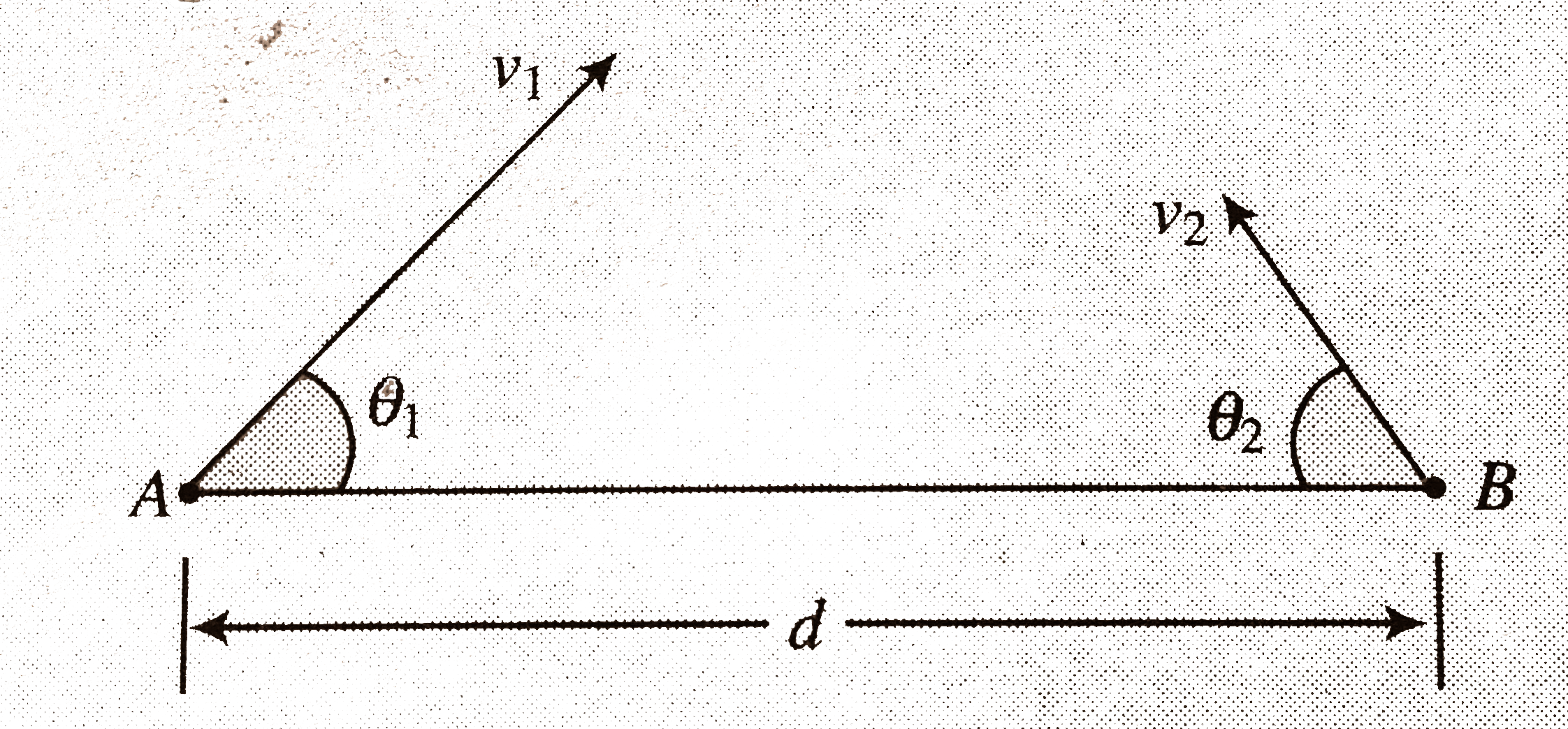
.View SolutionTwo particles AandB are moving with constant velocities v1andv2. At t=0, v1 makes an angle θ0 with the line joining AandBandv2 makes an angle θ2 with the line joining AandB.
(a) Find the condition for AandB to collide.
(b) Find the time after which AandB will collide if separation between them is datt=0.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One or More
If two bodies are in motion with velocity →v1 and →v2:
A|vrel|=√v21+v22B|→–––(rel)|=v1±v2∣Cvre=0Dvrel>c (speed of light) - Question 2 - Select One
Two particle A and B, move with constant velocities →v1and→v2. At the initial moment their position vectors are →r1 and →r2 respectively . The condition for particles A and B for their collision is
A→r1×→v1=→r2×→v2B→r1−→r2=→v1−→v2C→r1−→r2∣∣→r1−→r2∣∣=→v2−→v1∣∣→v2−→v1∣∣D→r1.→v1=→r2.→v2 - Question 3 - Select One
Two particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
AzeroBπ/4Cπ/2Dπ
Two particles are projected from the same point making angles, angle θ1 and θ2 with the horizontal respectively in such a way that their horizontal velocities are equal. The ratio of maximum heights (H1H2) will be equal to
View SolutionTwo particles, 1 and 2, move with constant velocities v1 and v2. At the initial moment their radius vectors are equal to r1 and r2. How must these four vectors be interrelated for the particles to collide?
View SolutionTwo particles A and B, move with constant velocities →v1 and →v2. At the initial moment their position vectors are →r1 and →r2 respectively. The condition for particle A and B for their collision is
View SolutionTwo point particles with masses m1andm2 are thrown at angles θ1andθ2 with horizontal with speeds v1andv2 respectively. R, H and T are range, height and total time of flight respectively. Let v1sinθ1=v2sinθ2. Then for both particles
View SolutionTwo balls are projected simultaneously in the same vertical plane from the same point with velocities v1 and v2 with angle θ1 and θ2 respectively with the horizontal. If v1 cos θ1=v2cosθ2, the path of one ball as seen from the position of other ball is :
View Solution

