Two particles of equal mass are connected to a rope AB of negligible mass, such that one is at end A and the other dividing the length of the rope in the ratio 1:2 from A.The rope is rotated about end B is a horizontal plane. Ratio of the tensions in the smaller part to the other is (ignore effect of gravity)
4:3
1:4
1:2
1:3
The correct Answer is:D
To solve the problem, we need to analyze the forces acting on the two masses connected by the rope and derive the ratio of the tensions in the two segments of the rope.
1. Understanding the Setup:
- We have two particles of equal mass m.
- The rope AB is divided into two parts: one part (AC) is L3 and the other part (CB) is 2L3.
- The rope is rotated about point B in a horizontal plane.
2. Identifying the Lengths:
- Let the total length of the rope L.
- The length of segment AC (the shorter part) is L3.
- The length of segment CB (the longer part) is 2L3.
3. Centripetal Force for Mass at C:
- For the mass at point C (which is at distance 2L3 from point B), the centripetal force required is provided by the tension T1 in the rope segment CB.
- The equation for the centripetal force is:
T1=mω2(2L3)
4. Centripetal Force for Mass at A:
- For the mass at point A (which is at distance L3 from point B), the centripetal force required is provided by the tension T2 in the rope segment AC.
- The equation for the centripetal force is:
T2=mω2(L3)
5. Finding the Ratio of Tensions:
- Now, we have:
T1=mω2(2L3)
T2=mω2(L3)
- To find the ratio T2T1:
T2T1=mω2(L3)mω2(2L3)=L32L3=12
6. Final Ratio:
- Therefore, the ratio of the tensions in the smaller part (T2) to the larger part (T1) is:
T2T1=12
Conclusion:
The ratio of the tensions in the smaller part to the larger part is 1:2.
- We have two particles of equal mass m.
- The rope AB is divided into two parts: one part (AC) is L3 and the other part (CB) is 2L3.
- The rope is rotated about point B in a horizontal plane.
- Let the total length of the rope L.
- The length of segment AC (the shorter part) is L3.
- The length of segment CB (the longer part) is 2L3.
- For the mass at point C (which is at distance 2L3 from point B), the centripetal force required is provided by the tension T1 in the rope segment CB.
- The equation for the centripetal force is:
T1=mω2(2L3)
- For the mass at point A (which is at distance L3 from point B), the centripetal force required is provided by the tension T2 in the rope segment AC.
- The equation for the centripetal force is:
T2=mω2(L3)
- Now, we have:
T1=mω2(2L3)
T2=mω2(L3)
- To find the ratio T2T1:
T2T1=mω2(L3)mω2(2L3)=L32L3=12
- Therefore, the ratio of the tensions in the smaller part (T2) to the larger part (T1) is:
T2T1=12
The ratio of the tensions in the smaller part to the larger part is 1:2.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.3
20 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.1
20 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
Two persons are holding a rope of negligible mass horizontally. A 20 kg mass is attached to the rope at the midpoint, as a result the rope deviates from the horizontal direction. The tension required to completely straighten the rope is (g=10m/s2)
View SolutionA straight rope of length 'L' is kept on a frictionless horizontal surface and a force 'F' is applied to one end of the rope in the direction of its length and away from that end. The tension in the rope at a distance 'I from that end is 9
View Solution
Knowledge Check
A straight rope of length 'L' is kept on a frictionless horizontal surface and a force 'F' is applied to one end of the rope in the direction of its length and away from that end. The tension in the rope at a distance 'l' from that end is
AFlBLFlC(1−lL)FD(1+lL)FA cart of mass M is tied to one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M. The entire system is on a smooth horizontal surface. The man is at x=0 and the cart at x=10m. If the man pulls the cart by the rope, the man and the cart will meet at the point
Athey will never meetBx=10mCx=5mDx=0In the figure a rope of mass m and length l is such that its one end is fixed to rigid wall and the other is applied with a horizontal force F as shown below, then tension at the middle of the string is:
 AFB2FCZeroDF/2
AFB2FCZeroDF/2
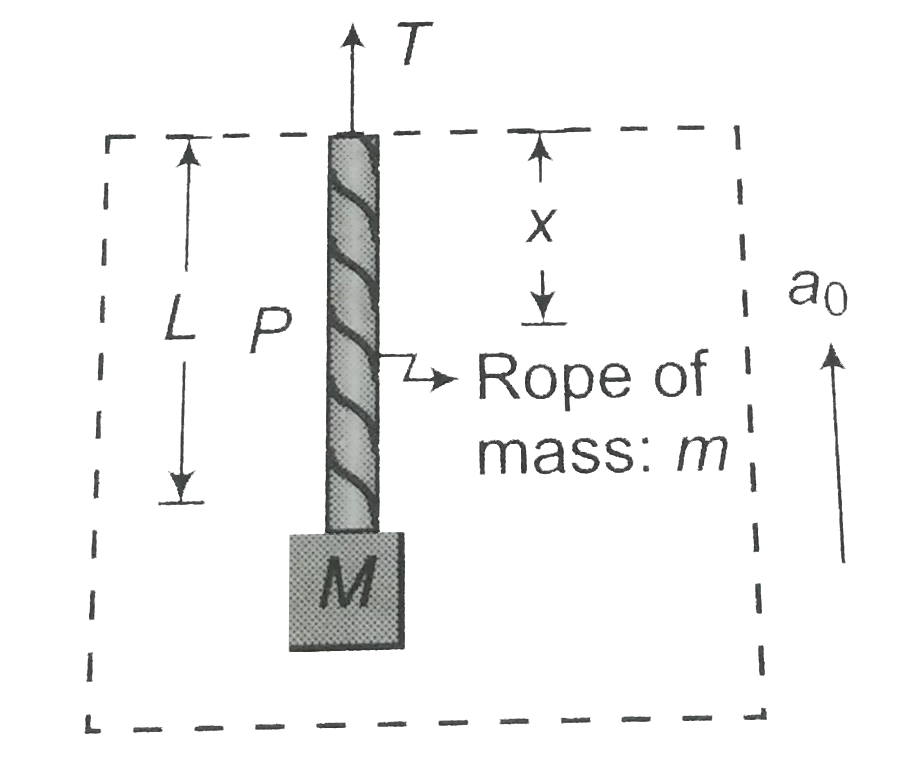
A uniform rope of mass m and length L is attached to the ceiling of a lift. The other end of the rope is attached to a block of mass M. The lift is moving up with a constant acceleration a0. Find the tension in the rope at a distance x from the ceiling as shown in the diagram.
View SolutionBlock A of mass m/2 is connected to one end of light rope which passes over a pulley as shown in fig. A man of mass m climbs the other end of rope with a relative acceleration of g/6 with respect to rope. Find the acceleration of block A and tension in the rope.

View SolutionA block of mass M is pulled along a horizontal frictionless surface by a rope of mass m as shown in A horizontal force F is applied to one end of the rope. Find
(a) the acceleration of the rope and block
(b) the force that the rope exerts on the block
(c) the tension in the rope at its mid point

View SolutionA rope of length 5 m is kept on frictionless surface and a force of 5 N is applied to one of its end. Find the tension in the rope at 1 m from this end
View SolutionA cart of mass M is tied to one end of a massless rope of length 10m. The other end of the rope is in the hands of a man of mass M2. The entire ststem is on a smooth horizontal surface. If the man pulls the cart by the rope then find the distance by which the man will slip on the horizontal surface before the man and the cart will meet each other.
View Solution