Two particles of masses 0.5 kg and 0.25kg moving with velocity 4.0 m/s and -3.0m/ collide head on in a perfectly inelastic collision. Find the velocity of the composite particle after collision and KE lost in the collision.
Here, m1=0.5kg,m2=0.25kg
u1=4.0m/s,u2=−3.0m/s
If v is velosity of composite particle, then from the principla of conservation of linear momentum,
(m1+m2)v=m1u1+m2u2
(0.5+0.25)v=0.5×4+0.25(−3.0)
v=2×0.750.75=1.250.75=1.67m/s
KE lost =12m1u21+12m2u22−12(m1+m2)v2
=12×0.5×42+12×0.25×(−3.0)2
−12(0.5+0.25)(53)2
=4+98−2524=9828=4.1J
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Conceptual Problems
27 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Very Short Anwer Question.
54 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Curiosity questions
2 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Two particles of mass 1 kg and 2 kg are moving with speed 2 m/s and 5 m/s as shoen in the figure, they collides perfectly inelastically. Find
(a) Their final velocity
(b) Loss in kinetic energy
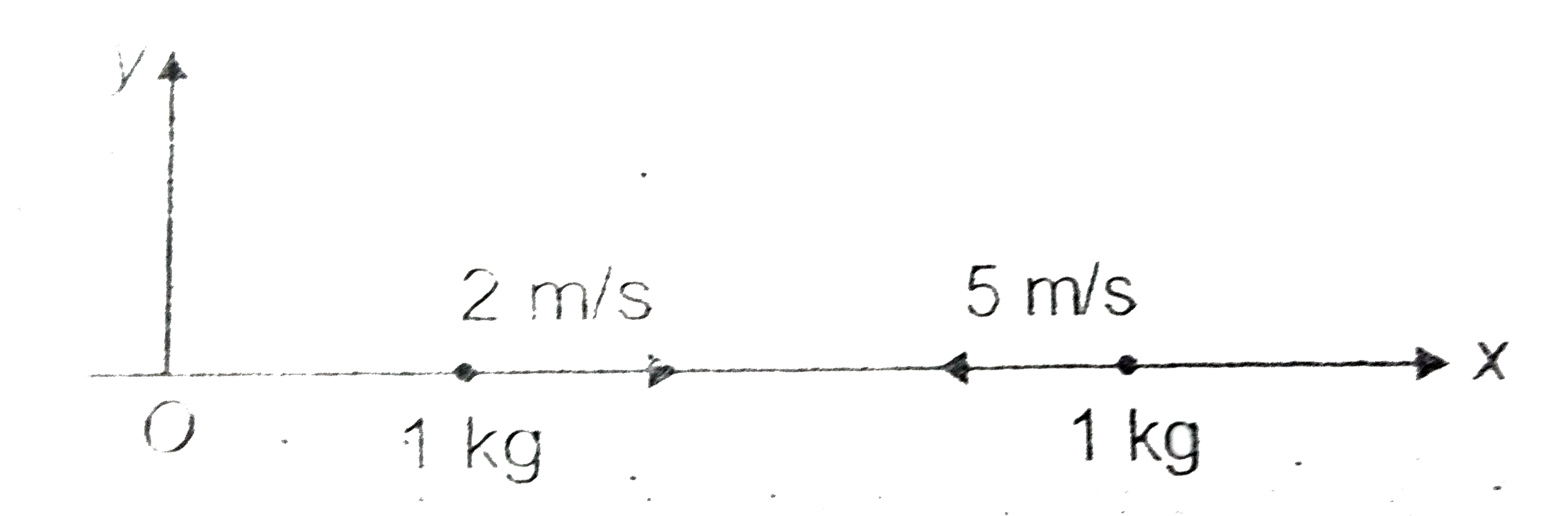
View SolutionTwo particles each of mass m moving with equal speed 2m/s along the indicated direction in the figure undergo a perfectly inelastic collision.Velocity of combined system after collision is ?
View Solution
Knowledge Check
Two identical balls moving in opposite direction with speed 20 m/s and 25 m/s undergo head on perfectly inelastic collision. The spped of combined mass after collision is
A20msB22.5msC25msD2.5msA particle of mass m moving with velocity →V makes a head on elastic collision with another particle of same mass initially at rest. The velocity of the first particle after the collision will be
A→VB−→VC−2→VDZeroA particle of mass m moving with velocity →V makes a head on elastic collision with another particle of same mass initially at rest. The velocity of the first particle after the collision will be
A→VB−→VC−2→VDzero
Two particles of mass 2m and m moving with speed v and 2v respectively pependicular to each other collides perfectly inelastically, then

Find the angle with the horizontal to which the particles move after collision.
View SolutionA particle (a mud pallet, say) of mass m strikes a smooth stationary wedge of mass M with as velocity v0 at an angle θ with horizontal. If the collision is perfectly inelastic, find the
a. velocity of the wedge just after the collision.
b. Chane in KE of the system (M+m) in collision.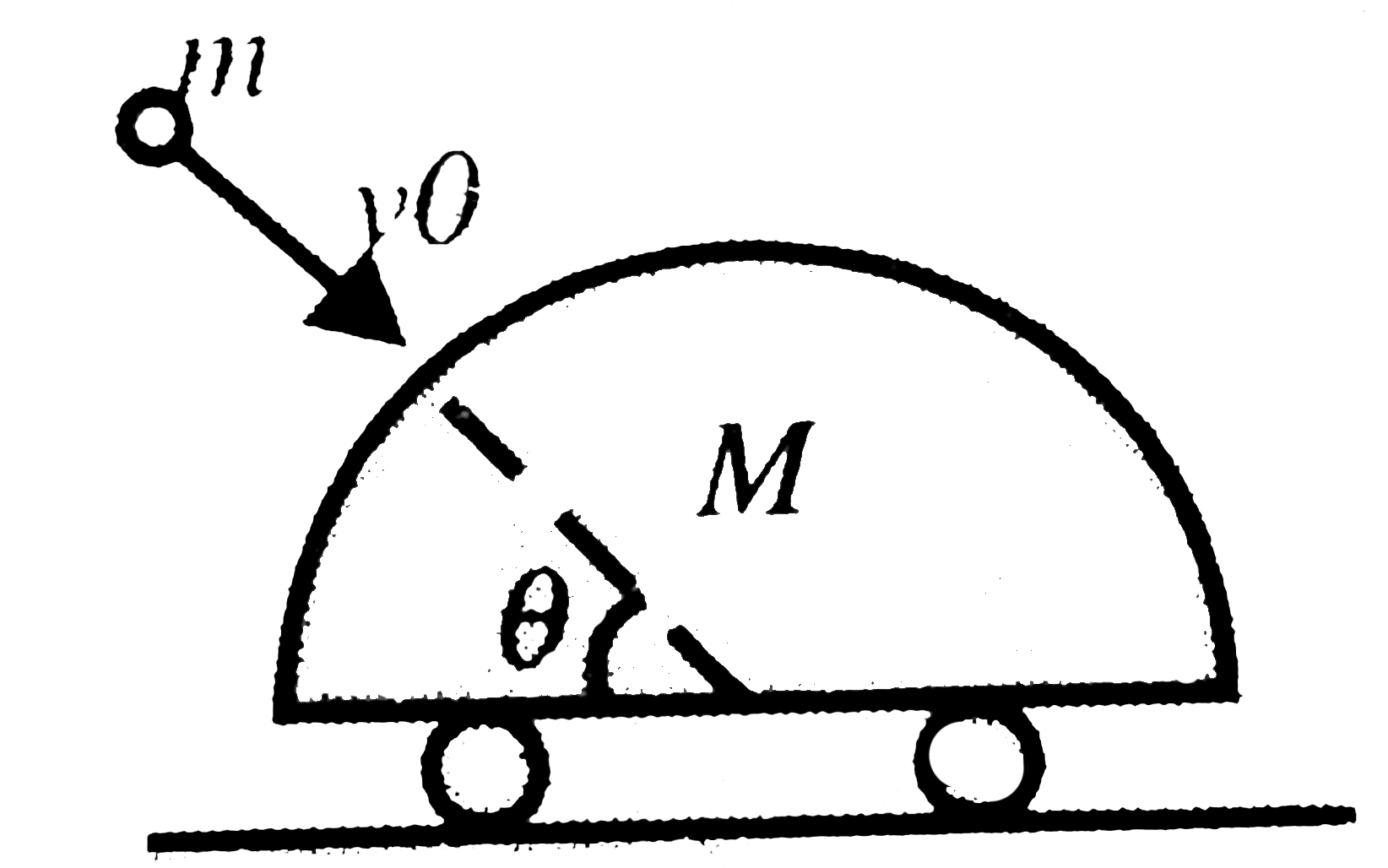
View SolutionA particle of mass 2kg moving with a velocity 5ˆim/s collides head-on with another particle of mass 3kg moving with a velocity −2ˆim/s. After the collision the first particle has speed of 1.6m/s in negative x-direction, Find
(a) velocity of the centre of mass after the collision,
(b) velocity of the second particle after the collision.
(c) coefficient of restitution.
View SolutionTwo identical balls moving in opposite directions with speed 20 m/s and 25 m/s undergo head on perfectly inelastic collision. The speed of combined mass after collision is
View SolutionA ball of mass M moving with a velocity v collides perfectly inelastically with another ball of same mass but moving with a velocity v in the opposite direction. After collision
View Solution
