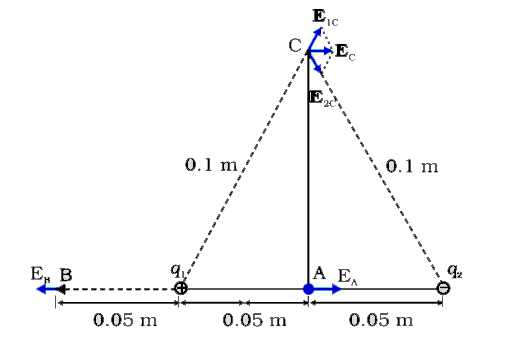
Two point charges q1andq2, of magnitude +10–8Cand–10–8C, respectively, are placed 0.1 m apart. Calculate the electric fields at points A, B and C shown in Fig. 1.14.
EA=7.2×104NC−1 EB=3.2×104NC−1
EB is directed towards the RIGHT.
EA=7.2×10−4NC−1 EB=3.2×104NC−1
EB is directed towards the left.
EA=7.2×104NC−1 EB=3.2×104NC−1
EB is directed towards the left.
EA=7.2×104NC−1 EB=3.2×10−4NC−1
EB is directed towards the left.
The correct Answer is:C
The electric field vector E1A at A due to the positive charge q1 points towards the right and has a magnitude
E1A=(9×109NmC2)×(10−8C)(0.05m2)=3.6104NC−1
The electric field vector E2A at A due to the negative charge q2 points towards the right and has the same magnitude. Hence the magnitude of the total electric field EA at A is
EA=E1A+E2A=7.2×104NC−1
EA is directed toward the light.
The electric field vector E1B at B due to the positive charge q1 points towards the left and has a magnitude
E1B=(9×109Nm2C−2)×(10−8C)(0.05m)2=3.6×104NC−1
The electric field vector E2B at B due to the negative charge q2 points towards the right and has a magnitude
E1B=(9×109Nm2C−2)×(10−8C)(0.15m)2=4×103NC−1
The magnitude of the total electric field at B is
EB=E1B−E2B=3.2×104NC−1
EB is directed towards the left.
The directions in which these two vectors point are indicated in Fig. 1.14. The resultant of these two vectors is
EC=E1cos(π)3+E2Cosπ3=9×10−3NC−1
Ec points towards the right.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERTCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE
34 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERTCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
37 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERTCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
17 Videos
Similar Questions
Two point charges q1andq2 of magnitude +10−8Cand−10−8C respectively are placed 0.1 m apart . Calculate the electric field at point B ,C and A
(##U_LIK_SP_PHY_XII_C01_E10_021_Q01.png" width="80%">
View SolutionTwo point charges q1andq2, of magnitude +10–8Cand–10–8C, respectively, are placed 0.1 m apart. Calculate the electric fields at points A, B and C shown in Fig. 1.14.

View Solution
Knowledge Check
Two point charges +10−7C and −10−7C are placed at A and B 20 cm apart as shown in the figure. Calculate the electric field at C, 20 cm apart from both A and B.
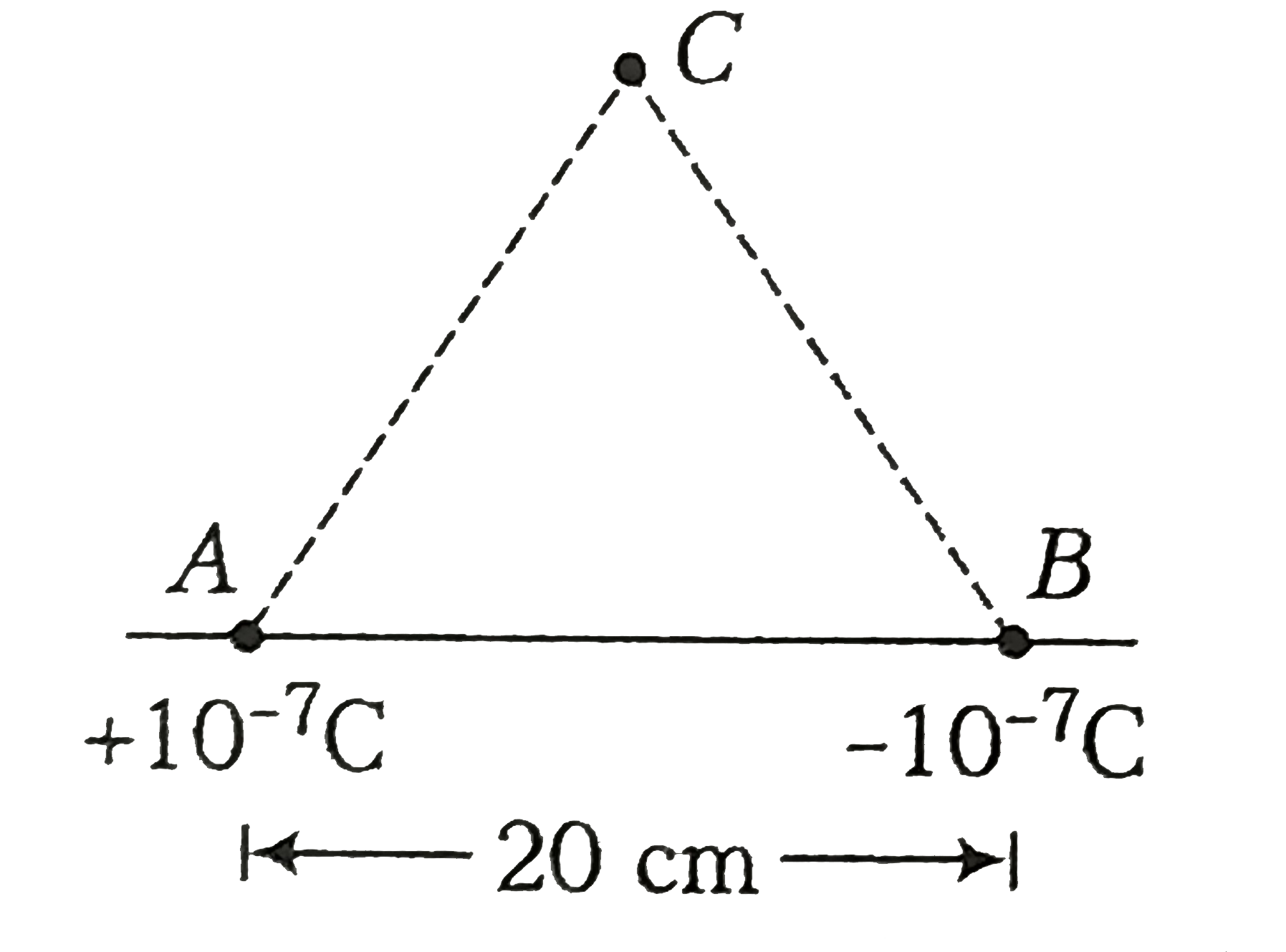 A1.5×10−5NC−1B2.2×104NC−1C3.5×106NC−1D3.0×105NC−1
A1.5×10−5NC−1B2.2×104NC−1C3.5×106NC−1D3.0×105NC−1In, the charges on C1,C2, and C3, are Q1,Q2, and Q3, respectively.
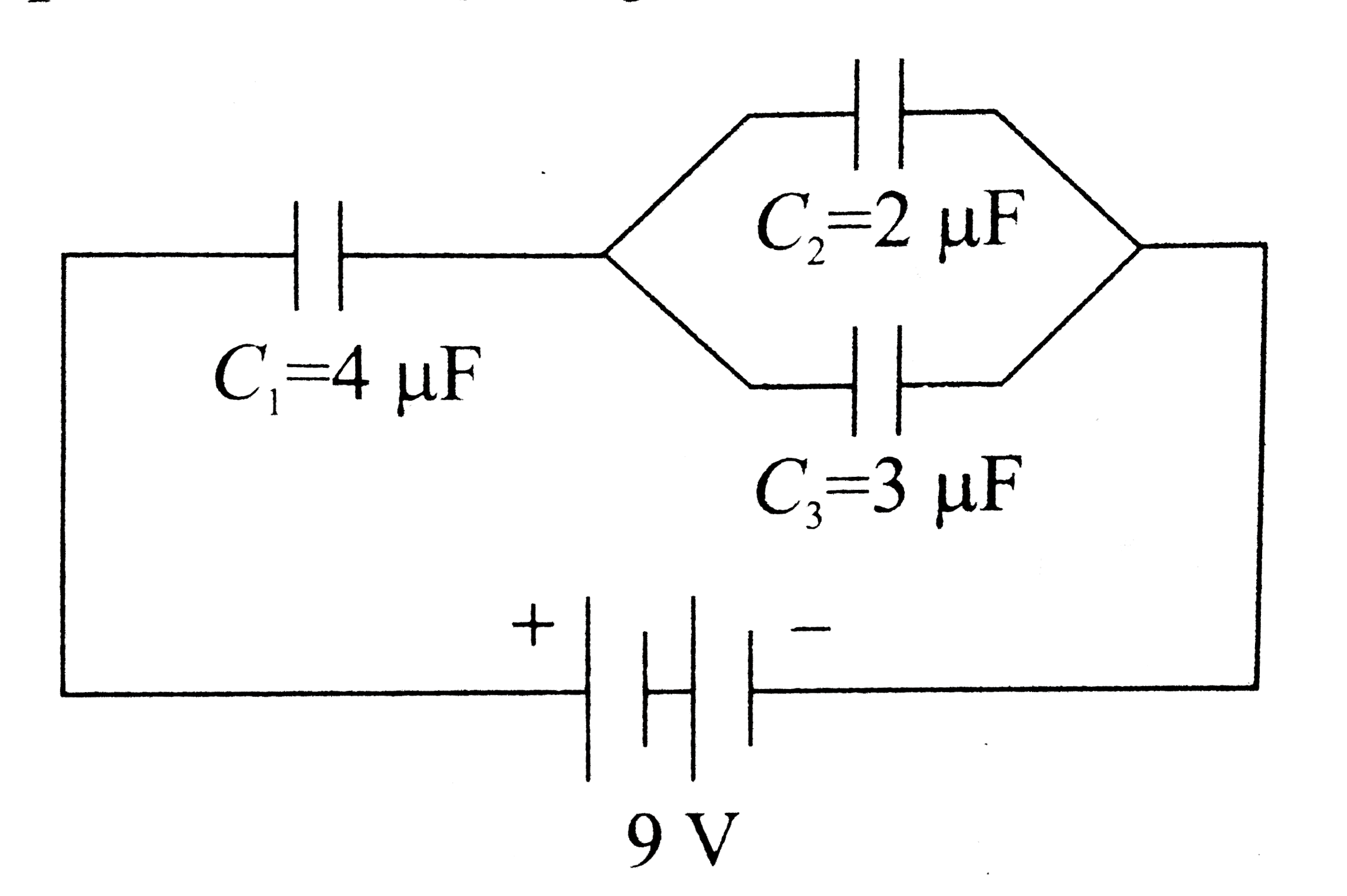 .AQ2=8μCBQ3=12μCCQ1=20μCDQ2=12μC
.AQ2=8μCBQ3=12μCCQ1=20μCDQ2=12μC
Two pont chargees q1 and q2 of 2×10−8C and −2×10−8C respectively are placed 0.4 m apart. Calculate the electric field at the centre of the line joining the two charges.
View SolutionTwo point charges, q1=10×10−8Candq2=−2×10−8C, are separated by a distance of 60 cm in air.
Also calculate the electrostatic potential energy of the system.
View SolutionTwo particles A and B, having opposite charges2.0×10−6 and −2.0×10−6C , are placed at a separaton of 1.0 cm.(b) Calculate the electric field at a point on the axis of the dipole 1.0 m away from the centre. (c ) Calculate the electric field at a point on the perpendicular bisector of the dipole and 1.0 m away from the centre.
View SolutionFind the expression for the potential energy of a system of two point charges q1,andq2 glocated at →r1and→r2 respectively in an external electric field →E.
(b) Draw equipotential surfaces due to an isolated point charge (-q) and depict the electric field lines.
(c) Three point charges +1μC,−1μCand+2μC are initially infinite distance apart. Calculate the work done in assembling these charges at the vertices of an equilateral triangle of side 10 cm.
View SolutionTwo charges +0.2μμCand−0.2μμC are placed 10−6cm apart. Calculate electric field at an axial point at a distance of 10 cm from their middle point.
View SolutionTwo point charges of, 1 μC and -1 μC are kept at (-2 m, 0) and (2 m, 0) respectively. Calculate the electric field at point (0, 5 m).
View Solution
