Two point charges with charge magnitudes 2 nC and 3 nC are placed at a separation of 1 cm from each other and then released. The mass of each point charge is 1 μg. Find the magnitude of acceleration of particles at the instant they are released.
To find the magnitude of acceleration of the two point charges at the instant they are released, we will follow these steps:
Step 1: Identify the Given Values
- Charge Q1=2nC=2×10−9C
- Charge Q2=3nC=3×10−9C
- Distance R=1cm=0.01m
- Mass of each charge m=1μg=1×10−9kg
Step 2: Calculate the Force Between the Charges Using Coulomb's Law
Coulomb's Law states that the force F between two point charges is given by:
F=kQ1Q2R2
Where k is Coulomb's constant, approximately 9×109N m2/C2.
Substituting the values:
F=9×109(2×10−9)(3×10−9)(0.01)2
Calculating R2:
R2=(0.01)2=0.0001m2
Now substituting this into the force equation:
F=9×109(6×10−18)0.0001
Calculating the force:
F=9×109×6×10−14=54×10−5N=5.4×10−4N
Step 3: Calculate the Acceleration of Each Charge
Using Newton's second law, the acceleration a can be calculated using the formula:
a=Fm
Substituting the values:
a=5.4×10−41×10−9
Calculating the acceleration:
a=5.4×105m/s2
Final Result
The magnitude of the acceleration of each charge at the instant they are released is:
5.4×105m/s2
- Charge Q1=2nC=2×10−9C
- Charge Q2=3nC=3×10−9C
- Distance R=1cm=0.01m
- Mass of each charge m=1μg=1×10−9kg
Coulomb's Law states that the force F between two point charges is given by:
Using Newton's second law, the acceleration a can be calculated using the formula:
The magnitude of the acceleration of each charge at the instant they are released is:
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Conceptual Questions
51 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Tough & Tricky Problems
20 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
Similar Questions
Two point charges 9 μC and −2μC are placed at a separation of 20 cm from each other.
(i) Calculate the electric potential energy of this system of two charges.
(ii) What amount of work is required to separate the particles to infinite separation?
View SolutionTwo charges of values 2μC and −50μC are placed at a distance of 6 cm from each other. The distance of the point (in cm) from the bigger charge where the electric intensity will be zero is :
View Solution
Knowledge Check
Two identical non-conducting spheres having charges of -12 nC and + 8 nC are touched together and then separated. The 3 nal charge on each is
A−2ncB−(23)ncC+2nCD+4nCTwo spheres of electric charges +2nC and −8nC are placed at a distance d apart. If they are allowed to touch each other, whatis the new distance between them to get a repulsive force of same magnitude as before?
AdBd2C3d4D4d4
Two point charges, each of mass m and charge q are released when they are at a distance from each other. What is the speed of each charged particle when they are at a distance 2r ?
View SolutionAn infinte wire having linear charge density λ is arranged as shown in fig. A charge particle of mass m and charge q is released charged q is released from point P. Find the initial acceleration of the particle just after the particle is released.
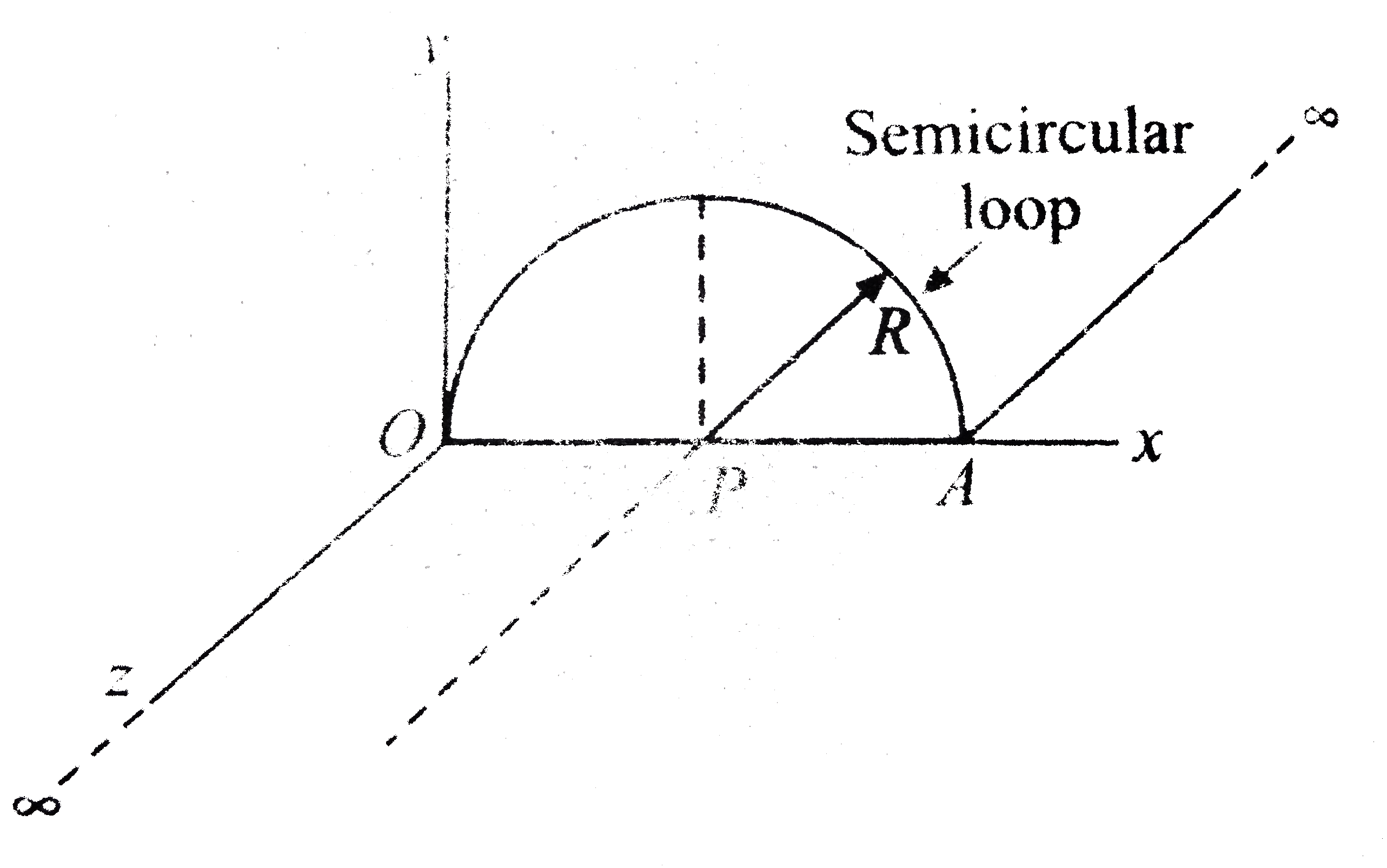
View SolutionTwo point charges Q are kept fixed on XY-plane at points A(0, d) and B(0, -d). Another point charge -q with mass m is held at a point, on the positive side of X-axis that is at the distance 2d from both the fixed point charges. What will be the magnitude of acceleration of the negative point charge (i) just after it is released from its location? (ii) at an instant it crosses the origin?
View SolutionThere are two point charges +4 mu C and -2 mu C .The ratio of magnitude of electrostatic forces acting on them respectively will be
View SolutionTwo positive point charges , each Q , are fixed at separation d. A third charge q is placed in the middle. Describe the equilibrium of the third charge.
View SolutionTwo small conducting spheres have charges 2.1nC and −0.1nCare touched to each other and then separated by a distance of 0.5 m. Find the force between them
View Solution
