Two resistors of resistance $R_{1}$ and $R_{2}$ having $R_{1} > R_{2}$ are connected in parallel. For equivalent resistance R, the correct statement is

Video Solution

Text Solution

Verified by Experts

Let $v_{0}$ be the terminal velocity attained by the rod PQ (in the steady state). If $i_{1}$ and $i_{2}$ be the currents flowing through $R_{1}$ and $R_{2}$ in this state, then current flowing through the rod PQ is $i = i_{1} + i_{2}$ (see the circuit diagram) as shown in Fig.

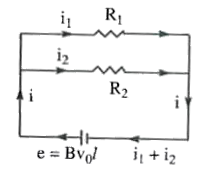
$∴$ Applying Kirchoff.s loop rule, yields.
$i_{1} R_{1} = B v_{0} l$ and $i_{2} R_{2} = B v_{0} l$
$∴ i_{1} + i_{2} = B v_{0} l (\frac{1}{R_{1}} + \frac{1}{R_{2}})$ .....(i)
Given that, $P_{1} = i_{1}^{2} R_{1} = \frac{B^{2} v_{0}^{2} l^{2}}{R_{1}}$ .....(ii) and $P_{2} = i_{2}^{2} R_{2} = \frac{B^{2} v_{0}^{2} l^{2}}{R_{2}}$ .....(iii)
Also in the steady state, the acceleration of PQ = 0.
$\Rightarrow m g = B (i_{1} + i_{2}) l$ (or) $m g = B^{2} l^{2} v_{0} (\frac{1}{R_{1}} + \frac{1}{R_{2}})$ .....(iv)
Multiplying both sides by $v_{0}$ , we get
$m g v_{0} = B^{2} l^{2} v_{0}^{2} (\frac{1}{R_{1}} + \frac{1}{R_{2}}) = P_{1} + P_{2}$ (From Eq. (ii) and (iii)]
$∴$ The terminal velocity is $v_{0} = \frac{P_{1} + P_{2}}{m g}$
Substituting for $v_{0}$ in Eq. (ii),
$P_{1} = \frac{B^{2} l^{2}}{R_{1}} (\frac{P_{1} + P_{2}}{m g}) \Rightarrow R_{1} = {[\frac{B l (P_{1} + P_{2})}{m g}]}^{2} \times \frac{1}{P_{1}}$
Similarly from Eg. (iii) $R_{2} = {[\frac{B l (P_{1} + P_{2})}{m g}]}^{2} \times \frac{1}{P_{2}}$

Updated on:21/07/2023