Two sphere AandB of masses m1andm2 respectivelly colides. A is at rest initally and B is moving with velocity v along x-axis. After collision B has a velocity v2 in a direction perpendicular to the original direction. The mass A moves after collision in the direction.
θ=tan−1(−1/2) to the x axis
same as that of B
opposite to that of B
θ=tan−1(1/2) to the x axis
To solve the problem, we will use the principle of conservation of momentum. The problem involves two spheres, A and B, with given masses and velocities before and after the collision. Let's break down the solution step by step.
Step 1: Define the Given Information
- Mass of sphere A: m1
- Mass of sphere B: m2
- Initial velocity of sphere A: u1=0 (at rest)
- Initial velocity of sphere B: u2=v (moving along the x-axis)
- Final velocity of sphere B after collision: v′2=v2 (perpendicular to the original direction, which we can assume is in the positive y-direction)
- Final velocity of sphere A after collision: v′1 (unknown, making an angle θ with the negative x-axis)
Step 2: Apply Conservation of Momentum in the x-direction
Before the collision, the total momentum in the x-direction is:
pinitial,x=m2⋅v+m1⋅0=m2v
After the collision, the momentum in the x-direction is:
pfinal,x=m1v′1cos(θ)+m2⋅0=m1v′1cos(θ)
Setting the initial and final momenta equal gives us:
m2v=m1v′1cos(θ)(Equation 1)
Step 3: Apply Conservation of Momentum in the y-direction
Before the collision, the total momentum in the y-direction is:
pinitial,y=0
After the collision, the momentum in the y-direction is:
pfinal,y=m1(−v′1sin(θ))+m2(v2)
Setting the initial and final momenta equal gives us:
0=m1(−v′1sin(θ))+m2(v2)(Equation 2)
Step 4: Solve the Equations
From Equation 1:
v′1cos(θ)=m2vm1
From Equation 2:
m1v′1sin(θ)=m2v2
Now we can express v′1 from both equations:
1. v′1=m2vm1cos(θ)
2. Substitute this into Equation 2:
m1(m2vm1cos(θ))sin(θ)=m2v2
This simplifies to:
m2vsin(θ)cos(θ)=m2v2
Cancelling m2v (assuming m2≠0 and v≠0):
sin(θ)cos(θ)=12
Thus, we have:
tan(θ)=12
Therefore:
θ=tan−1(12)
Conclusion
The direction in which mass A moves after the collision is at an angle θ=tan−1(12) with respect to the negative x-axis.
- Mass of sphere A: m1
- Mass of sphere B: m2
- Initial velocity of sphere A: u1=0 (at rest)
- Initial velocity of sphere B: u2=v (moving along the x-axis)
- Final velocity of sphere B after collision: v′2=v2 (perpendicular to the original direction, which we can assume is in the positive y-direction)
- Final velocity of sphere A after collision: v′1 (unknown, making an angle θ with the negative x-axis)
Before the collision, the total momentum in the x-direction is:
pinitial,x=m2⋅v+m1⋅0=m2v
After the collision, the momentum in the x-direction is:
pfinal,x=m1v′1cos(θ)+m2⋅0=m1v′1cos(θ)
Setting the initial and final momenta equal gives us:
m2v=m1v′1cos(θ)(Equation 1)
Before the collision, the total momentum in the y-direction is:
pinitial,y=0
After the collision, the momentum in the y-direction is:
pfinal,y=m1(−v′1sin(θ))+m2(v2)
Setting the initial and final momenta equal gives us:
0=m1(−v′1sin(θ))+m2(v2)(Equation 2)
From Equation 1:
v′1cos(θ)=m2vm1
From Equation 2:
m1v′1sin(θ)=m2v2
Now we can express v′1 from both equations:
1. v′1=m2vm1cos(θ)
2. Substitute this into Equation 2:
m1(m2vm1cos(θ))sin(θ)=m2v2
This simplifies to:
m2vsin(θ)cos(θ)=m2v2
Cancelling m2v (assuming m2≠0 and v≠0):
sin(θ)cos(θ)=12
Thus, we have:
tan(θ)=12
Therefore:
θ=tan−1(12)
The direction in which mass A moves after the collision is at an angle θ=tan−1(12) with respect to the negative x-axis.
Topper's Solved these Questions
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - AIIMS Questions
20 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Chapter Test
29 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Assertion Reasoning
17 Videos
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Chapter Test
30 Videos
Similar Questions
Two balls A and B of masses 0.3 kg and 0.2 kg respectively are moving along positive X-axis and negaive X-axis with velocities 2.0 m / s. They collide and thereafter move in the directions opposite to their original directions. Find the velocities of A and B after collision. Also, calculate total K.E. of the ball before and after collision.
View SolutionA ball moving with a velocity 12m/s strikes an identical ball at rest.After collision both balls move in directions making 300 with original direction of motion.speeds of the balls after collision are (in ms−1) ?
View Solution
Knowledge Check
Two spheres a and B of masses m1 and m2 respectively collide. A is at rest initially and B is moving with velocity v along x-axis. After collision B has a velocity v/2 in a direction perpendicular to the original direction. The mass A moves after collision in the direction
Asame as that of BBopposite to that of BCθ=tan−1(12) to the x-axisDθ=tan−1(−12) to the x-axisTwo spheres A and B of masses m1andm2 respectively collide. A is at rest initially and B is moving with velocity v along x-axis. After collision B has a velocity v2direction perpendicular to the original direction. The mass A moves after collision in the direction
Asame as that of BBopposite to that of BCθ=tan−1(12) to the axisDθ=tan−1(−12) to the x-axisA mass m moves with a velocity v and collides inelastically with another identical mass. After collision the 1st mass moves with velocity v√3 in a direction perpendicular to the initial direction of motion. Find the speed of the second mass after collision
AvB√3vC2√3vDv√3
A ball of mass m moving with velocity v collides head on elastically with another identical ball moving with velocity - V. After collision
View SolutionA sphere of mass m moves with a velocity 2v and collides inelastically with another identical sphere of mass m. After collision the first mass moves with velocity v in a direction perpendicular to the initial direction of motion . Find the speed of the second sphere after collision .
View SolutionA mass ‘m’ moves with a velocity ‘v’ and collides inelastically with another identical mass at rest. After collision the 1st mass moves with velocity v√3 in a direction perpendicular to the initial direction of motion. Find the speed of the 2nd mass after collision :
View SolutionA mass m moves with a velocity v and collides inelastically with another identical mass at rest. After collision, the first mass moves with velocity v√3 in a direction perpendicular to Before collision the initial direction of motion. The speed of the 2nd mass after collision
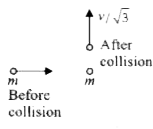
View SolutionA body of mass m moving at a constant velocity v hits another body of the same mass moving with a velocity v/2 but in the opposite direction and sticks to it. The common velocity after collision is
View Solution
