Two stars each of mass M and radius R are approaching each other for a head-on collision. They start approaching each other when their separation is r>>R. If their speed at this separation are negligible, the speed v with which they collide would be
v=√GM(1R−1r)
v=√GM(12R−1r)
v=√GM(1R+1r)
v=√GM(12R+1r)
The correct Answer is:B
To solve the problem of two stars colliding, we will use the principle of conservation of mechanical energy. Here's a step-by-step solution:
Step 1: Understand the Initial Conditions
- We have two stars, each with mass M and radius R.
- They start approaching each other from a separation r (where r≫R).
- Their initial speeds are negligible, meaning their initial kinetic energy is zero.
Hint: Identify the initial conditions clearly, including the masses, radii, and initial separation.
Step 2: Write the Initial Mechanical Energy
The initial mechanical energy Ei consists of gravitational potential energy and kinetic energy. Since the stars are at rest initially, the kinetic energy is zero.
Ei=Potential Energy+Kinetic Energy=Ui+Ki=Ui+0
The gravitational potential energy Ui at separation r is given by:
Ui=−GM2r
where G is the gravitational constant.
Hint: Remember that gravitational potential energy is negative and depends on the distance between the two masses.
Step 3: Write the Final Mechanical Energy
When the stars collide, they will be at a separation of 2R (the distance between their centers). The final mechanical energy Ef consists of kinetic energy and potential energy at this point.
1. Kinetic Energy: If both stars have the same speed v just before collision, their total kinetic energy is:
Kf=12Mv2+12Mv2=Mv2
2. Potential Energy: At separation 2R:
Uf=−GM22R
Thus, the final mechanical energy is:
Ef=Kf+Uf=Mv2−GM22R
Hint: Ensure to account for both kinetic and potential energy in the final state.
Step 4: Apply Conservation of Mechanical Energy
According to the conservation of mechanical energy, the initial energy equals the final energy:
Ei=Ef
Substituting the expressions we derived:
−GM2r=Mv2−GM22R
Hint: Set up the equation carefully, ensuring both sides represent the same physical quantity.
Step 5: Rearrange the Equation
Rearranging the equation to solve for v2:
Mv2=−GM2r+GM22R
Factoring out GM2:
Mv2=GM2(−1r+12R)
Dividing both sides by M:
v2=GM(−1r+12R)
Hint: Isolate v2 to find the expression for velocity.
Step 6: Solve for v
Taking the square root gives us the final speed v:
v=√GM(12R−1r)
Hint: Remember to take the square root and ensure the expression is simplified correctly.
Final Result
The speed v with which the two stars collide is:
v=√GM(12R−1r)
- We have two stars, each with mass M and radius R.
- They start approaching each other from a separation r (where r≫R).
- Their initial speeds are negligible, meaning their initial kinetic energy is zero.
The initial mechanical energy Ei consists of gravitational potential energy and kinetic energy. Since the stars are at rest initially, the kinetic energy is zero.
When the stars collide, they will be at a separation of 2R (the distance between their centers). The final mechanical energy Ef consists of kinetic energy and potential energy at this point.
According to the conservation of mechanical energy, the initial energy equals the final energy:
Rearranging the equation to solve for v2:
Taking the square root gives us the final speed v:
The speed v with which the two stars collide is:
Topper's Solved these Questions
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Problems Based On Mixed Concepts
29 Videos
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Assertion Reasoning
16 Videos
- GRAVITATIONBOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Gravitational Potential, Energyand Escape Velocity
32 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test
30 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBOOK - A2ZCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Chapter Test
29 Videos
Similar Questions
Two starts each of one solar mass (=2×1030kg) are approaching each other for a head on collision. When they are a distance 109km. their speeds are negligible. What is the speed with which they collide? The radius of each star is 104km. Assume the stars to remain undistorted until they collide. (Use the known value of G).
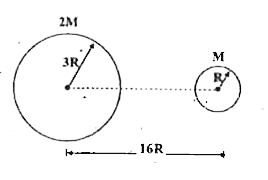
View SolutionTwo spherical bodies of masses 2M and M and radii 3R and R respectively are held at a distance 16R from each other in free space. When they are released they start approaching each other due to the gravitational force of attraction , then find
(a) the ratio of their accelerations during their motion
(b) their velocitites at the time of impact.
View Solution
Knowledge Check
Two particles of mass m1 and m2 are approaching towards each other under their mutual gravitational field. If the speed of the particle is v, the speed of the center of mass of the system is equal to :
Am1m2vBZeroC(m1−m2)vm1+m2Dm2m1vTwo pulses on a string approach each other at speeds of 1m/s what is the shape of the string at t=6s?
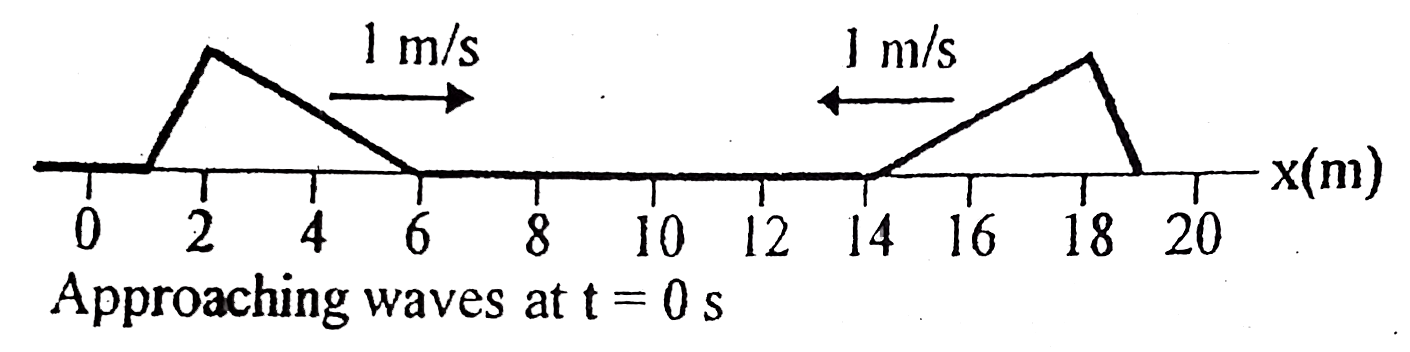 A
A B
B C
C D
D
Two particles of mass m and M are initially at rest and infinitely separated from each other. Due to mutual interaction, they approach each other.
Their relative velocity of approach at a separation d between them, isA[2 GdM + m]1/2B[2 G(M+m)d]1/2C2 GM+mdDNone of these
Two spheres of masses 2M and M are initially at rest at a distance R apart. Due to mutual force of attraction, they approach each other. When they are at separation R/2, the acceleration of the centre of mass of spheres would be
View SolutionWhat will happen when two clouds with unlike charges approach each other?
View SolutionTwo particles of mass m1 and m2, approach each other due to their mutual gravitational attraction only. Then
View SolutionTwo particles of mass m1 and m2, approach each other due to their mutual gravitational attraction only. Then
View SolutionThree solid spheres each of mass m and radius R are released from the position shown in figure. The speed of any one sphere at the time of collision would be
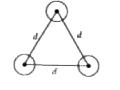
View Solution
