Two spherical bodies of masses $2 M$ and $M$ and radii $3 R$ and $R$ respectively are held at a distance $16 R$ from each other in free space. When they are released they start approaching each other due to the gravitational force of attraction , then find
$(a)$ the ratio of their accelerations during their motion
$(b)$ their velocitites at the time of impact.

Text Solution

Verified by Experts

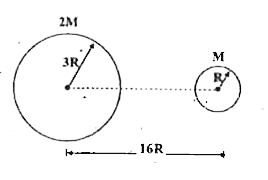
Taking both the bodies as a system, from conserving momentum of the system
$m_{1} v_{1} - m_{2} v_{2} = 0 \Rightarrow \frac{m_{1}}{m_{2}} = \frac{v_{2}}{v_{1}} = 2$
again , if the accelerations are $a_{1}$ and $a_{2}$ , the net external force on the system $= 0$
$\Rightarrow {\to a}_{C M} = 0 \Rightarrow m_{1} a_{1} - m_{2} a_{2} = 0$
$\frac{m_{1}}{m_{2}} = \frac{a_{2}}{a_{1}} = 2$
Now conserving the total mechanical energy, we have
$- \frac{G (2 M) M}{16 R} = - \frac{G (2 M) M}{4 R} + \frac{1}{2} (2 M) v_{1}^{2} + \frac{1}{2} (M) v_{2}^{2}$
$v_{1} = \sqrt{\frac{G M}{8 R}}$ , $v_{2} = 2 \sqrt{\frac{G M}{8 R}}$
Note : The velocities and accelerations are w.r.t. the inertial reference frame (i.e. the centre of mass of the system).

Updated on:21/07/2023

Two spherical bodies of masses m and 5m and radii R and 2R respectively, are released in free space with initial separation between their centres equal to 12 R. If they attract each other due to gravitational force only, the distance covered by smaller sphere just before collision is
A $\frac{15 R}{2}$
B $\frac{13 R}{2}$
C10R
D $\frac{17 R}{2}$
Two spherical bodies of masses $m$ and $4 m$ and radii R and 2R respectively are released in free space with initial separation between their centres equal to 15 R. If they attract each other due to gravitational force only, then the distance covered by smaller sphere just before collision will be

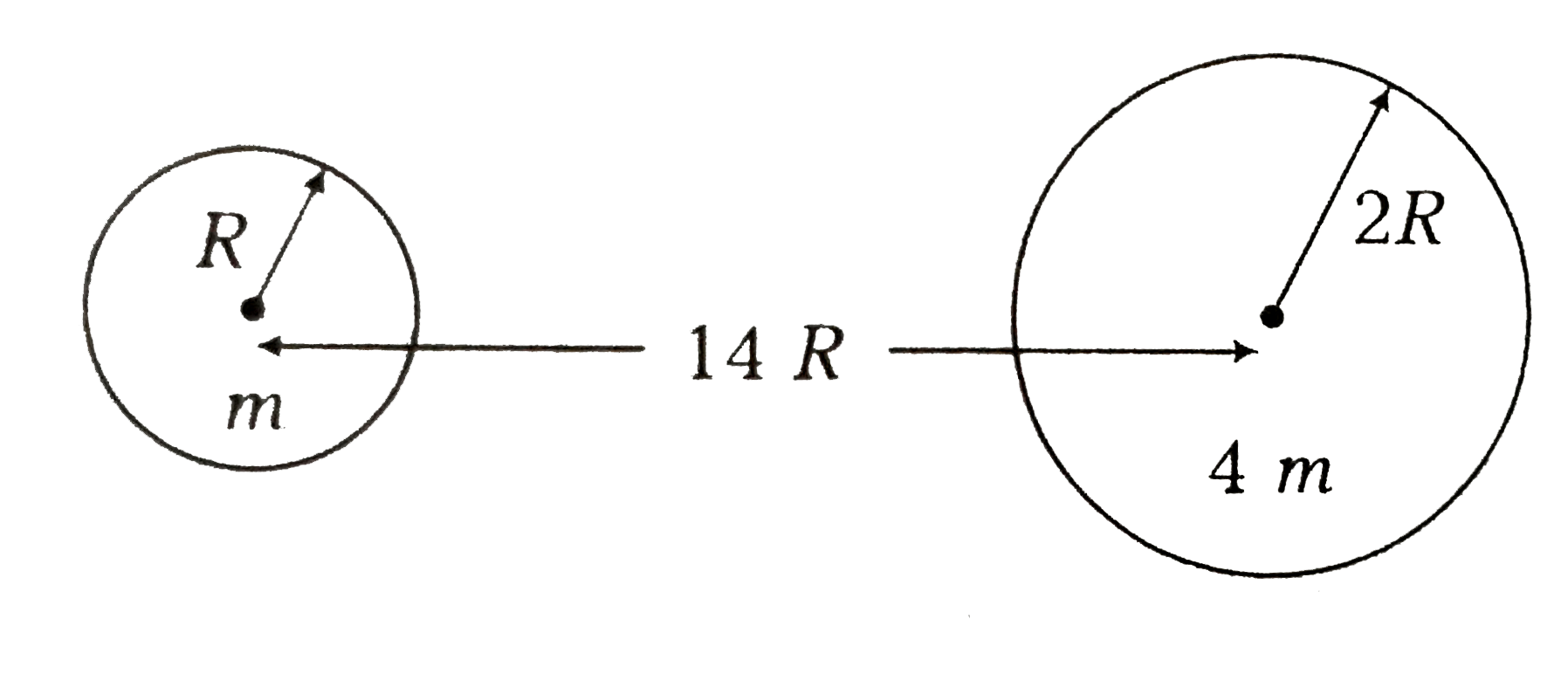A5 R
B9.6 R
C2.5 R
D6 R
Two spherical bodies of masses m and 6m and radii R and 2R respectively are released in free space with initial separation between their centres equal to 10 R . If they attract each other due to gravitational force only , then the distance covered by smaller sphere just before collisions will be
A6 R
B7.5 R
C2.5 R
D9 R