Two wires A and B have equal lengths and aremade of the same material , but diameter of wire A is twice that of wire B. Then, for a given load,
(A) the extension of B will be four times that of A
(B) the extensions of A and will be equal
(C) the strain in B is four times that in A
(D) the strains in A and B will be equal
The correct Answer is:A, C
To solve the problem, we will analyze the relationship between the extensions in two wires, A and B, which have the same length, are made of the same material, but have different diameters.
1. Understanding Young's Modulus:
Young's modulus (Y) is defined as the ratio of stress to strain. Mathematically, it is given by:
Y=F/AΔL/L
where:
- F is the applied force,
- A is the cross-sectional area,
- ΔL is the extension,
- L is the original length.
2. Cross-sectional Area Calculation:
The cross-sectional area A of a wire is given by:
A=πd24
where d is the diameter of the wire.
Given that the diameter of wire A is twice that of wire B, we can express the areas as:
- For wire A: AA=π(2dB)24=πd2B
- For wire B: AB=πd2B4
3. Setting Up the Equations for Young's Modulus:
For wire A:
YA=FAA⋅LΔLA⟹YA=Fπd2B⋅LΔLA
For wire B:
YB=FAB⋅LΔLB⟹YB=Fπd2B4⋅LΔLB=4Fπd2B⋅LΔLB
4. Equating Young's Modulus:
Since both wires are made of the same material, their Young's moduli are equal:
YA=YB
Thus, we have:
Fπd2B⋅LΔLA=4Fπd2B⋅LΔLB
5. Simplifying the Equation:
Canceling out common terms (F, π, and L) gives:
1ΔLA=4ΔLB
Rearranging this, we find:
ΔLA=4ΔLB
6. Conclusion:
The extension in wire A is four times the extension in wire B:
ΔLA=4ΔLB
Final Answer:
The extension in wire A is 4 times the extension in wire B.
Young's modulus (Y) is defined as the ratio of stress to strain. Mathematically, it is given by:
Y=F/AΔL/L
where:
- F is the applied force,
- A is the cross-sectional area,
- ΔL is the extension,
- L is the original length.
The cross-sectional area A of a wire is given by:
A=πd24
where d is the diameter of the wire.
Given that the diameter of wire A is twice that of wire B, we can express the areas as:
- For wire A: AA=π(2dB)24=πd2B
- For wire B: AB=πd2B4
For wire A:
YA=FAA⋅LΔLA⟹YA=Fπd2B⋅LΔLA
For wire B:
YB=FAB⋅LΔLB⟹YB=Fπd2B4⋅LΔLB=4Fπd2B⋅LΔLB
Since both wires are made of the same material, their Young's moduli are equal:
YA=YB
Thus, we have:
Fπd2B⋅LΔLA=4Fπd2B⋅LΔLB
Canceling out common terms (F, π, and L) gives:
1ΔLA=4ΔLB
Rearranging this, we find:
ΔLA=4ΔLB
The extension in wire A is four times the extension in wire B:
ΔLA=4ΔLB
The extension in wire A is 4 times the extension in wire B.
Topper's Solved these Questions
- ELASTICITYBook:DC PANDEY ENGLISHChapter:ELASTICITYExercise:Level 2 Comprehension Based
Explore 6 Videos
- ELASTICITYBook:DC PANDEY ENGLISHChapter:ELASTICITYExercise:Level 2 Subjective
Explore 10 Videos
- ELASTICITYBook:DC PANDEY ENGLISHChapter:ELASTICITYExercise:Level 2 Single Correct
Explore 10 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:All Questions
Explore 469 Videos
- ELECTROSTATICSBook:DC PANDEY ENGLISHChapter:ELECTROSTATICSExercise:Integer
Explore 17 Videos
Similar Questions
Two wires A and B have the same cross section and are made of the same material, but the length of wire A is twice that of B. Then, for a given load
View SolutionTwo wires A and B of equal masses and of the same material are taken. The diameter of wire A is half the diameter of wire B. if the resistance of wire A is 24Ω, calculate the resistance of wire B.
View SolutionTwo wires are together end to end . The wires are made of the same material , but the diameter of one is twice that of the other . They are subjected to a tension of 4.60N. The thin wire has a length of 40.0cm and a linear mass density of 2.00g/m. The combination is fixed at both ends and vibrated in such a way that two antinodes are present , with the node between them being precisely at the weld . (a) What is the frequency of vibration ? (b) Find the length of the thick wire .
View SolutionTwo wires P and Q are equally thick and made of the same material but the length of Pis twice that of Q
(a) Which wire will have a greater extension for a given load ?
(b) Which one will undergo a greater strain ?
View SolutionTwo uniform wires A and B are of the same total metal and have equal masses. The radius of wire A is twice that of wire B. The total resistance of A and B when connected in parallel is
View SolutionThe following diagram represents an electrical circui containing two uniform resistance wires A and B connected to a single ideal cell. Both wires have same length, but thickness of wire B is twice that of wire A. The conducting wires connecting A and B to the ideal cell are resistanceless. The dependence of electric potential on position along the length of two wires is given in option.
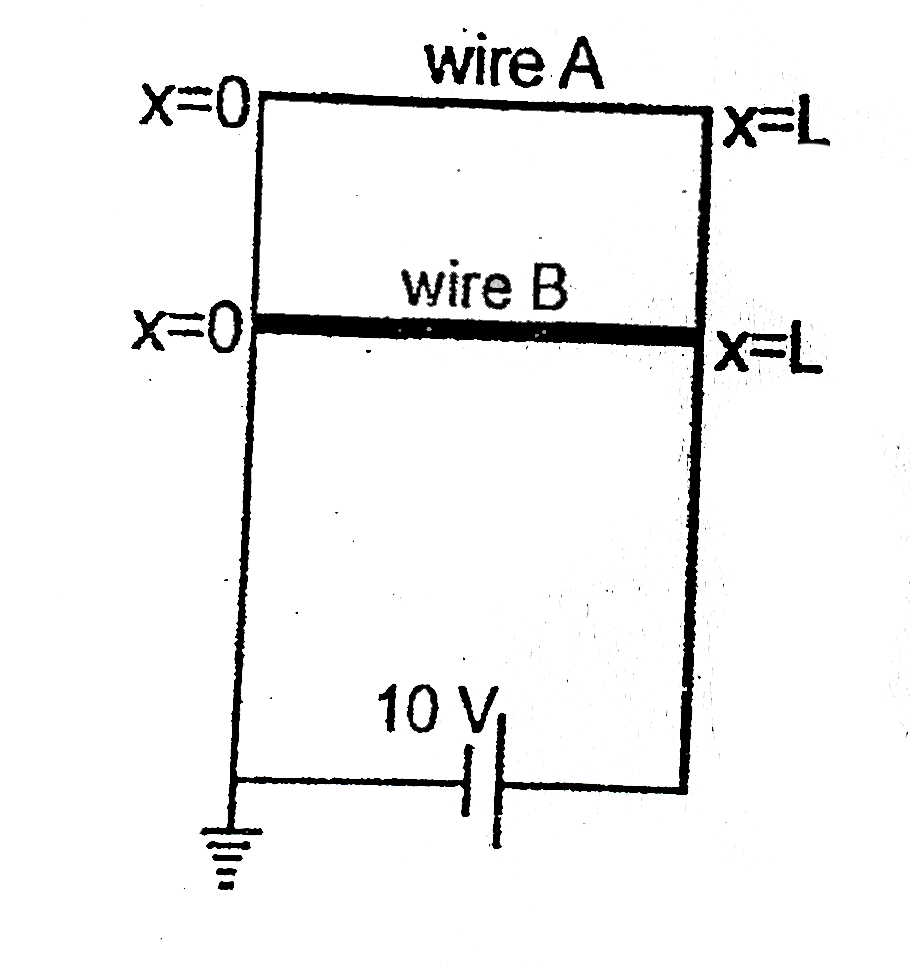
View SolutionTwo wires A and B of same length are made of same material . The figure represents the load F versus extension Δx graph for the wires. Then

View SolutionWires A and B have have identical lengths and have circular cross-sections. The radius of A is twice the radius of B i.e. RA=2RB. For a given temperature difference between the two ends, both wires conduct heat at the same rate. The relation between the thermal conductivities is given by-
View SolutionThe length of a wire is increased by 1mm on the application, of a given load. In a wire of the same material, but of length and radius twice that of the first, on application of the same load, extension is
View Solution
A wire of length 2L, is made by joining two wires A and B of same length but different radii r and 2r and made of the same material. It is vibrating at a frequency such that the joint of the two wires forms a node. If the number of antionodes in wire A is P and that in B is q then the ratio p:q is:
View Solution