Two wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×1011Nm−2
To solve the problem, we will use the concept of Young's modulus and the relationship between stress, strain, and elongation in the wires.
1. Identify Given Data:
- Young's modulus of steel, ES=2.0×1011N/m2
- Young's modulus of copper, EC=1.1×1011N/m2
- Let the lengths of the steel and copper wires be LS and LC respectively.
- The elongations in both wires are equal, ΔLS=ΔLC.
2. Understand the Relationship:
- The stress in each wire is the same because they are under the same tension and have equal cross-sectional areas.
- Stress is defined as Stress=TA, where T is the tension and A is the cross-sectional area.
- Since the areas are equal and the tension is the same, we have:
StressS=StressC
3. Relate Young's Modulus to Strain:
- Young's modulus is defined as:
E=StressStrain=T/AΔL/L
- Rearranging gives us:
Stress=E⋅Strain
- For steel:
StressS=ES⋅ΔLSLS
- For copper:
StressC=EC⋅ΔLCLC
4. Set the Stress Equations Equal:
- Since ΔLS=ΔLC and StressS=StressC:
ES⋅ΔLLS=EC⋅ΔLLC
- Cancel ΔL from both sides:
ES⋅1LS=EC⋅1LC
5. Rearranging for Length Ratio:
- Rearranging gives:
ESEC=LSLC
6. Substituting the Values:
- Substitute the values of Young's modulus:
2.0×10111.1×1011=LSLC
- Simplifying gives:
LSLC=2.01.1=2011
7. Final Result:
- Therefore, the ratio of the lengths of the two wires is:
LSLC=2011
- Young's modulus of steel, ES=2.0×1011N/m2
- Young's modulus of copper, EC=1.1×1011N/m2
- Let the lengths of the steel and copper wires be LS and LC respectively.
- The elongations in both wires are equal, ΔLS=ΔLC.
- The stress in each wire is the same because they are under the same tension and have equal cross-sectional areas.
- Stress is defined as Stress=TA, where T is the tension and A is the cross-sectional area.
- Since the areas are equal and the tension is the same, we have:
StressS=StressC
- Young's modulus is defined as:
E=StressStrain=T/AΔL/L
- Rearranging gives us:
Stress=E⋅Strain
- For steel:
StressS=ES⋅ΔLSLS
- For copper:
StressC=EC⋅ΔLCLC
- Since ΔLS=ΔLC and StressS=StressC:
ES⋅ΔLLS=EC⋅ΔLLC
- Cancel ΔL from both sides:
ES⋅1LS=EC⋅1LC
- Rearranging gives:
ESEC=LSLC
- Substitute the values of Young's modulus:
2.0×10111.1×1011=LSLC
- Simplifying gives:
LSLC=2.01.1=2011
- Therefore, the ratio of the lengths of the two wires is:
LSLC=2011
Topper's Solved these Questions
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises
32 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Question For short Anser
18 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMA ENGLISHCHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Objective-2
7 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
- SOUND WAVESBOOK - HC VERMA ENGLISHCHAPTER - SOUND WAVESEXERCISE - All Questions
133 Videos
Similar Questions
A steel wire and a copper wire of equal length and equal cross sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of a. The stresses developed in the two wire and b. the strains developed. Y of steel =2×1011Nm−2. Y of copper =1.3×1011Nm−2.
View SolutionOne end of a wire 2m long and 0.2 cm^2 in cross section is fixed in a ceiling and a load of 4.8 kg is attached to the free end. Find the extension of the wire. Young modulus of steel =2.0×1011Nm−2. Take g=10ms−2.
View SolutionIn figure the upper wire is made of steel and the lower of copper. The wires have equal cross section. Find the ratio of the longitudinal strains developed in the two wires.

View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of
copper/steel
(a) the stresses developed . In the two wires,
(b) the strains developed. (Y of steel =2×1011N/m2)
View SolutionA Copper wire of length 2.2m and a steel wire of length 1.6m, both of diameter 3.0mm are connected end to end. When stretched by a load, the net elongation is found to be 0.70 mm. Obtain the load applied . Young's modulus of copper is 1.1×1011Nm−2 and Young's modulus of steel is 2.0×1011Nm−2.
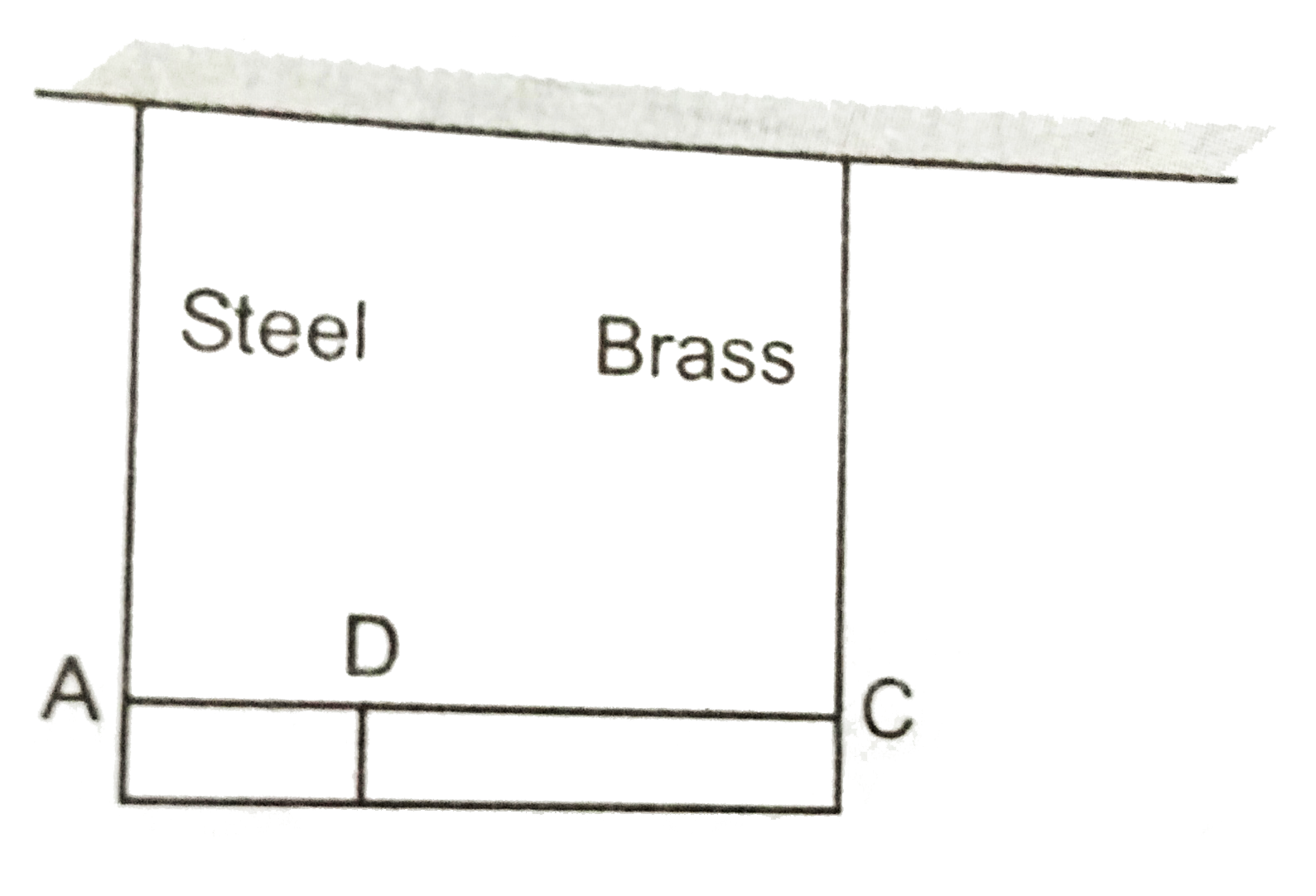
View SolutionA light rod AC of length 2.00 m is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. The position of point D from end A along the rod at which a weight may be hung to produce equal stress in both the wires is [Young's modulus of steel is 2×1011Nm2 and for brass is 1×1011Nm−2]
View SolutionCalculate the Poisson's ratio for steel. Given that Young's modulus is 2×1011Nm−2 and rigidity modulus is 8×1010Nm−2.
View SolutionTwo wires of diameter 0.25 cm, one made of steel and the other made of brass are loaded as shown in fig. The unloaded length of steel wire is 1.5m and that of brass wire is 1.0m. Compute the elongations of the steel and the brass wires.
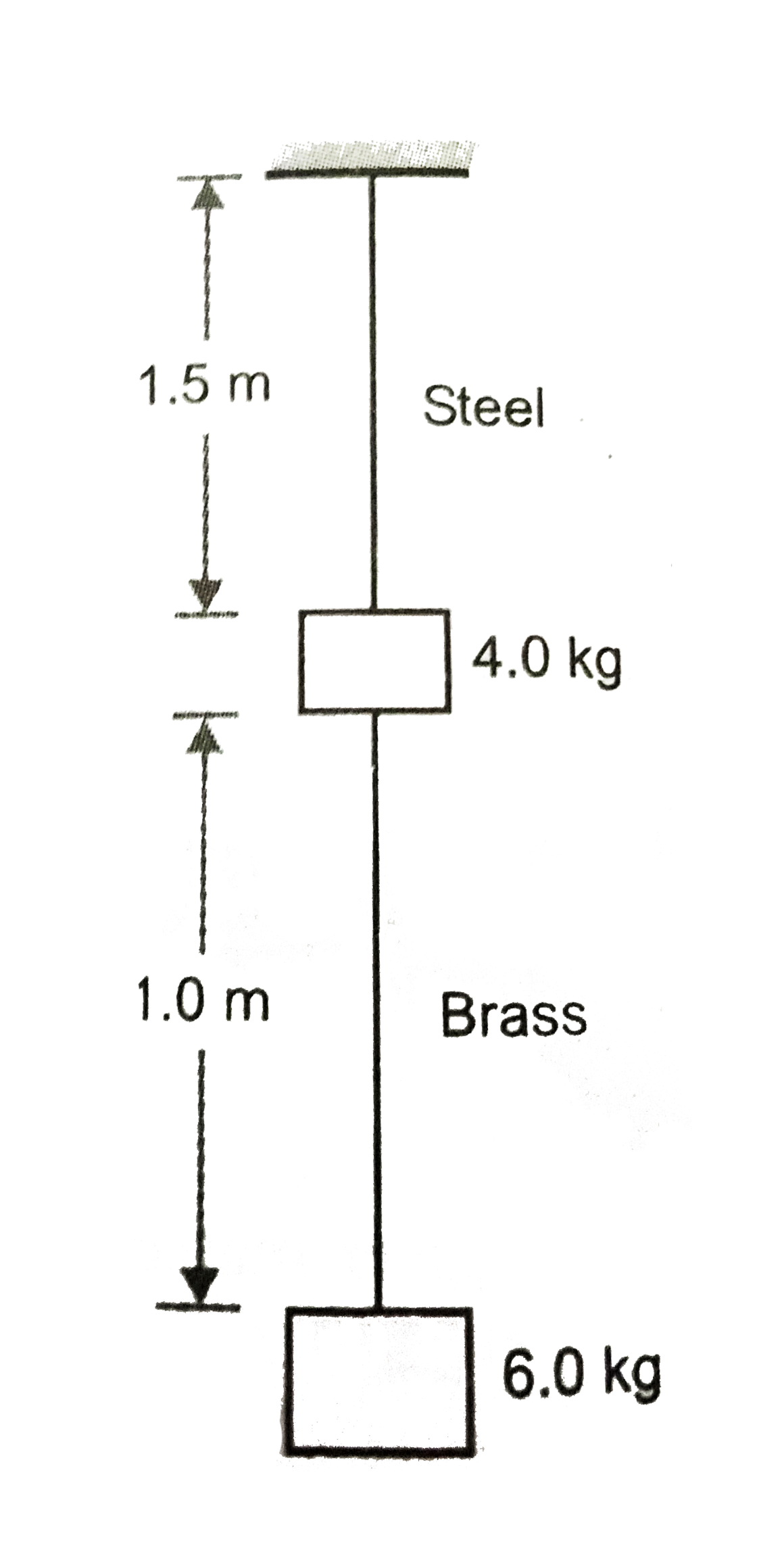
View SolutionTwo wires of diameter 0.25cm, one made of steel and other made of brass, are loaded as shown in the figure. The unloaded length of the steel wire is 1.5m and that of brass is 1.0m. Young's modulus of steel is 2.0×1011Pa and that of brass is 1.0×1011 Pa. Compute the ratio of elongations of steel and brass wires. △lsteel△lbrass=?

View SolutionA block of weight 10 N is fastened to one end of a wire of cross sectional area 3mm2 and is rotated in a vertical circle of radius 20 cm. The speed of the block at the bottom of the circle is 2ms−1. Find the elongation of the wire when the block is at the bottom of the circle. Young modulus of the material of the wire =2×1011Nm−2.
View Solution
